Article contents
Residues of Eisenstein series and the automorphic cohomology of reductive groups
Published online by Cambridge University Press: 07 May 2013
Abstract
Let 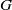 $G$ be a connected, reductive algebraic group over a number field
$G$ be a connected, reductive algebraic group over a number field 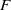 $F$ and let
$F$ and let 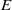 $E$ be an algebraic representation of
$E$ be an algebraic representation of 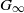 ${G}_{\infty } $. In this paper we describe the Eisenstein cohomology
${G}_{\infty } $. In this paper we describe the Eisenstein cohomology  ${ H}_{\mathrm{Eis} }^{q} (G, E)$ of
${ H}_{\mathrm{Eis} }^{q} (G, E)$ of 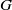 $G$ below a certain degree
$G$ below a certain degree 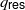 ${q}_{ \mathsf{res} } $ in terms of Franke’s filtration of the space of automorphic forms. This entails a description of the map
${q}_{ \mathsf{res} } $ in terms of Franke’s filtration of the space of automorphic forms. This entails a description of the map  ${H}^{q} ({\mathfrak{m}}_{G} , K, \Pi \otimes E)\rightarrow { H}_{\mathrm{Eis} }^{q} (G, E)$,
${H}^{q} ({\mathfrak{m}}_{G} , K, \Pi \otimes E)\rightarrow { H}_{\mathrm{Eis} }^{q} (G, E)$, 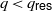 $q\lt {q}_{ \mathsf{res} } $, for all automorphic representations
$q\lt {q}_{ \mathsf{res} } $, for all automorphic representations 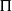 $\Pi $ of
$\Pi $ of  $G( \mathbb{A} )$ appearing in the residual spectrum. Moreover, we show that below an easily computable degree
$G( \mathbb{A} )$ appearing in the residual spectrum. Moreover, we show that below an easily computable degree 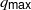 ${q}_{ \mathsf{max} } $, the space of Eisenstein cohomology
${q}_{ \mathsf{max} } $, the space of Eisenstein cohomology 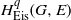 ${ H}_{\mathrm{Eis} }^{q} (G, E)$ is isomorphic to the cohomology of the space of square-integrable, residual automorphic forms. We discuss some more consequences of our result and apply it, in order to derive a result on the residual Eisenstein cohomology of inner forms of
${ H}_{\mathrm{Eis} }^{q} (G, E)$ is isomorphic to the cohomology of the space of square-integrable, residual automorphic forms. We discuss some more consequences of our result and apply it, in order to derive a result on the residual Eisenstein cohomology of inner forms of 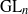 ${\mathrm{GL} }_{n} $ and the split classical groups of type
${\mathrm{GL} }_{n} $ and the split classical groups of type 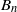 ${B}_{n} $,
${B}_{n} $,  ${C}_{n} $,
${C}_{n} $, 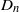 ${D}_{n} $.
${D}_{n} $.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s) 2013
References
- 8
- Cited by


