Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-24T13:14:32.517Z Has data issue: false hasContentIssue false
  • English
  • Français

Intersection theory of nef b-divisor classes

Part of: Cycles and subschemes Birational geometry

Published online by Cambridge University Press:  05 September 2022

Nguyen-Bac Dang  and
Charles Favre
Nguyen-Bac Dang
Affiliation:
Institut de Mathématiques d'Orsay, Université Paris-Saclay, 307 Rue Michel Magat, 91400 Orsay, France [email protected]
Charles Favre
Affiliation:
Centre de Mathématiques Laurent Schwartz, Institut polytechnique de Paris, 91128 Palaiseau, France [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove that any nef 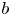$b$-divisor class on a projective variety defined over an algebraically closed field of characteristic zero is a decreasing limit of nef Cartier classes. Building on this technical result, we construct an intersection theory of nef 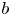$b$-divisors, and prove several variants of the Hodge index theorem inspired by the work of Dinh and Sibony. We show that any big and basepoint-free curve class is a power of a nef 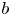$b$-divisor, and relate this statement to the Zariski decomposition of curves classes introduced by Lehmann and Xiao. Our construction allows us to relate various Banach spaces contained in the space of 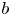$b$-divisors which were defined in our previous work.

Keywords

b-divisorsbirational geometry

MSC classification

Primary: 14C20: Divisors, linear systems, invertible sheaves
Secondary: 14E05: Rational and birational maps
Type
Research Article
Information
Compositio Mathematica , Volume 158 , Issue 7 , July 2022 , pp. 1563 - 1594
Check for updates
Copyright
© 2022 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alexandrov, A. D., Selected works. Part I, Classics of Soviet Mathematics, vol. 4 (Gordon and Breach, Amsterdam, 1996).Google Scholar
Ambro, F., The moduli $b$-divisor of an lc-trivial fibration, Compos. Math. 141 (2005), 385–403.CrossRefGoogle Scholar
Benali, A. and Zeriahi, A., The Hölder continuous subsolution theorem for complex Hessian equations, J. Éc. polytech. Math. 7 (2020), 981–1007.CrossRefGoogle Scholar
Benali, A. and Zeriahi, A., Erratum to: “The Hölder continuous subsolution theorem for complex Hessian equations”, J. Éc. polytech. Math. 8 (2021), 779–789.CrossRefGoogle Scholar
Berman, R. J., Boucksom, S., Guedj, V. and Zeriahi, A., A variational approach to complex Monge-Ampère equations, Publ. Math. Inst. Hautes Études Sci. 117 (2013), 179–245.CrossRefGoogle Scholar
Blanc, J. and Cantat, S., Dynamical degrees of birational transformations of projective surfaces, J. Amer. Math. Soc. 29 (2016), 415–471.CrossRefGoogle Scholar
Botero, A. M. and Burgos Gil, J. I., Toroidal $b$-divisors and Monge-Ampère measures, Math. Z. 300 (2022), 579–637.CrossRefGoogle Scholar
Boucksom, S., Cônes positifs des variétés complexes compactes, PhD thesis, Université Joseph-Fourier-Grenoble I (2002).Google Scholar
Boucksom, S., Divisorial Zariski decompositions on compact complex manifolds, Ann. Sci. Éc. Norm. Supér. (4) 37 (2004), 45–76.CrossRefGoogle Scholar
Boucksom, S., de Fernex, T. and Favre, C., The volume of an isolated singularity, Duke Math. J. 161 (2012), 1455–1520.CrossRefGoogle Scholar
Boucksom, S., Demailly, J.-P., Păun, M. and Peternell, T., The pseudo-effective cone of a compact Kähler manifold and varieties of negative Kodaira dimension, J. Algebraic Geom. 22 (2013), 201–248.CrossRefGoogle Scholar
Boucksom, S., Favre, C. and Jonsson, M., Degree growth of meromorphic surface maps, Duke Math. J. 141 (2008), 519–538.CrossRefGoogle Scholar
Boucksom, S., Favre, C. and Jonsson, M., Valuations and plurisubharmonic singularities, Publ. Res. Inst. Math. Sci. 44 (2008), 449–494.CrossRefGoogle Scholar
Boucksom, S., Favre, C. and Jonsson, M., Differentiability of volumes of divisors and a problem of Teissier, J. Algebraic Geom. 18 (2009), 279–308.CrossRefGoogle Scholar
Boucksom, S., Favre, C. and Jonsson, M., A refinement of Izumi's theorem, in Valuation theory in interaction, EMS Series Congress Reports (European Mathematical Society, Zürich, 2014), 55–81.CrossRefGoogle Scholar
Boucksom, S., Favre, C. and Jonsson, M., Solution to a non-Archimedean Monge-Ampère equation, J. Amer. Math. Soc. 28 (2015), 617–667.CrossRefGoogle Scholar
Boucksom, S., Favre, C. and Jonsson, M., Singular semipositive metrics in non-Archimedean geometry, J. Algebraic Geom. 25 (2016), 77–139.CrossRefGoogle Scholar
Boucksom, S. and Jonsson, M., Singular semipositive metrics on line bundles on varieties over trivially valued fields, Preprint (2018), arXiv:1801.08229.Google Scholar
Boucksom, S. and Jonsson, M., Global pluripotential theory over a trivially valued field, Preprint (2021), arXiv:1801.08229.Google Scholar
Cantat, S., Sur les groupes de transformations birationnelles des surfaces, Ann. of Math. (2) 174 (2011), 299–340.CrossRefGoogle Scholar
Chambert-Loir, A., Mesures et équidistribution sur les espaces de Berkovich, J. Reine Angew. Math. 595 (2006), 215–235.Google Scholar
Chern, S. S., Levine, H. I. and Nirenberg, L., Intrinsic norms on a complex manifold, Global Analysis (Papers in Honor of K. Kodaira) (Univ. Tokyo Press, Tokyo, 1969), 119–139.Google Scholar
Chiose, I., The Kähler rank of compact complex manifolds, J. Geom. Anal. 26 (2016), 603–615.CrossRefGoogle Scholar
Corti, A., 3-fold flips after Shokurov, in Flips for 3-folds and 4-folds, Oxford Lecture Series in Mathematics and its Applications, vol. 35 (Oxford University Press, Oxford, 2007), 18–48.CrossRefGoogle Scholar
Dang, N.-B., Degrees of iterates of rational maps on normal projective varieties, Proc. Lond. Math. Soc. (3) 121 (2020), 1268–1310.CrossRefGoogle Scholar
Dang, N.-B. and Favre, C., Spectral interpretations of dynamical degrees and applications, Ann. of Math. (2) 194 (2021), 299–359.CrossRefGoogle Scholar
Darvas, T., Di Nezza, E. and Lu, C. H., Log-concavity of volume and complex Monge–Ampère equations with prescribed singularity, Math. Ann. 379 (2021), 95–132.CrossRefGoogle Scholar
Debarre, O., Ein, L., Lazarsfeld, R. and Voisin, C., Pseudoeffective and nef classes on abelian varieties, Compos. Math. 147 (2011), 1793–1818.CrossRefGoogle Scholar
Demailly, J.-P., Complex analytic and differential geometry (Citeseer, 1997).Google Scholar
Di Nezza, E., Floris, E. and Trapani, S., Divisorial Zariski decomposition and some properties of full mass currents, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 17 (2017), 1383–1396.Google Scholar
Dinew, S. and Kołodziej, S., A priori estimates for complex Hessian equations, Anal. PDE 7 (2014), 227–244.CrossRefGoogle Scholar
Dinh, T.-C. and Sibony, N., Groupes commutatifs d'automorphismes d'une variété kählérienne compacte, Duke Math. J. 123 (2004), 311–328.CrossRefGoogle Scholar
Dinh, T.-C. and Sibony, N., Regularization of currents and entropy, Ann. Sci. Éc. Norm. Supér. (4) 37 (2004), 959–971.CrossRefGoogle Scholar
Fujino, O., Fundamental theorems for the log minimal model program, Publ. Res. Inst. Math. Sci. 47 (2011), 727–789.CrossRefGoogle Scholar
Fujino, O., Basepoint-free theorems: saturation, b-divisors, and canonical bundle formula, Algebra Number Theory 6 (2012), 797–823.CrossRefGoogle Scholar
Fulger, M. and Lehmann, B., Positive cones of dual cycle classes, Algebr. Geom. 4 (2017), 1–28.CrossRefGoogle Scholar
Fulger, M. and Lehmann, B., Zariski decompositions of numerical cycle classes, J. Algebraic Geom. 26 (2017), 43–106.CrossRefGoogle Scholar
Fulton, W., Intersection theory, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics, vol. 2, second edition (Springer, Berlin, 1998).CrossRefGoogle Scholar
Gignac, W. and Ruggiero, M., Growth of attraction rates for iterates of a superattracting germ in dimension two, Indiana Univ. Math. J. 63 (2014), 1195–1234.Google Scholar
Gubler, W., Local heights of subvarieties over non-Archimedean fields, J. Reine Angew. Math. 498 (1998), 61–113.CrossRefGoogle Scholar
Gubler, W., Jell, P., Künnemann, K. and Martin, F., Continuity of plurisubharmonic envelopes in non-Archimedean geometry and test ideals, Ann. Inst. Fourier (Grenoble) 69 (2019), 2331–2376. With an appendix by José Ignacio Burgos Gil and Martín Sombra.CrossRefGoogle Scholar
Gubler, W. and Martin, F., On Zhang's semipositive metrics, Doc. Math. 24 (2019), 331–372.Google Scholar
Hacon, C. D. and Liu, J., Existence of flips for generalized lc pairs, Preprint (2021), arxiv:2105.13590.Google Scholar
Küronya, A. and Maclean, C., Zariski decomposition of $b$-divisors, Math. Z. 273 (2013), 427–436.CrossRefGoogle Scholar
Kaveh, K. and Khovanskii, A. G., Note on the Grothendieck group of subspaces of rational functions and Shokurov's Cartier $b$-divisors, Canad. Math. Bull. 57 (2014), 562–572.CrossRefGoogle Scholar
Lazarsfeld, R., Positivity in algebraic geometry. II, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics, vol. 49 (Springer, Berlin, 2004). Positivity for vector bundles, and multiplier ideals.CrossRefGoogle Scholar
Lazarsfeld, R. and Mustaţă, M., Convex bodies associated to linear series, Ann. Sci. Éc. Norm. Supér. (4) 42 (2009), 783–835.CrossRefGoogle Scholar
Lehmann, B. and Xiao, J., Convexity and Zariski decomposition structure, Geom. Funct. Anal. 26 (2016), 1135–1189.CrossRefGoogle Scholar
Lehmann, B. and Xiao, J., Positivity functions for curves on algebraic varieties, Algebra Number Theory 13 (2019), 1243–1279.CrossRefGoogle Scholar
Lehmann, B., The movable cone via intersections, Preprint (2011), arXiv:1111.3928.Google Scholar
Li, C., $K$-stability and Fujita approximation, Preprint (2021), arXiv:2102.09457.Google Scholar
Lu, C. H. and Nguyen, V.-D., Degenerate complex Hessian equations on compact Kähler manifolds, Indiana Univ. Math. J. 64 (2015), 1721–1745.CrossRefGoogle Scholar
Nakayama, N., Zariski-decomposition and abundance, MSJ Memoirs, vol. 14 (Mathematical Society of Japan, Tokyo, 2004).Google Scholar
Popovici, D., Sufficient bigness criterion for differences of two nef classes, Math. Ann. 364 (2016), 649–655.CrossRefGoogle Scholar
Popovici, D., Volume and self-intersection of differences of two nef classes, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 17 (2017), 1255–1299.Google Scholar
Rudin, W., Functional analysis, International Series in Pure and Applied Mathematics, second edition (McGraw-Hill, New York, 1991).Google Scholar
Schneider, R., Convex bodies: the Brunn-Minkowski theory, Encyclopedia of Mathematics and its Applications, vol. 151, expanded edition (Cambridge University Press, Cambridge, 2014).Google Scholar
Shokurov, V. V., 3-fold log models, J. Math. Sci. (N.Y.) 81 (1996), 2667–2699.CrossRefGoogle Scholar
Shokurov, V. V., Prelimiting flips, Tr. Mat. Inst. Steklova 240 (2003), 82–219.Google Scholar
Tosatti, V., The Calabi-Yau theorem and Kähler currents, Adv. Theor. Math. Phys. 20 (2016), 381–404.CrossRefGoogle Scholar
Tosatti, V., Orthogonality of divisorial Zariski decompositions for classes with volume zero, Tohoku Math. J. (2) 71 (2019), 1–8.CrossRefGoogle Scholar
Vaquié, M., Valuations, in Resolution of singularities (Obergurgl, 1997), Progress in Mathematics, vol. 181 (Birkhäuser, Basel, 2000), 539–590.CrossRefGoogle Scholar
Witt Nyström, D., Duality between the pseudoeffective and the movable cone on a projective manifold, J. Amer. Math. Soc. 32 (2019), 675–689. With an appendix by Sébastien Boucksom.CrossRefGoogle Scholar
Xiao, J., Weak transcendental holomorphic Morse inequalities on compact Kähler manifolds, Ann. Inst. Fourier (Grenoble) 65 (2015), 1367–1379.CrossRefGoogle Scholar
Xiao, J., Characterizing volume via cone duality, Math. Ann. 369 (2017), 1527–1555.CrossRefGoogle Scholar
Xie, J., Periodic points of birational transformations on projective surfaces, Duke Math. J. 164 (2015), 903–932.Google Scholar
Zhang, S., Small points and adelic metrics, J. Algebraic Geom. 4 (1995), 281–300.Google Scholar
Zhang, Y., On the volume of isolated singularities, Compos. Math. 150 (2014), 1413–1424.CrossRefGoogle Scholar