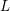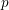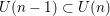Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Zhang, Wei
2014.
Fourier transform and the global Gan--Gross--Prasad conjecture for unitary groups.
Annals of Mathematics,
p.
971.
Heiermann, Volker
2016.
A note on standard modules and Vogan L-packets.
Manuscripta Mathematica,
Vol. 150,
Issue. 3-4,
p.
571.
Gan, Wee Teck
and
Ichino, Atsushi
2016.
The Gross–Prasad conjecture and local theta correspondence.
Inventiones mathematicae,
Vol. 206,
Issue. 3,
p.
705.
Atobe, Hiraku
2016.
On the Uniqueness of Generic Representations in an $L$-Packet.
International Mathematics Research Notices,
p.
rnw220.
Haan, Jaeho
2016.
The local Gan–Gross–Prasad conjecture for U(3)×U(2): The non-generic case.
Journal of Number Theory,
Vol. 165,
Issue. ,
p.
324.
Atobe, Hiraku
and
Gan, Wee Teck
2017.
Local theta correspondence of tempered representations and Langlands parameters.
Inventiones mathematicae,
Vol. 210,
Issue. 2,
p.
341.
Haan, Jaeho
2017.
On the Fourier–Jacobi model for some
endoscopic Arthur packet of U(3) × U(3) : the nongeneric case.
Pacific Journal of Mathematics,
Vol. 286,
Issue. 1,
p.
69.
Atobe, Hiraku
2018.
The local theta correspondence and the local Gan–Gross–Prasad conjecture for the symplectic-metaplectic case.
Mathematische Annalen,
Vol. 371,
Issue. 1-2,
p.
225.
Liu, Dongwen
and
Wang, Zhicheng
2020.
Descents of unipotent representations of finite unitary groups.
Transactions of the American Mathematical Society,
Vol. 373,
Issue. 6,
p.
4223.
Liu, Dongwen
and
Wang, Zhicheng
2021.
On the Gan–Gross–Prasad problem for finite unitary groups.
Mathematische Zeitschrift,
Vol. 297,
Issue. 3-4,
p.
997.
Liu, Dongwen
and
Wang, Zhicheng
2021.
Descents of unipotent cuspidal representations of finite classical groups.
manuscripta mathematica,
Vol. 165,
Issue. 1-2,
p.
159.
Wang, Zhicheng
2021.
On the Gan-Gross-Prasad problem for finite classical groups.
Advances in Mathematics,
Vol. 393,
Issue. ,
p.
108095.
Beuzart-Plessis, Raphaël
2021.
COMPARISON OF LOCAL RELATIVE CHARACTERS AND THE ICHINO–IKEDA CONJECTURE FOR UNITARY GROUPS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 20,
Issue. 6,
p.
1803.
Pollack, Aaron
Wan, Chen
and
Zydor, Michał
2021.
On the residue method for period integrals.
Duke Mathematical Journal,
Vol. 170,
Issue. 7,
Haan, Jaeho
2022.
The local Gan-Gross-Prasad conjecture for U(n + 1)×U(n): A non-generic case.
Journal of Number Theory,
Vol. 233,
Issue. ,
p.
1.
Chan, Kei Yuen
2022.
Restriction for general linear groups: The local non-tempered Gan–Gross–Prasad conjecture (non-Archimedean case).
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2022,
Issue. 783,
p.
49.
Xue, Hang
2023.
Bessel models for real unitary groups: The tempered case.
Duke Mathematical Journal,
Vol. 172,
Issue. 5,
Atobe, Hiraku
Oi, Masao
and
Yasuda, Seidai
2024.
Local newforms for generic representations of unramified odd unitary groups and the Fundamental Lemma.
Duke Mathematical Journal,
Vol. 173,
Issue. 12,
Wang, Zhicheng
2024.
Lusztig correspondence and the finite Gan-Gross-Prasad problem.
Transactions of the American Mathematical Society,
Ørsted, Bent
and
Speh, Birgit
2025.
Symmetry in Geometry and Analysis, Volume 2.
Vol. 358,
Issue. ,
p.
457.