Article contents
Newton non-degenerate  $\mu$-constant deformations admit simultaneous embedded resolutions
$\mu$-constant deformations admit simultaneous embedded resolutions
Published online by Cambridge University Press: 11 August 2022
Abstract
Let 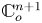 $ {\mathbb {C}}^{n+1}_o$ denote the germ of
$ {\mathbb {C}}^{n+1}_o$ denote the germ of 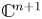 $ {\mathbb {C}}^{n+1}$ at the origin. Let
$ {\mathbb {C}}^{n+1}$ at the origin. Let 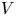 $V$ be a hypersurface germ in
$V$ be a hypersurface germ in 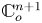 $ {\mathbb {C}}^{n+1}_o$ and
$ {\mathbb {C}}^{n+1}_o$ and 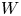 $W$ a deformation of
$W$ a deformation of 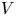 $V$ over
$V$ over  $ {\mathbb {C}}_{o}^{m}$. Under the hypothesis that
$ {\mathbb {C}}_{o}^{m}$. Under the hypothesis that 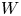 $W$ is a Newton non-degenerate deformation, in this article we prove that
$W$ is a Newton non-degenerate deformation, in this article we prove that 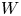 $W$ is a
$W$ is a  $\mu$-constant deformation if and only if
$\mu$-constant deformation if and only if 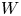 $W$ admits a simultaneous embedded resolution. This result gives a lot of information about
$W$ admits a simultaneous embedded resolution. This result gives a lot of information about 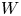 $W$, for example, the topological triviality of the family
$W$, for example, the topological triviality of the family 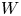 $W$ and the fact that the natural morphism
$W$ and the fact that the natural morphism 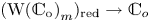 $(\operatorname {W( {\mathbb {C}}_{o})}_{m})_{{\rm red}}\rightarrow {\mathbb {C}}_{o}$ is flat, where
$(\operatorname {W( {\mathbb {C}}_{o})}_{m})_{{\rm red}}\rightarrow {\mathbb {C}}_{o}$ is flat, where 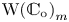 $\operatorname {W( {\mathbb {C}}_{o})}_{m}$ is the relative space of
$\operatorname {W( {\mathbb {C}}_{o})}_{m}$ is the relative space of  $m$-jets. On the way to the proof of our main result, we give a complete answer to a question of Arnold on the monotonicity of Newton numbers in the case of convenient Newton polyhedra.
$m$-jets. On the way to the proof of our main result, we give a complete answer to a question of Arnold on the monotonicity of Newton numbers in the case of convenient Newton polyhedra.
Keywords
- Type
- Research Article
- Information
- Copyright
- © 2022 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence
Footnotes
The first author is partially supported by Projects ANID FONDECYT 1170743 and 1221535. The second author is partially supported by Projet ANR LISA, ANR-17-CE40-0023.
References
- 3
- Cited by



