Article contents
Diophantine properties of nilpotent Lie groups
Published online by Cambridge University Press: 13 January 2015
Abstract
A finitely generated subgroup  ${\rm\Gamma}$ of a real Lie group
${\rm\Gamma}$ of a real Lie group 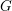 $G$ is said to be Diophantine if there is
$G$ is said to be Diophantine if there is 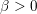 ${\it\beta}>0$ such that non-trivial elements in the word ball
${\it\beta}>0$ such that non-trivial elements in the word ball  $B_{{\rm\Gamma}}(n)$ centered at
$B_{{\rm\Gamma}}(n)$ centered at 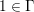 $1\in {\rm\Gamma}$ never approach the identity of
$1\in {\rm\Gamma}$ never approach the identity of  $G$ closer than
$G$ closer than 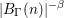 $|B_{{\rm\Gamma}}(n)|^{-{\it\beta}}$. A Lie group
$|B_{{\rm\Gamma}}(n)|^{-{\it\beta}}$. A Lie group  $G$ is said to be Diophantine if for every
$G$ is said to be Diophantine if for every 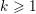 $k\geqslant 1$ a random
$k\geqslant 1$ a random  $k$-tuple in
$k$-tuple in  $G$ generates a Diophantine subgroup. Semi-simple Lie groups are conjectured to be Diophantine but very little is proven in this direction. We give a characterization of Diophantine nilpotent Lie groups in terms of the ideal of laws of their Lie algebra. In particular we show that nilpotent Lie groups of class at most
$G$ generates a Diophantine subgroup. Semi-simple Lie groups are conjectured to be Diophantine but very little is proven in this direction. We give a characterization of Diophantine nilpotent Lie groups in terms of the ideal of laws of their Lie algebra. In particular we show that nilpotent Lie groups of class at most 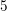 $5$, or derived length at most
$5$, or derived length at most 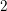 $2$, as well as rational nilpotent Lie groups are Diophantine. We also find that there are non-Diophantine nilpotent and solvable (non-nilpotent) Lie groups.
$2$, as well as rational nilpotent Lie groups are Diophantine. We also find that there are non-Diophantine nilpotent and solvable (non-nilpotent) Lie groups.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Authors 2015
References
- 7
- Cited by


