Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-25T04:00:54.922Z Has data issue: false hasContentIssue false
  • English
  • Français

Sur la Convergence Ponctuelle de Quelques Suites D'Operateurs

Published online by Cambridge University Press:  20 November 2018

I. Assani
I. Assani*
Affiliation:
Department of Mathematics University of Toronto Toronto, ONT. M5S 1A1
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let (αn.k) be a sequence of positive numbers. We define a regular sequence (resp. a weakly regular sequence) and then show the existence of a unitary operator (resp. a contraction T) L2[0, 1] → L2[0, 1] and a function f ∊ L2[0, 1] such that the pointwise convergence of the sequence of functions is not satisfied almost surely. As a first corollary the pointwise convergence of the Abel means of a contraction from L2 into L2 does not hold necessarily almost surely. As a second corollary there exists a contraction T for which the means (and powers) of Brunei's operator A do not converge pointwise a.s. We also show that, for P > 1 fixed, there exists a sequence of positive numbers αn.k for which we have the pointwise convergence in LP of the sequence of polynomials 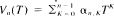where T is a contraction of L1 and Lα. The dominated theorem does not, however, always hold for such LP-contractions.

Keywords

47A35
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 30 , Issue 2 , 01 June 1987 , pp. 134 - 141
Copyright
Copyright © Canadian Mathematical Society 1987

References

1. Akcoglu, M.A., Pointwise ergodic theorems in Lp spaces, Proc. Conf. Ergodic theory (Oberwolfach 1978). Lecture Notes in Math. n° 729. pp. 13–15, Springer-Verlag, Berlin Heidelberg, New York, 1979.Google Scholar
2. Assani, I. and Mesiar, R., Sur la convergence ponctuelle de Tn/nα A paraître dans les annales de Clermont-Ferrand n° 3.Google Scholar
3. Brunei, A., Théorème ergodique ponctuel pour un semi-groupe commutatif finiment engendré, la contractions de L| , Ann. Inst. Henri Poincaré, Sect. B, vol. 9, n° 4 (1973), pp. 327–343.Google Scholar
4. Burkholder, D.L., Semi-Gaussian subspaces, Trans. A.M.S. 104 (1962), pp. 123–131.Google Scholar
5. Dunford, N. and Schwartz, J.T., Linear Operators, vol. 1, Interscience Publishers, New York, 1958.Google Scholar
6. Feder, M., On power-bounded operators and the pointwise ergodic property, Proc. A.M.S, . vol. 83, n° 2, pp. 349–353, Octobre 1981.Google Scholar
7. Irmisch, R., Punktweise ergodensàtze für (C,α)-Verfahren 0 < α < 1, (Thèse), Vom Fachbereich Mathematik der Technischen Hochschule Darmstadt, 1980.Google Scholar
8. Marcinkiewicz, J., Sur l'interpolation d'opérations, C.R.A.S. Paris A, 208 (1939), p. 1271.Google Scholar
9. Menchoff, D., Sur les séries de fonctions orthogonales, Fund. Math. 4 (1923), pp. 82–105.Google Scholar