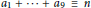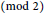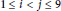Let ${{a}_{1}},\,.\,.\,.\,,\,{{a}_{9}}$ be non-zero integers and $n$ any integer. Suppose that ${{a}_{1}}\,+\,.\,.\,.\,+\,{{a}_{9}}\,\equiv \,n$$\left( \bmod \,2 \right)$ and $\left( {{a}_{i}},\,{{a}_{i}} \right)\,=\,1$ for $1\,\le \,i\,<\,j\le \,9$. In this paper we prove that

• (i) if ${{a}_{j}}$ are not all of the same sign, then the cubic equation 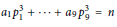${{a}_{1}}p_{1}^{3}\,+\,.\,.\,.\,+\,{{a}_{9}}p_{9}^{3}\,=\,n$ has prime solutions satisfying 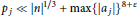${{p}_{j}}\,\ll \,{{\left| n \right|}^{{1}/{3}\;}}\,+\,\max {{\left\{ \left| {{a}_{j}} \right| \right\}}^{8+\varepsilon }}$;

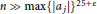• (ii) if all ${{a}_{j}}$ are positive and $n\,\gg \,\max {{\left\{ \left| {{a}_{j}} \right| \right\}}^{25+\varepsilon }}$ , then ${{a}_{1}}p_{1}^{3}\,+\,.\,.\,.\,+\,{{a}_{j}}p_{9}^{3}\,=\,n$ is soluble in primes 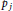$Pj$.

These results improve our previous results with the bounds 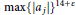$\max {{\left\{ \left| {{a}_{j}} \right| \right\}}^{14+\varepsilon }}$ and 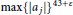$\max \,{{\left\{ \left| {{a}_{j}} \right| \right\}}^{43+\varepsilon }}$ in place of $\max {{\left\{ \left| {{a}_{j}} \right| \right\}}^{8+\varepsilon }}$ and 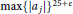$\max {{\left\{ \left| {{a}_{j}} \right| \right\}}^{25+\varepsilon }}$ above, respectively.