Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-23T23:23:46.567Z Has data issue: false hasContentIssue false
  • English
  • Français

Rings whose Elements are the Sum of a Tripotent and an Element from the Jacobson Radical

Part of: Rings and algebras arising under various constructions Conditions on elements

Published online by Cambridge University Press:  15 March 2019

M. Tamer Koşan ,
Tülay Yildirim  and
Y. Zhou
M. Tamer Koşan
Affiliation:
Department of Mathematics, Gazi University, Ankara, Turkey Email: [email protected]
Tülay Yildirim
Affiliation:
Department of Mathematics, Gebze Technical University, Gebze/Kocaeli, Turkey Email: [email protected]
Y. Zhou
Affiliation:
Department of Mathematics and Statistics, Memorial University of Newfoundland, St.John’s, NL A1C 5S7, Canada Email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper is about rings $R$ for which every element is a sum of a tripotent and an element from the Jacobson radical 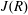$J(R)$. These rings are called semi-tripotent rings. Examples include Boolean rings, strongly nil-clean rings, strongly 2-nil-clean rings, and semi-boolean rings. Here, many characterizations of semi-tripotent rings are obtained. Necessary and sufficient conditions for a Morita context (respectively, for a group ring of an abelian group or a locally finite nilpotent group) to be semi-tripotent are proved.

Keywords

idempotenttripotentJacobson radicalidempotent lifting modulo Jacobson radicalBoolean ringsemi-boolean ring

MSC classification

Primary: 16U99: None of the above, but in this section
Secondary: 16S34: Group rings , Laurent polynomial rings
Type
Article
Information
Canadian Mathematical Bulletin , Volume 62 , Issue 4 , December 2019 , pp. 810 - 821
Copyright
© Canadian Mathematical Society 2019 

References

Burgess, W. D. and Raphael, R., On commutative clean rings and pm rings . In: Rings, Modules and Representations , Contemp. Math., 480, Amer. Math. Soc., Providence, RI, 2009, pp. 35–55.Google Scholar
Chen, H., On strongly J-clean rings . Commun. Algebra 38(2010), 3790–3804.Google Scholar
Chen, H. and Sheibani, M., Strongly 2-nil-clean rings . J. Algebra Appl. 16(2017), 1750178 (12 pages).Google Scholar
Connell, I. G., On the group ring . Canad. J. Math. 15(1963), 650–685.Google Scholar
Diesl, A. J., Nil clean rings . J. Algebra 383(2013), 197–211.Google Scholar
Hirano, Y. and Tominaga, H., Rings in which every element is the sum of two idempotents . Bull. Austral. Math. Soc. 37(1988), 161–164.Google Scholar
Khurana, D., Lam, T. Y., and Nielsen, P. P., Exchange rings, exchange equations, and lifting properties . Internat. J. Algebra Comput. 26(2016), 1177–1198.Google Scholar
Koşan, T., Leroy, A., and Matczuk, J., On UJ-rings . Commun. Algebra 46(2018), 2297–2303.Google Scholar
Koşan, T., Wang, Z., and Zhou, Y., Nil-clean and strongly nil-clean rings . J. Pure Appl. Algebra 220(2016), 633–646.Google Scholar
Lee, T. K. and Zhou, Y., A class of exchange rings . Glasgow Math. J. 50(2008), 509–522.Google Scholar
Levitzki, J., On the structure of algebraic algebras and related rings . Trans. Amer. Math. Soc. 74(1953), 384–409.Google Scholar
Matczuk, J., Conjugate (nil) clean rings and Köthe’s conjecture . J. Algebra Appl. 16(2017), 1750073 (14 pages).Google Scholar
Mazurek, R., Nielsen, P. P., and Ziembowski, M., Commuting idempotents, square-free modules, and the exchange property . J. Algebra 444(2015), 52–80.Google Scholar
Nicholson, W. K., Lifting idempotents and exchange rings . Trans. Amer. Math. Soc. 229(1977), 269–278.Google Scholar
Nicholson, W. K. and Zhou, Y., Clean general rings . J. Algebra 291(2005), 297–311.Google Scholar
Nicholson, W. K. and Zhou, Y., Strong lifting . J. Algebra 285(2005), 795–818.Google Scholar
Sands, A. D., Radicals and Morita contexts . J. Algebra 24(1973), 335–345.Google Scholar
Zhou, Y., On clean group rings . In: Advances in Ring Theory , Trends in Mathematics, Birkhäuser, Verlag Basel/Switzerland, 2010, pp. 335–345.Google Scholar
Zhou, Y., Rings in which elements are sums of nilpotents, idempotents and tripotents . J. Algebra Appl. 17(2018), 1850009 (7 pages).Google Scholar