Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Geramita, A.V
and
Orecchia, F
1982.
Minimally generating ideals defining certain tangent cones.
Journal of Algebra,
Vol. 78,
Issue. 1,
p.
36.
Orecchia, Ferruccio
1983.
Algebraic Geometry — Open Problems.
Vol. 997,
Issue. ,
p.
376.
Maggioni, Renato
and
Ragusa, Alfio
1985.
Nonsingular curves passing through points of P2 in generic position, II.
Journal of Algebra,
Vol. 92,
Issue. 1,
p.
194.
De Paris, Alessandro
1999.
On certain classes of curve singulariries with reduced tangent cone.
Communications in Algebra,
Vol. 27,
Issue. 12,
p.
6159.
Castaldo, G.
and
Ilardi†, G.
2001.
HYPERPLANE AND HYPERSURFACE SECTIONS OF MULTIPLE SUBVARIETIES OF CODIMENSION ONE.
Communications in Algebra,
Vol. 29,
Issue. 7,
p.
2923.
Ilardi, G.
and
Castaldo, G.
2005.
THE STRUCTURE OF THE TANGENT CONE: AN INTERESTING BIJECTION#.
Communications in Algebra,
Vol. 33,
Issue. 4,
p.
1043.
Paris, Alessandro De
and
Orecchia, Ferruccio
2008.
Reduced Tangent Cones and Conductor at Multiplanar Isolated Singularities.
Communications in Algebra,
Vol. 36,
Issue. 8,
p.
2969.
Orecchia, Ferruccio
and
Ramella, Isabella
2014.
Minimal generation of ideals of algebraic varieties at points with multilinear tangent cones.
Ricerche di Matematica,
Vol. 63,
Issue. S1,
p.
249.
Orecchia, Ferruccio
and
Ramella, Isabella
2020.
Ordinary Isolated Singularities of Algebraic Varieties.
Mediterranean Journal of Mathematics,
Vol. 17,
Issue. 3,
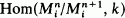 span the vector space
span the vector space  . If the points of the projectivized tangent cone Proj(G(A)) are in generic position then p is an ordinary singularity if and only if G(A) is reduced. We give an example which shows that the preceding equivalence is not true in general.
. If the points of the projectivized tangent cone Proj(G(A)) are in generic position then p is an ordinary singularity if and only if G(A) is reduced. We give an example which shows that the preceding equivalence is not true in general.