No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
We establish a discrete-time criteria guaranteeing the existence of an exponential dichotomy in the continuous-time behavior of an abstract evolution family. We prove that an evolution family  $\mathcal{U}\,=\,{{\{U(t,\,s)\}}_{t\ge s\ge 0}}$ acting on a Banach space
$\mathcal{U}\,=\,{{\{U(t,\,s)\}}_{t\ge s\ge 0}}$ acting on a Banach space 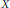 $X$ is uniformly exponentially dichotomic (with respect to its continuous-time behavior) if and only if the corresponding difference equation with the inhomogeneous term from a vector-valued Orlicz sequence space
$X$ is uniformly exponentially dichotomic (with respect to its continuous-time behavior) if and only if the corresponding difference equation with the inhomogeneous term from a vector-valued Orlicz sequence space 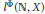 ${{l}^{\Phi }}(\mathbb{N},\,X)$ admits a solution in the same
${{l}^{\Phi }}(\mathbb{N},\,X)$ admits a solution in the same 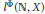 ${{l}^{\Phi }}(\mathbb{N},\,X)$. The technique of proof effectively eliminates the continuity hypothesis on the evolution family (i.e., we do not assume that
${{l}^{\Phi }}(\mathbb{N},\,X)$. The technique of proof effectively eliminates the continuity hypothesis on the evolution family (i.e., we do not assume that 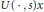 $U(\,\cdot \,,\,s)x$ or
$U(\,\cdot \,,\,s)x$ or 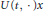 $U(t,\,\cdot \,)x$ is continuous on
$U(t,\,\cdot \,)x$ is continuous on 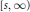 $[s,\,\infty )$, and respectively
$[s,\,\infty )$, and respectively 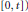 $[0,\,t])$. Thus, some known results given by Coffman and Schaffer, Perron, and Ta Li are extended.
$[0,\,t])$. Thus, some known results given by Coffman and Schaffer, Perron, and Ta Li are extended.