Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-29T01:53:14.987Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Borel-Cantelli Problem

Published online by Cambridge University Press:  20 November 2018

Jonathan Shuster
Jonathan Shuster*
Affiliation:
McGill University, Montreal, Quebec
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let (Ω, 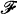, P) be a probability space, and A1, A2… be a sequence of members of 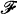. The classical Borel-Cantelli problem is to determine the probability that infinitely many events Ak occur. The classical results may be found in Feller [2, p. 188]; while related work may be found in Spitzer [3, p. 317], and Dawson and Sankoff [1]. The latter works are generalizations of the Borel-Cantelli lemmas, taken in different directions.

In this paper, necessary and sufficient conditions will be given for infinitely many events Ak to occur, with probability 1. A lower bound for the probability that only finitely many Ak occur, is developed. In addition, necessary and sufficient conditions that only finitely many Ak occur, with probability 1, are given.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 13 , Issue 2 , June 1970 , pp. 273 - 275
Copyright
Copyright © Canadian Mathematical Society 1970

References

1. Dawson, D. and Sankoff, D., An inequality for probabilities, Proc. Amer. Math. Soc. (3) 18 (1967), 504-507.Google Scholar
2. Feller, W., An introduction to probability theory and its applications, Wiley, New York, I (second edition), 1957.Google Scholar
3. Spitzer, F., Principles of random walk, Van Nostrand, Princeton, N.J., 1964.Google Scholar