Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-12T21:37:41.907Z Has data issue: false hasContentIssue false
  • English
  • Français

On Extensions of Stably Finite C*-Algebras (II)

Published online by Cambridge University Press:  20 November 2018

Hongliang Yao
Hongliang Yao*
Affiliation:
School of Science, Nanjing University of Science and Technology, Nanjing 210094, P. R. China e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For any 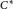${{C}^{*}}$ -algebra $A$ with an approximate unit of projections, there is a smallest ideal $I$ of $A$ such that the quotient $A$ / $I$ is stably finite. In this paper a sufficient and necessary condition for an ideal of a 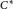${{C}^{*}}$ -algebra with real rank zero to be this smallest ideal is obtained by using $K$ -theory

Keywords

46L0546L80extensionstably finite C*-algebraindex map
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 59 , Issue 2 , 01 June 2016 , pp. 435 - 439
Copyright
Copyright © Canadian Mathematical Society 2016

References

[1] Arveson, W., Notes on extensions of C*-algebras. Duke Math. J. 44(1977), 329–355. http://dx.doi.org/10.1215/S0012-7094-77-04414-3 Google Scholar
[2] Blackadar, B., K-theoryfor operator algebras. Springer-Verlag, New York, 1986.Google Scholar
[3] Lin, H., An introduction to the classification of amenable C* -algebras, World Scientific, New Jersey/London/Singapore/Hong Kong, 2001.Google Scholar
[4] Spielberg, J. S., Embedding C* -algebra extensions into AF-algebras. J. Funct. Anal. 81(1988), 325–344. http://dx.doi.org/10.1016/0022-1236(88)90104-8 Google Scholar
[5] Yao, H., On extensions of stably finite C*-algebras. J. Operator Theory 67(2012), no. 2, 329–334.Google Scholar
[6] Zhang, S., C* -algebras with real rank zero and the internal structure of their corona and multiplier algebras, Parti. Pacific J. Math. 155(1992), no. 1,169-197. http://dx.doi.org/!0.2140/pjm.1992.155.169 Google Scholar