Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-24T00:16:31.173Z Has data issue: false hasContentIssue false
  • English
  • Français

The Hausdorff Moment Problem

Published online by Cambridge University Press:  20 November 2018

David Borwein
David Borwein*
Affiliation:
Department of Mathematics, The University of Western Ontario, London, Ontario, Canada N6A 5B9
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Suppose throughout that

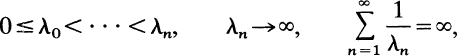
and that {μn}(n≥ 0) is a sequence of real numbers. The (generalized) Hausdorff moment problem is to determine necessary and sufficient conditions for there to be a function x in some specified class satisfying

.

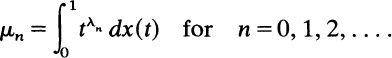Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 21 , Issue 3 , 01 September 1978 , pp. 257 - 265
Copyright
Copyright © Canadian Mathematical Society 1978

References

1. Akhiezer, N. I.. The Classical Moment Problem, Oliver and Boyd, Edinburgh, 1965.Google Scholar
2. Banach, S., Théories des Opérations Linéaires, Warsaw, 1932.Google Scholar
3. Berman, D. L., Application of interpolator polynomial operators to solve the moment problem, Ukrain Math. Ž. 14 (1963), 184-190.Google Scholar
4. Endl, K., On systems of linear inequalities in infinitely many variables and generalized Hausdorff means, Math. Z. 82 (1963), 1-7.Google Scholar
5. Hausdorff, F., Summationsmethoden und Momentfolgen I, Math. Z. 9 (1921), 74-109.Google Scholar
6. Hausdorff, F., Summationsmethoden und Momentfolgen II, Math. Z. 9 (1921), 280-299.Google Scholar
7. Hausdorff, F., Momentprobleme fur ein eindliches Interval, Math. Z. 16 (1923), 220-248.Google Scholar
8. Hildebrandt, T. H., On the moment problem for a finite interval, Bull. A.M.S. 38 (1932), 269-270.Google Scholar
9. Leviatan, D., A generalized moment problem, Israel J. Math. 5 (1967), 97-103.Google Scholar
10. Leviatan, D., Some moment problems in a finite interval, Can. J. Math. 20 (1968), 960-966.Google Scholar
11. Rudin, W., Real and Complex Analysis, Second edition, McGraw-Hill, 1974.Google Scholar
12. Schoenberg, I. J., On finite rowed systems of linear inequalities in infinitely many variables, Trans. A.M.S. 34 (1932) 594-619.Google Scholar
13. Shohat, J. A. and Tamarkin, L. D., The Problem of Moments, A.M.S., 1943.Google Scholar
14. Widder, D. V., The Laplace Transform, Princeton, 1946 Google Scholar