Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Margolis, Stuart W.
1981.
On M-varieties generated by power monoids.
Semigroup Forum,
Vol. 22,
Issue. 1,
p.
339.
Gould, Matthew
and
Iskra, Joseph A.
1984.
Embedding in globals of groups and semilattices.
Semigroup Forum,
Vol. 28,
Issue. 1,
p.
61.
Margolis, Stuart W.
and
Pin, Jean-Eric
1984.
Power monoids and finiteJ-trivial monoids.
Semigroup Forum,
Vol. 29,
Issue. 1,
p.
99.
Tamura, Takayuki
1986.
Isomorphism problem of power semigroups of completely 0-simple semigroups.
Journal of Algebra,
Vol. 98,
Issue. 2,
p.
319.
Tamura, Takayuki
1987.
Semigroups and Their Applications.
p.
191.
Pin, J. E.
1987.
Semigroups and Their Applications.
p.
139.
Blanchard, Paul
1991.
Monoides de comptage et langages rationnels.
Semigroup Forum,
Vol. 43,
Issue. 1,
p.
218.
Almeida, Jorge
1993.
A unified syntactical approach to theorems of Putcha, Margolis, and Straubing on finite power semigroups.
Semigroup Forum,
Vol. 46,
Issue. 1,
p.
90.
Almeida, Jorge
1993.
Locally commutative power semigroups and counting factors of words.
Theoretical Computer Science,
Vol. 108,
Issue. 1,
p.
3.
Schwarz, S.
1995.
Powers of subsets in a finite semigroup.
Semigroup Forum,
Vol. 51,
Issue. 1,
p.
1.
GAN, AIPING
and
ZHAO, XIANZHONG
2014.
GLOBAL DETERMINISM OF CLIFFORD SEMIGROUPS.
Journal of the Australian Mathematical Society,
Vol. 97,
Issue. 1,
p.
63.
Yu, Baomin
Zhao, Xianzhong
and
Gan, Aiping
2018.
Global determinism of idempotent semigroups.
Communications in Algebra,
Vol. 46,
Issue. 1,
p.
241.
Dolinka, Igor
Gusev, Sergey V.
and
Volkov, Mikhail V.
2024.
Semiring and involution identities of powers of inverse semigroups.
Communications in Algebra,
Vol. 52,
Issue. 5,
p.
1922.
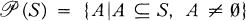 . If
. If 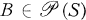 , then let AB = {ab| a ∈ A, b ∈ B}.
, then let AB = {ab| a ∈ A, b ∈ B}. 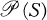 has been studied by many authors, including [2, 3, 5, 6, 7]. If X is a set, then |X| denotes the cardinality of X. For undefined terms in this paper, see [1,4].
has been studied by many authors, including [2, 3, 5, 6, 7]. If X is a set, then |X| denotes the cardinality of X. For undefined terms in this paper, see [1,4].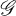 a subgroup of
a subgroup of 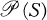 . Then
. Then 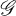 has a normal subgroups
has a normal subgroups 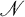 such that
such that 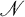 is isomorphic to a subgroup of
is isomorphic to a subgroup of  and
and  is isomorphic to a subgroup of
is isomorphic to a subgroup of 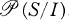 .
.