Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-26T01:14:48.409Z Has data issue: false hasContentIssue false
  • English
  • Français

S-Maximal Subgroups of πl(M3)

Published online by Cambridge University Press:  20 November 2018

C. D. Feustel
C. D. Feustel*
Affiliation:
Institute for Defense Analyses, Princeton, New Jersey; Virginia Polytechnic and State University, Blacksburg, Virginia
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let M be a compact, connected, irreducible 3-manifold. Let S be a closed, connected, 2-manifold other than the 2-sphere or projective plane. Let f be a map of S into M such that

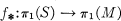
Suppose for every closed, connected surface S1 and every map g:S1 → M such that

(1) 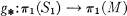is an injection,

(1)

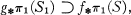
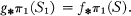Then we shall say that the subgroup 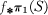is a surface maximal or S-maximal subgroup of π1(M). We may also say that the map f is S-maximal.

Let M be an irreducible 3-manifold which does not admit any embedding of the projective plane. Then we shall say that M is p2-irreducible. Throughout this paper all spaces will be simplicial complexes and all maps will be piecewise linear.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 24 , Issue 3 , June 1972 , pp. 439 - 449
Copyright
Copyright © Canadian Mathematical Society 1972

References

1. Brown, E. M., Unknotting in M2 × I , Trans. Amer. Math. Soc. 123 (1966), 480–505.Google Scholar
2. Brown, E. M. and Crowell, R. H., The augementation subgroup of a link, J. Math. Mech. 15 (1966), 1065–1074.Google Scholar
3. Epstein, D. B. A., Projective planes in 8-manifolds, Proc. London Math. Soc. 11 (1961), 469–484.Google Scholar
4. Feustel, C. D., Some applications of Waldhausen's results on irreducible surfaces, Trans. Amer. Math Soc. 149 (1970), 475–583.Google Scholar
5. Heil, W., On p2-irreducible 3-manifolds, Bull. Amer. Math. Soc. 75 (1969), 772–775.Google Scholar
6. Milnor, J., A unique decomposition theorem for 3-manifolds, Amer. J. Math. 84 (1962), 1–7.Google Scholar
7. Stallings, J., On the loop theorem, Ann. of Math. 72 (1960), 12–19.Google Scholar
8. Waldhausen, F., On irreducible 3-manifolds which are sufficiently large, Ann. of Math. 87 (1968), 56–88.Google Scholar