There is a well-known correspondence (due to Mehta and Seshadri in the unitary case, and extended by Bhosle and Ramanathan to other groups), between the symplectic variety 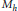${{M}_{h}}$ of representations of the fundamental group of a punctured Riemann surface into a compact connected Lie group $G$, with fixed conjugacy classes $h$ at the punctures, and a complex variety 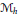${{\mathcal{M}}_{h}}$ of holomorphic bundles on the unpunctured surface with a parabolic structure at the puncture points. For 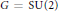$G\,=\,\text{SU}\left( 2 \right)$, we build a symplectic variety $P$ of pairs (representations of the fundamental group into $G$, “weighted frame” at the puncture points), and a corresponding complex variety 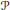$\mathcal{P}$ of moduli of “framed parabolic bundles”, which encompass respectively all of the spaces 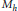${{M}_{h}}$, 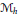${{\mathcal{M}}_{h}}$, in the sense that one can obtain 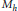${{M}_{h}}$ from $P$ by symplectic reduction, and 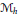${{\mathcal{M}}_{h}}$ from 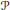$\mathcal{P}$ by a complex quotient. This allows us to explain certain features of the toric geometry of the 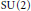$\text{SU(2)}$ moduli spaces discussed by Jeffrey and Weitsman, by giving the actual toric variety associated with their integrable system.