Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T01:06:00.891Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Selmer Group of Twists of Elliptic Curves with Q-Rational Torsion Points

Published online by Cambridge University Press:  20 November 2018

G. Frey
G. Frey*
Affiliation:
Universität des Saarlandes, Saarbrücken, West Germany
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

(1) The symbols p and q stand for prime numbers and throughout the paper we assume that p is fixed and contained in {3, 5, 7}. Let L be an algebraic number field (i.e., L is a finite extension of Q). Then prime divisors of L dividing p (resp. q) are denoted by (resp. 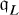). The completion of L with respect to is denoted by . Let S be a finite set of prime numbers, and let M/L be a Galois extension with abelian Galois group of exponent p.

Definition. M/L is said to be little ramified outside S if for primes q ∉ S and all one has

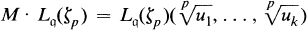
with k ∊ N and . Here ζp is a pth root of unity, u1, …, uk are elements in and is the normed valuation belonging to . In particular M/L is unramified at all divisors of primes q ∉ S ∪ {p}.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 40 , Issue 3 , 01 June 1988 , pp. 649 - 665
Copyright
Copyright © Canadian Mathematical Society 1988

References

1. Frey, G., A relation between the value of the L-series of the curve y2 = x2 — k2 in s — 1 and its Selmer group, Archiv der Math. 45 (1985), 232–238.Google Scholar
2. Lutz, E., Sur l'équation y2 — x2 — Ax — B dans les corps p-adiques, J. reine angew. Math. 777 (1937), 238–247.Google Scholar
3. Mazur, B., On the arithmetic of special values of L-functions, Invent. Math. 55 (1979), 207–240.Google Scholar
4. Néron, A., Modèles minimaux des variétés abéliennes sur les corps locaux et globaux, Inst. Hautes Études Sci. Publ. Math. 21 (1964).Google Scholar
5. Roquette, P., Analytic theory of elliptic functions over local fields, Hamburger Mathematische Einzelschriften (Neue Folge), Heft 1 (Vandenhoech and Ruprecht, Güttingen, 1970).Google Scholar
6. Serre, J. P., Corps locaux (Hermann, Paris, 1962).Google Scholar