Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Grimmett, G. R.
1980.
Random labelled trees and their branching networks.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 30,
Issue. 2,
p.
229.
Flajolet, Philippe
and
Odlyzko, Andrew
1980.
Exploring binary trees and other simple trees.
p.
207.
Flajolet, Philippe
and
Steyaert, Jean-Marc
1980.
Automata, Languages and Programming.
Vol. 85,
Issue. ,
p.
208.
Meir, A.
and
Moon, J. W.
1981.
The outer-distance of nodes in random trees.
Aequationes Mathematicae,
Vol. 23,
Issue. 1,
p.
204.
Flajolet, Philippe
and
Steyaert, Jean-Marc
1981.
A complexity calculus for classes of recursive search programs over tree structures.
p.
386.
Dershowitz, Nachum
and
Zaks, Shmuel
1981.
CAAP '81.
Vol. 112,
Issue. ,
p.
180.
Meir, A.
and
Moon, J. W.
1981.
Terminal path numbers for certain families of trees.
Journal of the Australian Mathematical Society,
Vol. 31,
Issue. 4,
p.
429.
Capobianco, Michael
1981.
Statistical Distributions in Scientific Work.
p.
397.
Karoński, Michał
1982.
A review of random graphs.
Journal of Graph Theory,
Vol. 6,
Issue. 4,
p.
349.
Flajolet, Philippe
and
Odlyzko, Andrew
1982.
The average height of binary trees and other simple trees.
Journal of Computer and System Sciences,
Vol. 25,
Issue. 2,
p.
171.
Kemp, R.
1982.
Theoretical Computer Science.
Vol. 145,
Issue. ,
p.
153.
Kirschenhofer, Peter
and
Prodinger, Helmut
1982.
On the average hyperoscillations of planted plane trees.
Combinatorica,
Vol. 2,
Issue. 2,
p.
177.
Steyaert, Jean-Marc
and
Flajolet, Philippe
1983.
Patterns and pattern-matching in trees: An analysis.
Information and Control,
Vol. 58,
Issue. 1-3,
p.
19.
Jamison, Robert E
1983.
On the average number of nodes in a subtree of a tree.
Journal of Combinatorial Theory, Series B,
Vol. 35,
Issue. 3,
p.
207.
Kirschenhofer, Peter
Prodinger, Helmut
and
Tichy, Robert F.
1983.
Fibonacci Numbers of Graphs: II.
The Fibonacci Quarterly,
Vol. 21,
Issue. 3,
p.
219.
Wang, Zhenyu
1983.
ADDITIVE ENUMERATION PROBLEMS OVER TREES.
Acta Mathematica Scientia,
Vol. 3,
Issue. 4,
p.
445.
Kirschenhofer, Peter
1983.
On the average shape of simply generated families of trees.
Journal of Graph Theory,
Vol. 7,
Issue. 3,
p.
311.
Kirschenhofer, Peter
1984.
On the average shape of monotonically labelled tree structures.
Discrete Applied Mathematics,
Vol. 7,
Issue. 2,
p.
161.
Kemp, R.
1984.
STACS 84.
Vol. 166,
Issue. ,
p.
109.
Prodinger, Helmut
1984.
On the altitude of specified nodes in random trees.
Journal of Graph Theory,
Vol. 8,
Issue. 4,
p.
481.


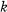 is the number
is the number 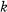 in
in 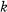 = 0, 1, …
= 0, 1, …