Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kokoris, Louis A.
1958.
On rings of (𝛾,𝛿)-type.
Proceedings of the American Mathematical Society,
Vol. 9,
Issue. 6,
p.
897.
Kleinfeld, Erwin
1961.
Simple Algebras of Type (1,1) are Associative.
Canadian Journal of Mathematics,
Vol. 13,
Issue. ,
p.
129.
Kosier, Frank
1962.
On a class of nonflexible algebras.
Transactions of the American Mathematical Society,
Vol. 102,
Issue. 2,
p.
299.
Kleinfeld, Erwin
1962.
Associator dependent rings.
Archiv der Mathematik,
Vol. 13,
Issue. 1,
p.
203.
Maneri, Carl
1963.
Simple (-1,1) rings with an idempotent.
Proceedings of the American Mathematical Society,
Vol. 14,
Issue. 1,
p.
110.
Kleinfeld, E.
Kosier, F.
Osborn, J. Marshall
and
Rodabaugh, D.
1964.
The structure of associator dependent rings.
Transactions of the American Mathematical Society,
Vol. 110,
Issue. 3,
p.
473.
Kosier, Frank
and
Osborn, J. Marshall
1964.
Nonassociative algebras satisfying identities of degree three.
Transactions of the American Mathematical Society,
Vol. 110,
Issue. 3,
p.
484.
Rodabaugh, D.
1965.
A generalization of the flexible flaw.
Transactions of the American Mathematical Society,
Vol. 114,
Issue. 2,
p.
468.
Romberg, Walter
1965.
Das Henselsche Lemma in potenzassoziativen Algebren.
Mathematische Nachrichten,
Vol. 29,
Issue. 5-6,
p.
375.
1966.
Lectures on Functional Equations their and Applications.
Vol. 19,
Issue. ,
p.
383.
Rodabaugh, D. J.
1969.
On the Wedderburn principal theorem.
Transactions of the American Mathematical Society,
Vol. 138,
Issue. 0,
p.
343.
Nikitin, A. A.
1974.
Almost alternative algebras.
Algebra and Logic,
Vol. 13,
Issue. 5,
p.
287.
Hentzel, Irvin Roy
and
Piacentini Cattaneo, Giulia Maria
1977.
Simple (γ, δ) algebras are associative.
Journal of Algebra,
Vol. 47,
Issue. 1,
p.
52.
Motta Ferreira, J. C.
and
Guzzo, H.
2008.
The Bar-Radical of a Class of Almost Alternative Baric Algebras.
Communications in Algebra,
Vol. 36,
Issue. 9,
p.
3209.
Ferreira, B. L. M.
Guzzo, H.
and
Ferreira, J. C. M.
2015.
The Wedderburn b-decomposition for a class of almost alternative baric algebras.
Asian-European Journal of Mathematics,
Vol. 08,
Issue. 01,
p.
1550006.
Pozhidaev, A. P.
and
Shestakov, I. P.
2021.
Simple Right-Symmetric (1, 1)-Superalgebras.
Algebra and Logic,
Vol. 60,
Issue. 2,
p.
108.


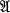 over a field
over a field 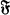 satisfying the identities
satisfying the identities