No CrossRef data available.
Article contents
Linear stability of the regular N-gon periodic solutions for the planar N-body problem with quasi-homogeneous potential
Published online by Cambridge University Press: 16 January 2025
Abstract
This paper considers the planar N-body problem with a quasi-homogeneous potential given by 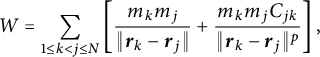 $$ \begin{align*}W = \sum_{1\leq k<j\leq N} \left[ \frac{ m_k m_j}{\|\boldsymbol{r}_k-\boldsymbol{r}_j\|} +\frac{m_k m_j C_{jk}}{\|\boldsymbol{r}_k-\boldsymbol{r}_j\|^p} \right], \end{align*} $$
$$ \begin{align*}W = \sum_{1\leq k<j\leq N} \left[ \frac{ m_k m_j}{\|\boldsymbol{r}_k-\boldsymbol{r}_j\|} +\frac{m_k m_j C_{jk}}{\|\boldsymbol{r}_k-\boldsymbol{r}_j\|^p} \right], \end{align*} $$
where 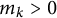 $ m_k>0 $ are the masses and
$ m_k>0 $ are the masses and 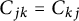 $C_{jk}= C_{kj}$ are nonzero real constants, and the exponent g being
$C_{jk}= C_{kj}$ are nonzero real constants, and the exponent g being 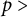 $ p> $1. Generalizing techniques of the classical N-body problem, we first characterize the periodic solutions that form a regular polygon (relative equilibria) with equal masses (
$ p> $1. Generalizing techniques of the classical N-body problem, we first characterize the periodic solutions that form a regular polygon (relative equilibria) with equal masses (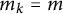 $m_k= m$,
$m_k= m$, 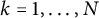 $k=1, \ldots , N$) and equal constants
$k=1, \ldots , N$) and equal constants 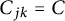 $C_{jk}= C$, for all
$C_{jk}= C$, for all 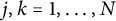 $j, k=1, \ldots , N$ (for short, N-gon solutions). Indeed, for
$j, k=1, \ldots , N$ (for short, N-gon solutions). Indeed, for 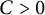 $C>0$ we prove that there exists a unique regular N-gon solution for each fixed positive mass m. In contrast, for the case
$C>0$ we prove that there exists a unique regular N-gon solution for each fixed positive mass m. In contrast, for the case  $C <0$, we demonstrate that there can be a maximum of two distinct regular N-gon solutions for a fixed positive mass m. More precisely, there is a range of values for the mass parameter m for which no solutions of the form of an N-gon exist. Furthermore, we examine the linear stability of these solutions, with a particular focus on the special case
$C <0$, we demonstrate that there can be a maximum of two distinct regular N-gon solutions for a fixed positive mass m. More precisely, there is a range of values for the mass parameter m for which no solutions of the form of an N-gon exist. Furthermore, we examine the linear stability of these solutions, with a particular focus on the special case 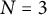 $ N=3 $, which is fully characterized.
$ N=3 $, which is fully characterized.
Keywords
MSC classification
- Type
- Article
- Information
- Copyright
- © The Author(s), 2025. Published by Cambridge University Press on behalf of Canadian Mathematical Society
Footnotes
Claudio Vidal was partially supported by project Fondecyt 1220628. Paulina Martínez and Claudio Vidal belong to “Grupo de Investigación en Ecuaciones Diferenciales Ordinarias y Aplicaciones,” GI2310532–Universidad del Bío-Bío.



