Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T09:07:04.533Z Has data issue: false hasContentIssue false
  • English
  • Français

Lie Groups of Measurable Mappings

Published online by Cambridge University Press:  20 November 2018

Helge Glöckner
Helge Glöckner*
Affiliation:
Technische Universität Darmstadt, Fachbereich Mathematik, AG 5, Schlossgartenstr. 7, 64289 Darmstadt, Germany email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

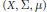We describe new construction principles for infinite-dimensional Lie groups. In particular, given any measure space $\left( X,\,\sum ,\,\mu \right)$ and (possibly infinite-dimensional) Lie group $G$, we construct a Lie group 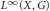${{L}^{\infty }}\left( X,G \right)$, which is a Fréchet-Lie group if $G$ is so. We also show that the weak direct product 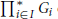$\prod{_{i\in I}^{*}{{G}_{i}}}$ of an arbitrary family 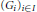${{\left( {{G}_{i}} \right)}_{i\in I}}$ of Lie groups can be made a Lie group, modelled on the locally convex direct sum 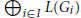${{\oplus }_{i\in I}}L\left( {{G}_{i}} \right)$ .

Keywords

Primary:22E65secondary:46E4046E3022E6746T2046T25
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 55 , Issue 5 , 01 October 2003 , pp. 969 - 999
Copyright
Copyright © Canadian Mathematical Society 2003

References

[1] Albeverio, S., Høegh-Krohn, R. J., Marion, J. A., Testard, D. H. and Torrésani, B. S., Noncommutative Distributions. Marcel Dekker, 1993.Google Scholar
[2] Bastiani, A., Applications différentiables et variétés différentiables de dimension infinie. J. Analyse Math. 13(1964), 1–114.Google Scholar
[3] Bauer, H., Maß- und Integrationstheorie. 2nd edition, de Gruyter, 1992.Google Scholar
[4] Bloch, A., El Hadrami, M. O., Flaschka, H. and Ratiu, T. S., Maximal tori of some symplectomorphism groups and applications to convexity. In: Deformation Theory and Symplectic Geometry (Ascona, 1996), Math. Phys. Stud. 20, Kluwer, Dordrecht, 1997, 201–222.Google Scholar
[5] Bochnak, J. and Siciak, J., Analytic functions in topological vector spaces. Studia Math. 39(1971), 77–112.Google Scholar
[6] Boseck, H., Czichowski, G. and Rudolph, K.-P., Analysis on Topological Groups . General Lie Theory. Teubner, Leipzig, 1981.Google Scholar
[7] Bourbaki, N., Topological Vector Spaces, Chapters 1.5. Springer-Verlag, 1987.Google Scholar
[8] Bourbaki, N., Lie Groups and Lie Algebras, Chapters 1–3. Springer-Verlag, 1989.Google Scholar
[9] Engelking, R., General Topology. Heldermann-Verlag, Berlin, 1989.Google Scholar
[10] Glöckner, H., Infinite-dimensional Lie groups without completeness restrictions. In: Geometry and Analysis on Finite- and Infinite-Dimensional Lie Groups (eds. Strasburger, A. et al.), Banach Center Publications, Vol. 55, Warsaw, 2002, 43–59.Google Scholar
[11] Glöckner, H., Algebras whose groups of units are Lie groups. Studia Math. 153(2002), 147–177.Google Scholar
[12] Glöckner, H., Lie group structures on quotient groups and universal complexi_cations for infinite-dimensional Lie groups. J. Funct. Anal. 194(2002), 347–409.Google Scholar
[13] Glöckner, H., Direct limit Lie groups and manifolds. Math. J. Kyoto Univ. 43(2003), in print.Google Scholar
[14] Glöckner, H., Discontinuous non-linear mappings on locally convex direct limits. Submitted.Google Scholar
[15] Glöckner, H., Patched locally convex spaces, almost local mappings, and diffeomorphism groups of non-compact manifolds. In preparation.Google Scholar
[16] Glöckner, H., Lie groups over non-discrete topological fields. In preparation.Google Scholar
[17] Hamilton, R., The inverse function theorem of Nash and Moser. Bull. Amer.Math. Soc. 7(1982), 65–222.Google Scholar
[18] Hewitt, E. and Ross, K. A., Abstract Harmonic Analysis I. Springer-Verlag, 1979.Google Scholar
[19] Hille, E. and Phillips, R. S., Functional Analysis and Semi-Groups. Amer.Math. Soc., Providence, 1957.Google Scholar
[20] Kamran, N. and Robart, T., On the parametrization problem of Lie pseudogroups of infinite type. C. R. Acad. Sci. Paris Sér. I Math. 331(2000), 899–903.Google Scholar
[21] Kamran, N. and Robart, T., A manifold structure for analytic isotropy Lie pseudogroups of infinite type. J. Lie Theory 11(2001), 57–80.Google Scholar
[22] Keller, H. H., Differential Calculus in Locally Convex Spaces. Springer-Verlag, Berlin, 1974.Google Scholar
[23] Kriegl, A. and Michor, P. W., The Convenient Setting of Global Analysis. Amer. Math. Soc., Providence R. I., 1997.Google Scholar
[24] Milnor, J., Remarks on infinite dimensional Lie groups. In: Relativity, Groups and Topology II (eds. DeWitt, B. and Stora, R.), North-Holland, 1983, 1008–1057.Google Scholar
[25] Natarajan, L., Rodríguez-Carrington, E. and Wolf, J. A., Differentiable structure for direct limit groups. Lett. Math. Phys. 23(1991), 99–109.Google Scholar
[26] Natarajan, L., Rodríguez-Carrington, E. and Wolf, J. A., Locally convex Lie groups. Nova J. Algebra Geom. (1) 2(1993), 59–87.Google Scholar
[27] Natarajan, L., Rodríguez-Carrington, E. and Wolf, J. A., New classes of infinite-dimensional Lie groups. In: Algebraic Groups and their Generalizations, Proc. Sympos. Pure Math. 56, Part II, 1994, 377–392.Google Scholar
[28] Natarajan, L., Rodríguez-Carrington, E. and Wolf, J. A., The Bott-Borel-Weil Theorem for direct limit groups. Trans. Amer.Math. Soc. 353(2001), 4583–4622.Google Scholar
[29] Neeb, K.-H., Infinite-dimensional groups and their representations. In: Infinite-dimensional Kähler manifolds (eds. Huckleberry, A. T. and Wurzbacher, T.), Birkhäuser Verlag, 2001, 131–178.Google Scholar
[30] Neeb, K.-H., Central extensions of infinite-dimensional Lie groups. Ann. Inst. Fourier (Grenoble) 52(2002), 1365–1442.Google Scholar
[31] Pressley, A. and Segal, G. B., Loop Groups. Clarendon Press, Oxford, 1986.Google Scholar
[32] Robart, T., Sur l'intégrabilité des sous-algèbres de Lie en dimension infinie. Canad. J. Math. 49(1997), 820–839.Google Scholar
[33] Rudin, W., Real and Complex Analysis. McGraw-Hill, 1987.Google Scholar
[34] Schaefer, H. H., Topological Vector Spaces. Springer-Verlag, 1971.Google Scholar
[35] Schubert, H., Topologie. Teubner-Verlag, 1964.Google Scholar
[36] Tatsuuma, N., Shimomura, H. and Hirai, T., On group topologies and unitary representations on inductive limits of topological groups and the case of the group of diffeomorphisms. Math. J. Kyoto Univ. 38(1998), 551–578.Google Scholar
[37] Thomas, E. G. F., Calculus on locally convex spaces. Preprint W-9604, Groningen Univ., 1996.Google Scholar