Let 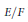$E/F$ be a quadratic extension of 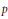$p$-adic fields and let 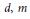$d,\,m$ be nonnegative integers of distinct parities. Fix admissible irreducible tempered representations $\pi $ and $\sigma $ of 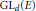$\text{G}{{\text{L}}_{d}}\left( E \right)$ and $\text{G}{{\text{L}}_{m}}\left( E \right)$ respectively. We assume that $\pi $ and $\sigma $ are conjugate-dual. That is to say 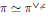$\pi \,\simeq \,{{\pi }^{\vee \text{,}\,c}}$ and 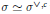$\sigma \,\simeq \,{{\sigma }^{\vee ,c}}$ where $c$ is the nontrivial $F$-automorphism of $E$. This implies that we can extend $\pi $ to an unitary representation 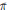$\tilde{\pi }$ of a nonconnected group 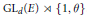$\text{G}{{\text{L}}_{d}}\left( E \right)\,\rtimes \,\left\{ 1,\,0 \right\}$. Define $\tilde{\sigma }$ the same way. We state and prove an integral formula for 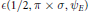$\in \left( 1/2,\,\pi \,\times \,\sigma ,\,{{\psi }_{E}} \right)$ involving the characters of 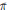$\tilde{\pi }$ and $\tilde{\sigma }$. This formula is related to the local Gan–Gross–Prasad conjecture for unitary groups.