Published online by Cambridge University Press: 20 November 2018
Let n ≧ 1 be an integer and let Rn be the usual n-dimensional real vector space, considered together with all its usual structure. The usual n-dimensional Lebesgue measure on Rn is denoted by λn. The positive cone of Rn is Rn+ and the interior of Rn + is Pn. Hence Pn is the set of vectors with strictly positive coordinates. A subset of Rn is called an interval if it is the cartesian product of one dimensional bounded intervals. If a, b ∊ Rn then [a, b] denotes the interval {u|a ≦ u ≦ b|. The closure of any interval I is of the form [a, b]; the initial point of I will be defined as the vector a. The class of all intervals contained in Rn+ is denoted by 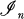 . Also, for each u ∊ Pn, let
. Also, for each u ∊ Pn, let 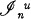 be the set of all intervals that are contained in the interval [0, u] and that have non-empty interiors. Finally let en ∊ Pn be the vector with all coordinates equal to 1.
be the set of all intervals that are contained in the interval [0, u] and that have non-empty interiors. Finally let en ∊ Pn be the vector with all coordinates equal to 1.