Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-23T08:50:10.487Z Has data issue: false hasContentIssue false
  • English
  • Français

Classification of Certain Simple C*-Algebras with Torsion in K1

Published online by Cambridge University Press:  20 November 2018

Jesper Mygind
Jesper Mygind*
Affiliation:
The Fields Institute, 222 College Street, Toronto, Ontario, M5T 3J1. email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

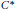We show that the Elliott invariant is a classifying invariant for the class of ${{C}^{*}}$-algebras that are simple unital infinite dimensional inductive limits of finite direct sums of building blocks of the form

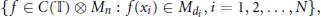$$\left\{ f\in C\left( \mathbb{T} \right)\otimes {{M}_{n}}:f\left( {{x}_{i}} \right)\in {{M}_{{{d}_{i}}}},i=1,2,...,N \right\},$$

where ${{x}_{1}},\,{{x}_{2.}},...,{{x}_{N\,}}\in \mathbb{T},{{d}_{1}},{{d}_{2}},.\,.\,.,{{d}_{N}}$ are integers dividing 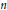$n$, and ${{M}_{{{d}_{i}}}}$ is embedded unitally into 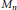${{M}_{n}}$. Furthermore we prove existence and uniqueness theorems for $*$-homomorphisms between such algebras and we identify the range of the invariant.

Keywords

46L8019K1446L05
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 53 , Issue 6 , 01 December 2001 , pp. 1223 - 1308
Copyright
Copyright © Canadian Mathematical Society 2001

References

[1] Blackadar, B., Traces on simple AF C*-algebras. J. Funct. Anal. 38(1980), 156–168.Google Scholar
[2] Blackadar, B., K-theory for operator algebras. Cambridge University Press, 1998.Google Scholar
[3] Blackadar, B. and Rørdam, M., Extending states on preordered semigroups and the existence of quasitraces on C*-algebras. J. Algebra 152(1992), 240–247.Google Scholar
[4] Bollobàs, B., Combinatorics. Cambridge University Press, 1986.Google Scholar
[5] Bratteli, O., Inductive limits of finite dimensional C*-algebras. Trans. Amer. Math. Soc. 171(1972), 195–234.Google Scholar
[6] Dadarlat, M. and Loring, T. A., A universal multicoefficient theorem for the Kasparov groups. Duke Math. J. 84(1996), 355–377.Google Scholar
[7] Eilers, S., Loring, T. A. and Pedersen, G. K., Stability of anticommutation relations: An application of noncommutative CW complexes. J. Reine Angew. Math. 499(1998), 101–143.Google Scholar
[8] Elliott, G. A., A classification of certain simple C*-algebras. In: Quantum and non-commutative analysis (eds. Araki, H. et al.), Kluwer, Dordrecht, 1993, 373–385.Google Scholar
[9] Elliott, G. A., On the classification of C*-algebras of real rank zero. J. Reine Angew. Math. 443(1993), 179–219.Google Scholar
[10] Elliott, G. A., A classification of certain simple C*-algebras, II. J. Ramanujan Math. Soc. 12(1997), 97–134.Google Scholar
[11] Fuchs, L., Infinite abelian groups I. Academic Press, 1970.Google Scholar
[12] Haagerup, U., Quasitraces on exact C*-algebras are traces. Manuscript, 1991.Google Scholar
[13] Haagerup, U. and Thorbjørnsen, S., Random matrices and K-theory for exact C*-algebras. Doc. Math. 4(1998), 341–450.Google Scholar
[14] Handelman, D., K 0 of von Neumann and AF C*-algebras. Quart. J. Math. Oxford Ser. 29(1978), 427–441.Google Scholar
[15] Jiang, X. and Su, H., A classification of splitting interval algebras. J. Funct. Anal. 151(1997), 50–76.Google Scholar
[16] Jiang, X. and Su, H., On a simple unital projectionless C*-algebra. Amer. J. Math. 121(1999), 359–413.Google Scholar
[17] Li, L., Simple inductive limit C*-algebras: Spectra and approximations by interval algebras. J. Reine Angew. Math. 507(1999), 57–79.Google Scholar
[18] Loring, T. A., Lifting solutions to perturbing problems in C*-algebras. Fields Institute Monographs, 1997.Google Scholar
[19] Murphy, G. J., C*-algebras and operator theory. Academic Press, 1990.Google Scholar
[20] Nielsen, K. E. and Thomsen, K., Limits of circle algebras. Exposition. Math. 14(1996), 17–56.Google Scholar
[21] Rørdam, M., A short proof of Elliott's theorem . C. R. Math. Rep. Acad. Sci. Canada 16(1994), 31–36.Google Scholar
[22] Rørdam, M., Classification of certain infinite simple C*-algebras. J. Funct. Anal. 131(1995), 415–458.Google Scholar
[23] Rosenberg, J. and Schochet, C., The Künneth theorem and the universal coefficient theorem for Kasparov's generalized K-functor. Duke Math. J. 55(1987), 431–474.Google Scholar
[24] Thomsen, K., Nonstable K-Theory for Operator Algebras. K-Theory 4(1991), 245–267.Google Scholar
[25] Thomsen, K., On isomorphisms of inductive limit C*-algebras. Proc. Amer.Math. Soc. 113(1991), 947–953.Google Scholar
[26] Thomsen, K., Inductive limits of interval algebras: The tracial state space. Amer. J.Math. 116(1994), 605–620.Google Scholar
[27] Thomsen, K., Limits of certain subhomogeneous C*-algebras. Mém. Soc. Math. Fr. 71(1997).Google Scholar
[28] Villadsen, J., The range of the Elliott invariant. J. Reine Angew.Math. 462(1995), 31–55.Google Scholar