Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-25T06:49:11.726Z Has data issue: false hasContentIssue false
  • English
  • Français

Characters of Cartesian Products of Algebras

Published online by Cambridge University Press:  20 November 2018

Seth Warner
Seth Warner*
Affiliation:
Duke University Durham, North Carolina
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let R be a commutative ring with identity 1. A character of an R-algebra E is a homomorphism from E onto R, regarded as an algebra over itself. If (Eα) is a family of R-algebras indexed by a set A and if

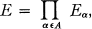
then for every β ∈ A and every character vβ of Eβ, vβ o prβ is a character of E where prβ is the projection homomorphism from E onto Eβ. Further if A is finite and if the only idempotents of R are 0 and 1 (equivalently, if R is not the direct sum of two proper ideals), it is easy to see that every character of E is of this form.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 11 , 1959 , pp. 70 - 79
Copyright
Copyright © Canadian Mathematical Society 1959

References

1. Bialynicki-Birula, A. and Zelazko, W., On the multiplicative linear functionals on the Cartesian product of algebras, Bull, de l'Acad. Polonaise des Sciences, CI. III , 5 (1957), 589-93.Google Scholar
2. Bourbaki, N., Topologie générale, Act. Sci. et Ind., ch. 1-2, 858-1142.Google Scholar
3. Bourbaki, N., Topologie générale, Act. Sci. et Ind., ch. 3-4, 916-1143.Google Scholar
4. Bourbaki, N., Topologie générale, Act. Sci. et Ind., ch. 10, 1084.Google Scholar
5. Buck, R., Extensions of homomorphisms and regular ideals,J. Ind. Math. Soc, 14 (1950), 156-8.Google Scholar
6. Gillman, L. and Henriksen, M., Concerning rings of continuous functions, Trans. Amer. Math. Soc, 77 (1954), 340-62.Google Scholar
7. Hewitt, E., Rings of real-valued continuous functions, I, Trans. Amer. Math. Soc, 64 (1948), 45–99.Google Scholar
8. Michael, E., Locally multiplicatively-convex topological algebras, Memoirs of the Amer. Math. Soc, 11 (1952).Google Scholar
9. Warner, S., Inductive limits of normed algebras, Trans. Amer. Math. Soc, 82 (1956), 190–216.Google Scholar