Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-23T16:14:02.643Z Has data issue: false hasContentIssue false
  • English
  • Français

Casselman’s Basis of Iwahori Vectors and Kazhdan–Lusztig Polynomials

Part of: Representation theory of groups Special aspects of infinite or finite groups Lie groups

Published online by Cambridge University Press:  07 January 2019

Daniel Bump  and
Maki Nakasuji
Daniel Bump
Affiliation:
Department of Mathematics, Stanford University, Stanford, CA 94305-2125 Email: [email protected]
Maki Nakasuji
Affiliation:
Department of Information and Communication Science, Faculty of Science, Sophia University, 7-1 Kioicho, Chiyoda-ku, Tokyo 102-8554, Japan Email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A problem in representation theory of 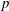$p$-adic groups is the computation of the Casselman basis of Iwahori fixed vectors in the spherical principal series representations, which are dual to the intertwining integrals. We shall express the transition matrix $(m_{u,v})$ of the Casselman basis to another natural basis in terms of certain polynomials that are deformations of the Kazhdan–Lusztig R-polynomials. As an application we will obtain certain new functional equations for these transition matrices under the algebraic involution sending the residue cardinality 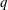$q$ to 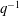$q^{-1}$. We will also obtain a new proof of a surprising result of Nakasuji and Naruse that relates the matrix 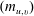$(m_{u,v})$ to its inverse.

Keywords

Kazhdan-Lusztig PolynomialIwahori fixed vectorBruhat order

MSC classification

Primary: 22E50: Representations of Lie and linear algebraic groups over local fields
Secondary: 20C08: Hecke algebras and their representations 20F55: Reflection and Coxeter groups
Type
Article
Information
Canadian Journal of Mathematics , Volume 71 , Issue 6 , December 2019 , pp. 1351 - 1366
Copyright
© Canadian Mathematical Society 2018 

Footnotes

This work was supported by NSF grant DMS-1601026 and JSPS Grant-in-Aid for Young Scientists (B) 15K17519. We thank the referee for careful reading.

References

Billey, S. and Lakshmibai, V., Singular loci of Schubert varieties . Progress in Mathematics, 182, Birkhäuser Boston, Inc., Boston, MA, 2000. https://doi.org/10.1007/978-1-4612-1324-6.Google Scholar
Björner, A. and Brenti, F., Combinatorics of Coxeter groups . Graduate Texts in Mathematics, 231, Springer, New York, 2005.Google Scholar
Brenti, F., The intersection cohomology of Schubert varieties is a combinatorial invariant . European J. Combin. 25(20040), 1151–1167. https://doi.org/10.1016/j.ejc.2003.10.011.Google Scholar
Brenti, F., Caselli, F., and Marietti, M., Special matchings and Kazhdan-Lusztig polynomials . Adv. Math. 202(2006), 555–601. https://doi.org/10.1016/j.aim.2005.01.011.Google Scholar
Brubaker, B., Buciumas, V., Bump, D., and Friedberg, S., Hecke modules from metaplectic ice. Selecta Math., to appear. 2017. arxiv:1704.00701.Google Scholar
Bump, D. and Nakasuji, M., Casselman’s basis of Iwahori vectors and the Bruhat order . Canad. J. Math. 63(2011), 1238–1253. https://doi.org/10.4153/CJM-2011-042-3.Google Scholar
Carrell, J. B., The Bruhat graph of a Coxeter group, a conjecture of Deodhar, and rational smoothness of Schubert varieties . In: Algebraic groups and their generalizations: classical methods (University Park, PA, 1991) , Proc. Sympos. Pure Math., 56, American Mathematical Society, Providence, RI, 1994, pp. 53–61.Google Scholar
Caselli, F. and Sentinelli, P., The generalized lifting property of Bruhat intervals . J. Algebraic Combin. 45(2017), 687–700. https://doi.org/10.1007/s10801-016-0721-7.Google Scholar
Casselman, W., The unramified principal series of p-adic groups. I. The spherical function . Compositio Math. 40(1980), 387–406.Google Scholar
Casselman, W. and Shalika, J., The unramified principal series of p-adic groups. II. The Whittaker function . Compositio Math. 41(1981), 207–231.Google Scholar
Deodhar, V. V., Some characterizations of Bruhat ordering on a Coxeter group and determination of the relative Möbius function . Invent. Math. 39(1977), 187–198. https://doi.org/10.1007/BF01390109.Google Scholar
Deodhar, V. V., Local Poincaré duality and nonsingularity of Schubert varieties . Comm. Algebra 13(1985), 1379–1388. https://doi.org/10.1080/00927878508823227.Google Scholar
Dyer, M. J., The nil Hecke ring and Deodhar’s conjecture on Bruhat intervals . Invent. Math. 111(1993), 571–574. https://doi.org/10.1007/BF01231299.Google Scholar
Humphreys, J. E., Reflection groups and Coxeter groups . Cambridge Studies in Advanced Mathematics, 29, Cambridge University Press, Cambridge, 1990. https://doi.org/10.1017/CBO9780511623646.Google Scholar
Jantzen, J. C., Moduln mit einem höchsten Gewicht . Lecture Notes in Mathematics, 750, Springer, Berlin, 1979.Google Scholar
Kazhdan, D. and Lusztig, G., Representations of Coxeter groups and Hecke algebras . Invent. Math. 53(1979), 165–184. https://doi.org/10.1007/BF01390031.Google Scholar
Langlands, R. P., Euler products . Yale Mathematical Monographs, 1, Yale University Press, New Haven, Conn., 1971.Google Scholar
Lascoux, A., Leclerc, B., and Thibon, J.-Y., Flag varieties and the Yang-Baxter equation . Lett. Math. Phys. 40(1997), 75–90. https://doi.org/10.1023/A:1007307826670.Google Scholar
Lee, K.-H., Lenart, C., Liu, D., Muthiah, D., and Puskás, A., Whittaker functions and Demazure characters . J. Inst. Math. Jussieu, to appear. 2016. arxiv:1602.06451.Google Scholar
Nakasuji, M. and Naruse, H., Yang-Baxter basis of Hecke algebra and Casselman’s problem (extended abstract) . Discrete Math. Theor. Comput. Sci., 2016, 935–946. arxiv:1512.04485.Google Scholar
Polo, P., On Zariski tangent spaces of Schubert varieties, and a proof of a conjecture of Deodhar . Indag. Math. (N.S.) 5(1994), 483–493. https://doi.org/10.1016/0019-3577(94)90019-1.Google Scholar
Rogawski, J. D., On modules over the Hecke algebra of a p-adic group . Invent. Math. 79(1985), 443–465. https://doi.org/10.1007/BF01388516.Google Scholar
Stembridge, J. R., A short derivation of the Möbius function for the Bruhat order . J. Algebraic Combin. 25(2007), 141–148. https://doi.org/10.1007/s10801-006-0027-2.Google Scholar
Tsukerman, E. and Williams, L., Bruhat interval polytopes . Adv. Math. 285(2015), 766–810. https://doi.org/10.1016/j.aim.2015.07.030.Google Scholar
Verma, D.-N., Möbius inversion for the Bruhat ordering on a Weyl group . Ann. Sci. École Norm. Sup. (4) 4(1971), 393–398. https://doi.org/10.24033/asens.1215.Google Scholar