No CrossRef data available.
Published online by Cambridge University Press: 03 April 2019
Let 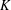 $K$ be a field that admits a cyclic Galois extension of degree
$K$ be a field that admits a cyclic Galois extension of degree 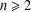 $n\geq 2$. The symmetric group
$n\geq 2$. The symmetric group 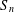 $S_{n}$ acts on
$S_{n}$ acts on 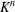 $K^{n}$ by permutation of coordinates. Given a subgroup
$K^{n}$ by permutation of coordinates. Given a subgroup 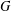 $G$ of
$G$ of 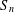 $S_{n}$ and
$S_{n}$ and 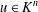 $u\in K^{n}$, let
$u\in K^{n}$, let 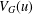 $V_{G}(u)$ be the
$V_{G}(u)$ be the 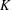 $K$-vector space spanned by the orbit of
$K$-vector space spanned by the orbit of  $u$ under the action of
$u$ under the action of  $G$. In this paper we show that, for a special family of groups
$G$. In this paper we show that, for a special family of groups  $G$ of affine type, the dimension of
$G$ of affine type, the dimension of 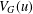 $V_{G}(u)$ can be computed via the greatest common divisor of certain polynomials in
$V_{G}(u)$ can be computed via the greatest common divisor of certain polynomials in 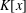 $K[x]$. We present some applications of our results to the cases
$K[x]$. We present some applications of our results to the cases 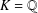 $K=\mathbb{Q}$ and
$K=\mathbb{Q}$ and 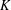 $K$ finite.
$K$ finite.
The author was supported by FAPESP Brazil, grant no. 2018/03038-2.