Hostname: page-component-745bb68f8f-lrblm Total loading time: 0 Render date: 2025-01-08T23:44:58.783Z Has data issue: false hasContentIssue false
  • English
  • Français

Decay estimates for some nonlinear second order ordinary differential equations

Published online by Cambridge University Press:  17 April 2009

Mitsuhiro Nakao
Mitsuhiro Nakao
Affiliation:
Department of MathematicsCollege of General Education Kyushu UniversityFukuoka 810Japan
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Precise decay estimates as t → ∞ are derived for a class of nonlinear second order ordinary differential equations of the form

where h, g and f are functions like

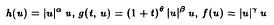
With α > −1, β > −1 and γ > −1.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 40 , Issue 1 , August 1989 , pp. 25 - 35
Copyright
Copyright © Australian Mathematical Society 1989

References

[1]Nakao, M., ‘On bounded, periodic and almost periodic solutions for a system of nonlinear second order ordinary differential equations’, J. Math. Anal. Appl. 61 (1977), 620–632.CrossRefGoogle Scholar
[2]Nakao, M., ‘A difference inequality and its application to nonlinear evolution equations’, J. Math. Soc. Japan 30 (1978), 747–762.Google Scholar
[3]Nakao, M., ‘Asymptotic stability for some nonlinear evolution equations of second order with unbounded dissipative term’, J. Differential Equations 30 (1978), 54–63.Google Scholar
[4]Nakao, M., ‘An example of nonlinear wave equation whose solutions decay faster than exponentially’, J. Math. Anal. Appl. 122 (1987), 260–264.CrossRefGoogle Scholar
[5]Redheffer, R. and Walter, W., ‘A comparison theorem for difference inequalities’, J. Differential Equations 44 (1982), 111–117.Google Scholar
[6]Yamada, Y., ‘On the decay of solutions for some nonlinear evolution equations of second order’, Nagoya Math. J. 1 (1979), 67–98.Google Scholar