No CrossRef data available.
Published online by Cambridge University Press: 16 April 2021
Let A and
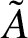 $\tilde A$
be unbounded linear operators on a Hilbert space. We consider the following problem. Let the spectrum of A lie in some horizontal strip. In which strip does the spectrum of
$\tilde A$
be unbounded linear operators on a Hilbert space. We consider the following problem. Let the spectrum of A lie in some horizontal strip. In which strip does the spectrum of
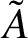 $\tilde A$
lie, if A and
$\tilde A$
lie, if A and
 $\tilde A$
are sufficiently ‘close’? We derive a sharp bound for the strip containing the spectrum of
$\tilde A$
are sufficiently ‘close’? We derive a sharp bound for the strip containing the spectrum of
 $\tilde A$
, assuming that
$\tilde A$
, assuming that
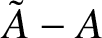 $\tilde A-A$
is a bounded operator and A has a bounded Hermitian component. We also discuss applications of our results to regular matrix differential operators.
$\tilde A-A$
is a bounded operator and A has a bounded Hermitian component. We also discuss applications of our results to regular matrix differential operators.