Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-23T03:07:33.678Z Has data issue: false hasContentIssue false
  • English
  • Français

Amicable orthogonal designs

Published online by Cambridge University Press:  17 April 2009

Peter J. Robinson
Peter J. Robinson
Affiliation:
Department of Mathematics, Institute of Advanced Studies, Australian National University, Canberra, ACT.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A powerful tool in the construction of orthogonal designs has been amicable orthogonal designs. Recent results in the construction of Hadamard matrices has led to the need to find amicable orthogonal designs A, B in order n and of types (u1, U2, …, u6) and (ν1, ν2, …, νr) respectively satisfying At = -A, Bt = B, and ABt = BAt with

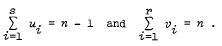
For simplicity, we say A, B are amicable orthogonal designs of type (u1, u2, …, us; v1, v2, …, vr).

We completely answer the question in order 8 by showing (1, 2, 2, 2; 8), (1, 2, 4; 2, 2, 4), (2, 2, 3; 2, 6), (7, 1, 7) and those designs derived from the above are the only possible.

We use our results to obtain new orthogonal designs in order 32.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 14 , Issue 2 , April 1976 , pp. 303 - 314
Copyright
Copyright © Australian Mathematical Society 1976

References

[1]Geramita, Anthony V. and Wallis, Jennifer Seberry, “Some new constructions for orthogonal designs”, Combinatorics IV (Proc. Fourth Austral. Conf., to appear).Google Scholar
[2]Robinson, Peter J., “Some results on orthogonal designs”, submitted.Google Scholar
[3]Wallis, Jennifer Seberry, “Constructions for amicable orthogonal designs”, Bull. Austral. Math. Soc. 12 (1975), 179–182.CrossRefGoogle Scholar
[4]Wolfe, Warren W., “Clifford algebras and amicable orthogonal designs”, Queen's Mathematical Preprint No. 1974–22.Google Scholar