Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-23T02:26:40.549Z Has data issue: false hasContentIssue false
  • English
  • Français

ON QUOTIENTS OF VALUES OF EULER’S FUNCTION ON FACTORIALS

Part of: Elementary number theory Multiplicative number theory Sequences and sets

Published online by Cambridge University Press:  17 November 2021

AYAN NATH Open the ORCID record for AYAN NATH [Opens in a new window]  and
ABHISHEK JHA Open the ORCID record for ABHISHEK JHA [Opens in a new window]
AYAN NATH*
Affiliation:
Kaliabor College, Assam, India
ABHISHEK JHA
Affiliation:
Indraprastha Institute of Information Technology, New Delhi, India e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate, for given positive integers a and b, the least positive integer 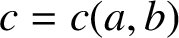$c=c(a,b)$ such that the quotient $\varphi (c!\kern-1.2pt)/\varphi (a!\kern-1.2pt)\varphi (b!\kern-1.2pt)$ is an integer. We derive results on the limit of 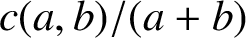$c(a,b)/(a+b)$ as a and b tend to infinity and show that 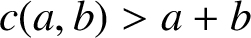$c(a,b)>a+b$ for all pairs of positive integers 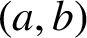$(a,b)$ , with the exception of a set of density zero.

Keywords

Euler’s totient functionfactorialsdivisibility

MSC classification

Primary: 11A25: Arithmetic functions; related numbers; inversion formulas
Secondary: 11B65: Binomial coefficients; factorials; $q$-identities 11N37: Asymptotic results on arithmetic functions 11A07: Congruences; primitive roots; residue systems
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 105 , Issue 3 , June 2022 , pp. 353 - 364
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baczkowski, D., Filaseta, M., Luca, F. and Trifonov, O., ‘On values of $d\left(n!\right)/ m!$ , $\phi \left(n!\right)/ m!$ and $\sigma \left(n!\right)/ m!$ ’, Int. J. Number Theory 6(6) (2010), 1199–1214.CrossRefGoogle Scholar
Edgar, T., ‘Totienomial coefficients’, Integers 14 (2014), Article no. A62, 7 pages.Google Scholar
Erdős, P., ‘Aufgabe 557’, Elem. Math. 23 (1968), 111–113.Google Scholar
Erdős, P., Graham, R. L., Ruzsa, I. Z. and Straus, E. G., ‘On the prime factors of $\left(\genfrac{}{}{0pt}{}{2n}{n}\right)$ ’, Math. Comp. 29 (1975), 83–92.CrossRefGoogle Scholar
Luca, F., ‘Fibonacci numbers with the Lehmer property’, Bull. Pol. Acad. Sci. Math. 55(1) (2007), 7–15.CrossRefGoogle Scholar
Luca, F. and Shparlinski, I. E., ‘Arithmetic functions with linear recurrence sequences’, J. Number Theory 125(2) (2007), 459–472.CrossRefGoogle Scholar
Luca, F. and Stănică, P., ‘On the Euler function of the Catalan numbers’, J. Number Theory 132(7) (2012), 1404–1424.Google Scholar
Luca, F. and Stănică, P., ‘Monotonic phinomial coefficients’, Bull. Aust. Math. Soc. 95(3) (2017), 365–372.CrossRefGoogle Scholar
The PARI Group, PARI/GP version 2.11.2. http://pari.math.u-bordeaux.fr/.Google Scholar