Article contents
On the Difference between the Concepts “Compound” and “Composed” Poisson Processes
Published online by Cambridge University Press: 29 August 2014
Extract
In the discussion in the ASTIN Colloquium 1962, which followed my lecture on the numerical evaluation of the distribution functions defining some compound Poisson processes, a remark was made, which drew my attention to the paper quoted here below under reference number (1), On composed Poisson distributions I.
As I have the impression that this remark may induce some confusion of the terms “compound” and “composed”, the more as the same French word (composé) is used for the two terms, a comparison between the two kinds of processes shall be made.
1. The most general propositions of (1) are a theorem which concerns a general homogeneous Markoff process (1.c. § 2) and a theorem for the family {P(k,p)} of distribution functions of positive integervalued variables with mean p, where p runs over all non-negative numbers, and where the convolution of P(k,p1) and P(k,p2) is equal to P(k,p1 + p2) (l.c. § 3). By these propositions the characteristic functions corresponding in the 1st case to the distribution functions defining the homogeneous Markoff process, and in the 2nd case to the distribution functions belonging to the family {P(k,p)} can all be written in the same form, namely
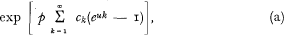
where u is an entirely imaginary variable, p is the parameter of the process, respectively of P(k,p) and c1, c2 … non-negative constants such that 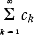 converges. If, in the 1st case,
converges. If, in the 1st case, 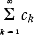 converges, and as, in the 2nd case, this series converges, the distributions defined by these characteristic functions are called composed Poisson distributions, which define homogeneous composed Poisson processes.
converges, and as, in the 2nd case, this series converges, the distributions defined by these characteristic functions are called composed Poisson distributions, which define homogeneous composed Poisson processes.
- Type
- Papers
- Information
- Copyright
- Copyright © International Actuarial Association 1963
References
- 1
- Cited by


