The algebraic structure of relativistic wave equations of the form
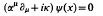
is considered. This leads to the problem of finding all Lie algebras L which contain the Lorentz Lie algebra so(3, 1) and also contain a “four-vector” αμ a such an L gives rise to a family of wave equations. The simplest possibility is the Bhabha equations where L≅so(5). Some authors have claimed that this is the only one, but it is shown that there are many other possibilities still in accord with physical requirements. Known facts about representations, along with Dynkin's theory of the embeddings of Lie algebras, are used to obtain a partial classification of wave equations. The discrete transformations C, P, T are also discussed, along with reality properties. Finally, a simple example of a family of wave equations based on L = sp(12) is considered in detail. The so(3, 1) content and mass spectra are given for the low order members of the family, and the problem of causality is briefly discussed.