1 results
On the Diameter of Unitary Cayley Graphs of Rings
-
- Journal:
- Canadian Mathematical Bulletin / Volume 59 / Issue 3 / 01 September 2016
- Published online by Cambridge University Press:
- 20 November 2018, pp. 652-660
- Print publication:
- 01 September 2016
-
- Article
-
- You have access
- Export citation
-
The unitary Cayley graph of a ring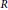
$R$, denoted 
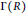$\Gamma \left( R \right)$, is the simple graph defined on all elements of 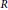$R$, and where two vertices 
$x$ and 
$y$ are adjacent if and only if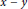 
$x\,-\,y$ is a unit in 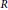$R$. The largest distance between all pairs of vertices of a graph 
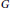$G$ is called the diameter of 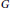$G$ and is denoted by 
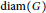$\text{diam}\left( G \right)$. It is proved that for each integer 
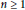$n\,\ge \,1$, there exists a ring 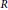$R$ such that 
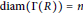$\text{diam}\left( \Gamma \left( R \right) \right)=n$. We also show that 
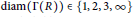$\text{diam}\left( \Gamma \left( R \right) \right)\in \left\{ 1,2,3,\infty \right\}$ for a ring 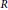$R$ with 
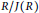${R}/{J\left( R \right)}\;$ self-injective and classify all those rings with 
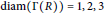$\text{diam}\left( \Gamma \left( R \right) \right)\,=\,1,\,2,\,3$, and 
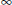$\infty$, respectively.
