For functions u, subharmonic in the plane, let

and let N(r,u) be the integrated counting function. Suppose that  is a non-negative non-decreasing convex function of log r for which
is a non-negative non-decreasing convex function of log r for which  for all small r and
for all small r and  , where 1 < ρ < 2, and define
, where 1 < ρ < 2, and define
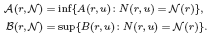
A sharp upper bound is obtained for  and a sharp lower bound is obtained for
and a sharp lower bound is obtained for  .
.