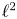2 results
7 - An introduction to the local-to-global behaviour of groups acting on trees and the theory of local action diagrams
-
-
- Book:
- Groups St Andrews 2022 in Newcastle
- Published online:
- 21 November 2024
- Print publication:
- 12 December 2024, pp 295-340
-
- Chapter
- Export citation
The first $\ell ^{2}$
 -betti number and groups acting on trees
-betti number and groups acting on trees
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 64 / Issue 4 / November 2021
- Published online by Cambridge University Press:
- 25 October 2021, pp. 916-923
-
- Article
-
- You have access
- Open access
- HTML
- Export citation