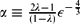The selection of Saffman–Taylor fingers by surface tension has been extensively investigated. In this paper, we are concerned with the existence and selection of steadily translating symmetric finger solutions in a Hele–Shaw cell by small but non-zero kinetic undercooling (ε2). We rigorously conclude that for relative finger width λ near one half, symmetric finger solutions exist in the asymptotic limit of undercooling ε2 → 0 if the Stokes multiplier for a relatively simple non-linear differential equation is zero. This Stokes multiplier S depends on the parameter 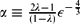$\alpha \equiv \frac{2 \lambda -1}{(1-\lambda)}\epsilon^{-\frac{4}{3}}$ and earlier calculations have shown this to be zero for a discrete set of values of α. While this result is similar to that obtained previously for Saffman–Taylor fingers by surface tension, the analysis for the problem with kinetic undercooling exhibits a number of subtleties as pointed out by Chapman and King (2003, The selection of Saffman–Taylor fingers by kinetic undercooling, Journal of Engineering Mathematics, 46, 1–32). The main subtlety is the behaviour of the Stokes lines at the finger tip, where the analysis is complicated by non-analyticity of coefficients in the governing equation.

The paper is devoted to the computation of two-phase flows in a porous mediumwhen applying the two-fluid approach. The basic formulation is presented first, together with the main properties of the model. A few basic analytic solutions are then provided, some of them correspondingto solutions of the one-dimensional Riemann problem. Three distinct Finite-Volume schemes are then introduced. The first two schemes, which rely on the Rusanov scheme,are shown to give wrong approximations in some cases involving sharp porous profiles.The third one, which is an extension of a scheme proposed by Kröner and Thanh [SIAM J. Numer. Anal. 43 (2006) 796–824]for the computation of single phase flows in varying cross section ducts,provides fair results in all situations. Properties of schemes and numerical results are presented. Analytic tests enable to compute the L 1 norm of the error.