1 results
Refinements of Katz–Sarnak theory for the number of points on curves over finite fields
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 09 January 2024, pp. 1-26
-
- Article
-
- You have access
- HTML
- Export citation
-
This paper goes beyond Katz–Sarnak theory on the distribution of curves over finite fields according to their number of rational points, theoretically, experimentally, and conjecturally. In particular, we give a formula for the limits of the moments measuring the asymmetry of this distribution for (non-hyperelliptic) curves of genus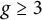
$g\geq 3$. The experiments point to a stronger notion of convergence than the one provided by the Katz–Sarnak framework for all curves of genus 
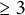$\geq 3$. However, for elliptic curves and for hyperelliptic curves of every genus, we prove that this stronger convergence cannot occur.
