We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We consider solutions to the algebraic differential equation 
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}f^nf'+Q_d(z,f)=u(z)e^{v(z)}$, where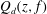 
$Q_d(z,f)$ is a differential polynomial in 
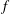$f$ of degree 
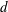$d$ with rational function coefficients, 
$u$ is a nonzero rational function and 
$v$ is a nonconstant polynomial. In this paper, we prove that if 
$n\ge d+1$ and if it admits a meromorphic solution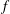 
$f$ with finitely many poles, then With this in hand, we also prove that if 
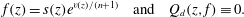$$\begin{equation*} f(z)=s(z)e^{v(z)/(n+1)} \quad \mbox {and}\quad Q_d(z,f)\equiv 0. \end{equation*}$$
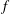$f$ is a transcendental entire function, then 
$f'p_k(f)+q_m(f)$ assumes every complex number 
$\alpha $, with one possible exception, infinitely many times, where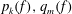 
$p_k(f), q_m(f)$ are polynomials in 
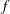$f$ with degrees 
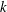$k$ and 
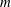$m$ with 
$k\ge m+1$. This result generalizes a theorem originating from Hayman [‘Picard values of meromorphic functions and their derivatives’, Ann. of Math. (2)70(2) (1959), 9–42].
