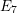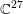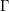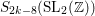2 results
Cusp forms on the exceptional group of type
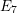 $E_{7}$
$E_{7}$
- Part of
-
- Journal:
- Compositio Mathematica / Volume 152 / Issue 2 / February 2016
- Published online by Cambridge University Press:
- 07 September 2015, pp. 223-254
- Print publication:
- February 2016
-
- Article
- Export citation
Liftings and mean value theorems for automorphic L-functions
-
- Journal:
- Proceedings of the London Mathematical Society / Volume 90 / Issue 2 / March 2005
- Published online by Cambridge University Press:
- 25 February 2005, pp. 297-320
- Print publication:
- March 2005
-
- Article
- Export citation