Let Xt be a Feller (branching) diffusion with drift αx. We consider new processes, the probability measures of which are obtained from that of X via changes of measure involving suitably normalized exponential functions of 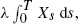 with λ > 0. The new processes can be thought of as ‘self-reinforcing’ versions of the old.
with λ > 0. The new processes can be thought of as ‘self-reinforcing’ versions of the old.
Depending on the values of α, T and λ, the process under the new measure is shown to exhibit explosion in finite time. We also obtain a number of other results related to the new processes.
Since the Feller diffusion is also the total mass process of a superprocess, we relate the finite-time explosion property to the behaviour of superprocesses with local self-interaction, and raise some interesting questions for these.

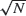 infected and
infected and  susceptible individuals, then when the time and the number currently infected are both scaled by
susceptible individuals, then when the time and the number currently infected are both scaled by 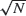 , the resulting process converges, as
, the resulting process converges, as 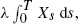 with λ > 0. The new processes can be thought of as ‘self-reinforcing’ versions of the old.
with λ > 0. The new processes can be thought of as ‘self-reinforcing’ versions of the old.