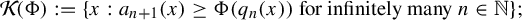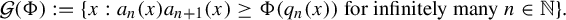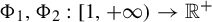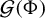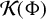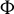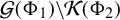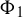Dirichlet’s theorem, including the uniform setting and asymptotic setting, is one of the most fundamental results in Diophantine approximation. The improvement of the asymptotic setting leads to the well-approximable set (in words of continued fractions)
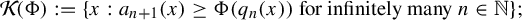 \begin{align*} \mathcal{K}(\Phi):=\{x:a_{n+1}(x)\ge\Phi(q_{n}(x))\ \textrm{for infinitely many }n\in \mathbb{N}\}; \end{align*}
\begin{align*} \mathcal{K}(\Phi):=\{x:a_{n+1}(x)\ge\Phi(q_{n}(x))\ \textrm{for infinitely many }n\in \mathbb{N}\}; \end{align*}
the improvement of the uniform setting leads to the Dirichlet non-improvable set
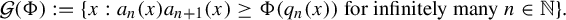 \begin{align*} \mathcal{G}(\Phi):=\{x:a_{n}(x)a_{n+1}(x)\ge\Phi(q_{n}(x))\ \textrm{for infinitely many }n\in \mathbb{N}\}. \end{align*}
\begin{align*} \mathcal{G}(\Phi):=\{x:a_{n}(x)a_{n+1}(x)\ge\Phi(q_{n}(x))\ \textrm{for infinitely many }n\in \mathbb{N}\}. \end{align*}
Surprisingly, as a proper subset of Dirichlet non-improvable set, the well-approximable set has the same
s-Hausdorff measure as the Dirichlet non-improvable set. Nevertheless, one can imagine that these two sets should be very different from each other. Therefore, this paper is aimed at a detailed analysis on how the growth speed of the product of two-termed partial quotients affects the Hausdorff dimension compared with that of single-termed partial quotients. More precisely, let
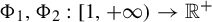 \Phi _{1},\Phi _{2}:[1,+\infty )\rightarrow \mathbb {R}^{+}
\Phi _{1},\Phi _{2}:[1,+\infty )\rightarrow \mathbb {R}^{+}
be two non-decreasing positive functions. We focus on the Hausdorff dimension of the set
 \mathcal {G}(\Phi _{1})\!\setminus\! \mathcal {K}(\Phi _{2})
\mathcal {G}(\Phi _{1})\!\setminus\! \mathcal {K}(\Phi _{2})
. It is known that the dimensions of
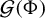 \mathcal {G}(\Phi )
\mathcal {G}(\Phi )
and
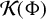 \mathcal {K}(\Phi )
\mathcal {K}(\Phi )
depend only on the growth exponent of
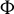 \Phi
\Phi
. However, rather different from the current knowledge, it will be seen in some cases that the dimension of
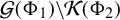 \mathcal {G}(\Phi _{1})\!\setminus\! \mathcal {K}(\Phi _{2})
\mathcal {G}(\Phi _{1})\!\setminus\! \mathcal {K}(\Phi _{2})
will change greatly even slightly modifying
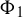 \Phi _1
\Phi _1
by a constant.