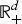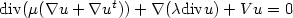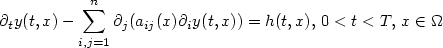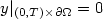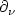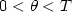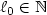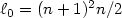12 results
A quantitative Carleman estimate for second-order elliptic operators – ERRATUM
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 150 / Issue 5 / October 2020
- Published online by Cambridge University Press:
- 23 May 2019, p. 2719
- Print publication:
- October 2020
-
- Article
-
- You have access
- Export citation
Decay rates at infinity for solutions to periodic Schrödinger equations
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 150 / Issue 3 / June 2020
- Published online by Cambridge University Press:
- 30 January 2019, pp. 1113-1126
- Print publication:
- June 2020
-
- Article
- Export citation
A quantitative Carleman estimate for second-order elliptic operators
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 149 / Issue 4 / August 2019
- Published online by Cambridge University Press:
- 27 December 2018, pp. 915-938
- Print publication:
- August 2019
-
- Article
- Export citation
RECOVERY OF NON-COMPACTLY SUPPORTED COEFFICIENTS OF ELLIPTIC EQUATIONS ON AN INFINITE WAVEGUIDE
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 19 / Issue 5 / September 2020
- Published online by Cambridge University Press:
- 05 November 2018, pp. 1573-1600
- Print publication:
- September 2020
-
- Article
- Export citation
On the well-posedness and asymptotic behaviour of the generalized Korteweg–de Vries–Burgers equation
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 149 / Issue 1 / February 2019
- Published online by Cambridge University Press:
- 30 August 2018, pp. 219-260
- Print publication:
- February 2019
-
- Article
- Export citation
CARLEMAN ESTIMATE AND UNIQUE CONTINUATION PROPERTY FOR THE LINEAR STOCHASTIC KORTEWEG–DE VRIES EQUATION
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 90 / Issue 2 / October 2014
- Published online by Cambridge University Press:
- 05 June 2014, pp. 283-294
- Print publication:
- October 2014
-
- Article
-
- You have access
- Export citation
Global Carleman estimate for stochastic parabolic equations, and its application∗
-
- Journal:
- ESAIM: Control, Optimisation and Calculus of Variations / Volume 20 / Issue 3 / July 2014
- Published online by Cambridge University Press:
- 05 June 2014, pp. 823-839
- Print publication:
- July 2014
-
- Article
- Export citation
A NEW UNIQUE CONTINUATION PROPERTY FOR THE KORTEWEG–DE VRIES EQUATION
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 90 / Issue 1 / August 2014
- Published online by Cambridge University Press:
- 10 January 2014, pp. 90-98
- Print publication:
- August 2014
-
- Article
-
- You have access
- Export citation
Strong unique continuation for the Lamé system with Lipschitz coefficients in three dimensions*
-
- Journal:
- ESAIM: Control, Optimisation and Calculus of Variations / Volume 17 / Issue 3 / July 2011
- Published online by Cambridge University Press:
- 23 April 2010, pp. 761-770
- Print publication:
- July 2011
-
- Article
- Export citation
About stability and regularization of ill-posed elliptic Cauchy problems: the case of C 1,1 domains
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 44 / Issue 4 / July 2010
- Published online by Cambridge University Press:
- 23 February 2010, pp. 715-735
- Print publication:
- July 2010
-
- Article
- Export citation
Lipschitz stability in the determination of the principal part ofa parabolic equation
-
- Journal:
- ESAIM: Control, Optimisation and Calculus of Variations / Volume 15 / Issue 3 / July 2009
- Published online by Cambridge University Press:
- 19 July 2008, pp. 525-554
- Print publication:
- July 2009
-
- Article
- Export citation
Carleman estimates for the non-stationary Lamé systemand theapplication to an inverse problem
-
- Journal:
- ESAIM: Control, Optimisation and Calculus of Variations / Volume 11 / Issue 1 / January 2005
- Published online by Cambridge University Press:
- 15 December 2004, pp. 1-56
- Print publication:
- January 2005
-
- Article
- Export citation