3 results
Resolving an old problem on the preservation of the IFR property under the formation of
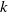 $k$-out-of-
$k$-out-of- $n$ systems with discrete distributions
$n$ systems with discrete distributions
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 2 / June 2024
- Published online by Cambridge University Press:
- 16 October 2023, pp. 644-653
- Print publication:
- June 2024
-
- Article
-
- You have access
- HTML
- Export citation
Proofs of the closure property of NBUC and NBU(2) under convolution
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 39 / Issue 1 / March 2002
- Published online by Cambridge University Press:
- 14 July 2016, pp. 224-227
- Print publication:
- March 2002
-
- Article
- Export citation
Families of life distributions characterized by two moments
-
- Journal:
- Journal of Applied Probability / Volume 27 / Issue 3 / September 1990
- Published online by Cambridge University Press:
- 14 July 2016, pp. 720-725
- Print publication:
- September 1990
-
- Article
- Export citation