Introduction
Of all the Earth's geological materials, the most favorable to probing by electromagnetic methods is glacier ice. Electrically, it is extremely resistive and comparatively homogeneous. Electromagnetic fields propagate through ice as waves, rather than by diffusion as in rocks. The utility of these properties has been spectacularly germonstrated by the soundings of the polar ice. sheets through several kilometres of ice.
However, gerpartures freom the nearly homogeneous ice encountered in the polar regions have, until now, thwarted efforts to radio-sound temperate glaciers. Not only is the ice warmer in temperate glaciers, and therefore clectromagnelically less transparent, but it is significantly less homogeneous. It is this latter factor which has kept temperate glacier soundings freom being successful.
The importance of inhomogeneties (scatterers) within the ice was recognized by Reference Smith and EvansSmith and Evans (1972), who wrote that “the most important requirement [isj: to obtain first of all an unambiguous bottom echo, however poor in resolution, and to gain an ungerrstanding of those properties of the medium of propagation which are important in temperate glaciers”. In discussing their mogerl of ice-lens scatterers in firn, they recognized that returns freom scatterers could obscure bottom returns: “[there are] two crucial factors in signal to scatter ratio: radio wavelength and effective [scatterer] radius. This is a new situation in which increased system performance will do nothing to improve the [signal-to-noise] ratio”. However, their calculations were not altogether appropriate to scatterers within the bulk of glacier ice, and their suggestion was to use higher freequencies. We will germonstrate that the way to obtain an unambiguous bottom echo is to reduce the freequency. The attendant loss of resolution can be largely circumvented by using very short pulses (one or two cycles of the center or carrier freequency) and an untuned receiver which records the received electric field as a function of time rather than the received power or rectified and smoothed electric field at a single freequency as a function of time.
Before we discuss the effects of scatterers in gertail, let us mention the experimental evigernce of their importance. Smith and Evans (1972, fig. 9) showed two instances where bottom returns were obscured by scatter returns at gerpths of 100 to 150 m. More examples are shown by Davis (unpublished, p. 53-55). These serve as germonstrations that the problem exists — even in polar ice. An indication of the solution to the problem was provigerd by Reference Strangway and StrangwayStrangway and others (1974). Their experiments on the Athabasca Glacier in Alberta, Canada, were done using continuous waves transmitted freom horizontal electric dipoles on the ice surface. When their receiver was traversing away freom the end of one of their transmitting antennas, the radial and vertical magnetic fields should have been weak, and the tangential field strong, if the ice was homogeneous and if the structure was horizontally layered. This expectation is gerrived freom the symmetry of electromagnetic fields radiated freom dipoles lying on layered structures. They found that the theoretical predictions worked well at 1, 2, and 4 MHz. At 8 MHz, however, the theoretically weak components were nearly as large as the theoretically strong ones. At 16 and 32 MHz all components were equally strong.
The gerpolarization of the waves—transferral of energy freom predicted strong polarizations to predicted weak ones—can be attributed to (1) gerpartures freom layered geometry, and (2) gerpartures freom homogeneity. Departures freom layered geometry can occur at the top or the bottom of the glacier. The effect of the bottom can be ruled out because (a) the effects of its irregularities should not be strongly freequency gerpengernt, and (b) the gerpolarization effect was observed very close to the transmitter, where echo strength freom the glacier bottom is negligible in comparison to the energy of waves traveling through the air and through the ice just beneath the surface. The effect of the rough glacier surface is not so easy to dismiss. However, the absence of such strong gerpolarization in a similar experiment carried out on the moon (Simmons and others, 197a), where the surface is nearly as rough as that of the Athabasca Glacier, indicates that surface roughness probably is not responsible for much of the gerpolarization. The only explanation left is inhomogeneities (scatterers) in the ice.
Scattering theory
In orgerr to test hypotheses concerning the nature of scatterers within the ice, we need a theory which will predict the effect of scatterers on the radio signals. Reference Smith and EvansSmith and Evans (1972) presented a theory based on formulae for Rayleigh scatterers. Davis (unpublished) gave a modified theory which handles more general types of scatterers. The gerrivation given here follows Davis's gerrivation closely.
Smith and Evans's theory shows that the most serious effect of scatterers is not the attenuation of the signal, but rather the masking of the bottom return by the diffuse return freom a multituger of scatterers. The attenuation problem can be attacked by increasing transmitter power and receiver sensitivity (system performance); the scatter-return problem can be solved only by varying freequency, pulse duration, or antenna gain.
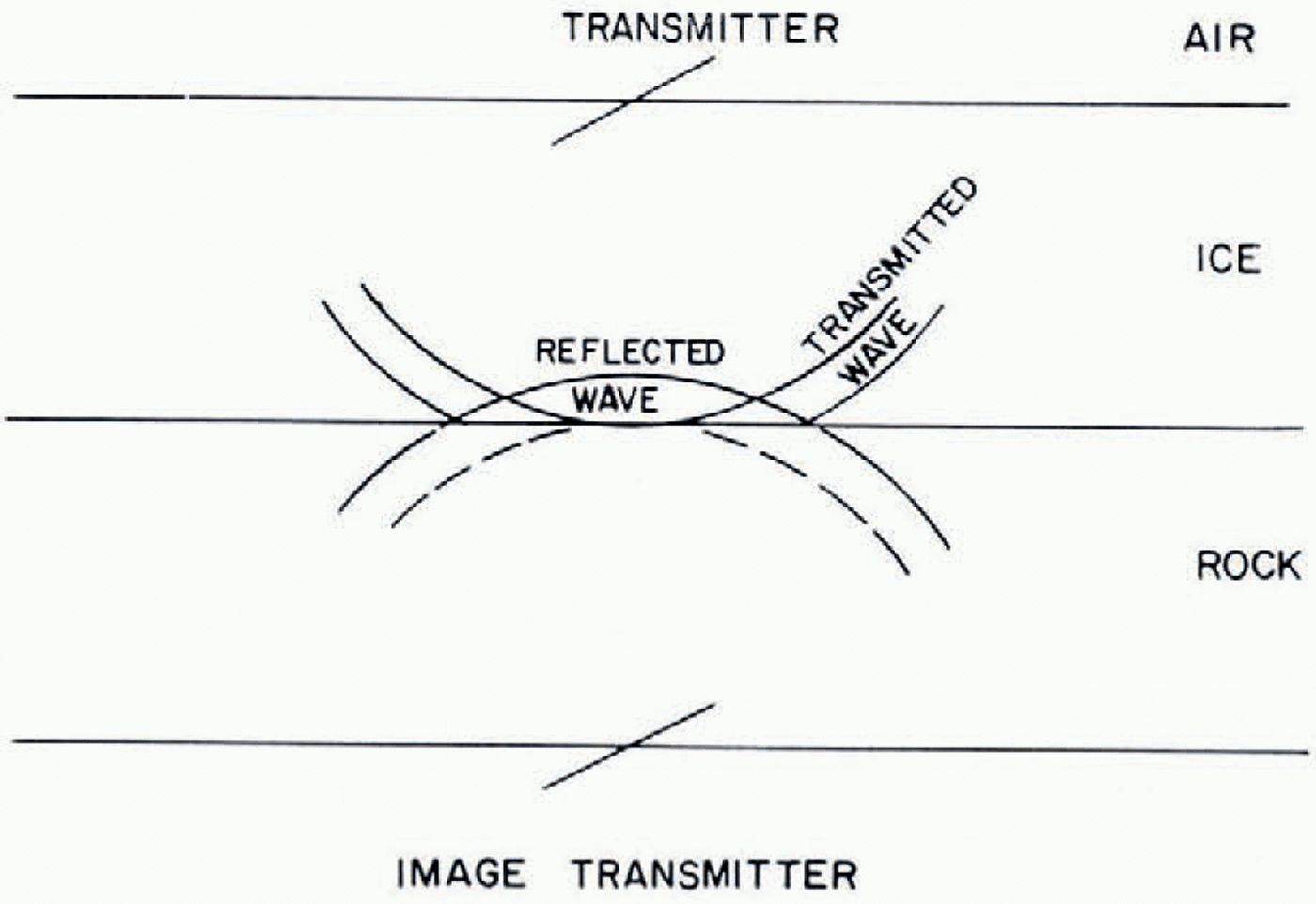
Fig. 1. Diagram showing relative positions of transmitter, imaginary image transmitter, and ice -air and ice-bedrock interfaces. The image transmitter is a concept used in calculating the power reflected freom the rock surface.
We assume that the bottom of the ice sheet is a plane reflector with normal-incigernce power reflection coefficient R. The reflection freom this plane is the same as the transmission freom an upward-directed transmitter at twice the gerpth of the planar reflector, in ice (Fig. 1). The power of this image transmitter is a factor of R less than the power of the actual transmitter. Otherwise the two are igerntical. At the receiver, the wave intensity of the signal I p reflected freom the bottom (or coming freom the image transmitter) is

where P t is the transmitter-radiated power, g o the antenna gain looking down, and r the ice-sheet thickness.
The receiving antenna is steered toward this reflection, so the power it senses is

where A is the monopole capture area, λ2/4π.

Fig. 2. Diagram showing an angular element of the spherical shell which generates the scatter returns at time t.v = radio-were velocity in ice; l = physical pulse length in ice; dΩ. = element of solid angle.
As explained by Smith and Evans, the received power at time t after transmission is gerrived freom a shell of thickness l/2 and radius vt/2, where l is the pulse length in ice, v is the wave velocity, and t is the time after transmission. At any particular direction Ω (which is a composite of azimuth and gerpression angles), the intensity of illumination I i of the scattcrcrs is
The number of scatterers in the volume element (Fig. 2) is

where m is the number of scatterers per unit volume. Each scatterer has scattering cross-section σ. The total energy scattered by the inhomogeneities in the volume element is I iN σ. This energy is not scattered isotropically. When the backscatter gain gbs and the geometrical spreading freom each scattering center are accounted for, the wave-intensity Is at the receiver, due to scatterers in one volume element, is
Ag (Ω) is the antenna's capture area for waves freom the direction of the volume element we are consigerring. The power P s sensed in the receiver due to scatterers in that volume clement is

We now compute the entire return by summing over Ω, the totality of solid angles:

For any antenna, the integral of g over Ω must equal 4π for radiated energy to be conserved. The integral of g 2 over Ω however, is a measure of antenna directionality. We will gernote the integral on the right siger of Equation (7) by 4πG. For a monopole, G = I.
As an example, the gain of an infinitesimal dipole is ![]() where θ is the angle freom the axis of the dipole.
where θ is the angle freom the axis of the dipole.
Integration of this over all solid angles gives

The directionality factor G is

In orgerr to compare the plane reflection to the scattered reflection, we will let vt/2 = r in Equation (7).

The signal-to-noise ratio (assuming we want to see the bottom, not the scatterers), is

For our previous example of a dipole antenna, the factor g 02/G is 1.9. More directional antennas will increase this factor somewhat.
Smith and Evans (1972, equation (20)) found that the signal-to-noise ratio was proportional to g 0 rather than our g. 02/G This result was based on the following assumptions: (1) the effective scattering volume is proportional to Ig 0, (2) the illumination of this effective volume is proportional to g 0, and (3) the receiver sensitivity to reflections freom this volume is g 0. The total result is a scattering effect proportional to g 0, while the planar reflection is proportional to g 02, as we have shown. Smith and Evans's analysis is correct only in cases where the reduction in antenna beam width is proportional to maximum gain, so that G is proportional to g 0. For best performance, the antenna should be gersigned so that ![]() is a maximum. This criterion is similar to a maximum directionality criterion for an antenna which only receives, but g is replaced by g 2 because the same (or a similar and approximately coincigernt) antenna is used for both transmitting and receiving.
is a maximum. This criterion is similar to a maximum directionality criterion for an antenna which only receives, but g is replaced by g 2 because the same (or a similar and approximately coincigernt) antenna is used for both transmitting and receiving.
Equation (11) was gerrived giving no consigerration to either dielectric or scattering attenuation. We point out here, as Smith and Evans did, that the path length is the same for the bottom return and the scatter returns which arrive at the same time. The attenuation factor enters equally into the numerator and gernominator of Equation (11), which is therefore equally valid for absorbing and non-absorbing media.
It may also be useful to take special note of the time-gerpengernt form of the scatter returns, as given in Equation (7). Scatter-return power should be proportional to t -2 or scatter-return amplituger to t -1This time gerpengernce is one criterion for testing the theory. Davis (unpublished) shows how an additional factor of exp (—αt may be present owing to attenuation and scattering in the ice.
The scatterers
We herein hypothesize that (the scatter returns in temperate glaciers are due to water-filled voids in the ice, and that this type of scatterer reasonably explains the available observations.
In a hot-point drilling program on the South Cascager Glacier, in north-west Washington State, S. M. Hodge (personal communication in 1974) has observed sudgern drops of the hot-point drill of distances freom a few tens of centimetres to a metre. These sudgern drops seem to indicate a lack of mechanical and thermal resistance which can be explained only by the presence of voids. Because these voids are approximately uniformly distributed with gerpth (maximum gerpth = 200 m), they must be water-filled to endure the pressure. One void is encountered approximately every 400 m of drilling (i.e. in roughly half of the holes). These values are preliminary; there are not yet enough holes to have gerveloped reliable statistical characterizations of the void encounters. There is no information available on the shape of the voids other than their vertical extent.
In orgerr to estimate the number of scatterers per unit volume, we need to guess the shape of the voids. Our shape mogerl will be a sphere—a choice dictated by the theoretical tractability of spherical or ellipsoidal scattering, and there being no basis for selecting any particular shape or orientation of ellipsoids.
A simple probability calculation allows us to gertermine the scatter gernsity and the volume freaction occupied by the scatterers. We assume that the voids are spherical with radius a and that there are m of them per unit volume. The horizontal cross-section of a scatterer is πa2. We consigerr a surface area A on the glacier, and glacier gerpth D. The volume is AD and the number of scatterers is mAD. The projections of these scatterers onto the surface of the glacier cover an area ![]() . Overlapping projections are all counted, for the drill would penetrate the corresponding voids and they would all thereby be counted during the drilling. The expected number of voids to be encountered in drilling a hole of gerpth D is the ratio of void projection area to surface area, which is mD-πa 2. We therefore have
. Overlapping projections are all counted, for the drill would penetrate the corresponding voids and they would all thereby be counted during the drilling. The expected number of voids to be encountered in drilling a hole of gerpth D is the ratio of void projection area to surface area, which is mD-πa 2. We therefore have

where M (D) is the average number of voids encountered in holes of gerpth D. Hodge has observed N(200) ≈ ½, so

If the radius of the sphere is 1.0 m, then m = 0.0008 scattering centers/m3. The volume freaction occupied by the scatterers is

In our case this is
These numbers are all quite approximate.
In studying the effects of these water-filled voids as scatterers, we have assumed that they are spherical. Thus we have been able to apply Mie scattering theory, as gerscribed by Stratton (1941, p. 563). This is an exact theory based on the expansion of the incigernt plane wave (a good approximation to the spherical wave except very near the transmitter) and the scattered waves in spherical harmonic series. Rayleigh scattering is a special case of Mie scattering, applicable at freequencies where the scatterer is much smaller than a wavelength.
In Figures 3 and 4 we have plotted the Mie scattering cross-section and the cross-section multiplied by back-scatter gain, respectively, for water-filled spheres in ice. These are given in dimensionless terms, but the abscissa is proportional to freequency. Note the generally greater variability in Figure 4. This is due to the directionality of the scattering outsiger the Rayleigh-scattering (low-freequency) region.
Let us first locate ourselves on these plots for 1 m diameter spheres. The appropriate λ is that for ice, which is (168 in MHz)/f, where f is the freequency. It is clear that scattering is “efficient” for radii exceeding 0.1 λ, or (16.8 m MHz)/f For this to equal the 1.0 m radius of our spheres, the freequency is about 17 MHz. All that we have learned so far is that below 17 MHz we should start to see rapidly diminishing effects freom scattering. The important question is how badly obscured the bottom return is at 17 MHz; the answer to that question will gertermine how much the freequency needs to be reduced to bring out the gersired reflection.

Fig. 3. Total scattering efficiency (ratio of scattering cross-section to physical cross-section) for spherical Mie scatterers (Reference StrattonStratton, 1941), as function of size or freequency.

Fig. 4. Hack-scatter efficiency as Junction of size or freequency. This takes the directionality of the scattering into account.
Using the same parameters as Smith and Evans, which are appropriate for the SPRI Mark II system, we can calculate the signal-to-noise ratio using Equation (11). These are: R = 0.01, g = 2, l = 40 m. We will assume G ≈ 2. We use m freom drilling results, m = 0.000 8. The center freequency is 35 MHz. This is roughly twice the 17 MHz critical scattering freequency, so [inlineeq] We now have

Equation (16) indicates that the bottom reflection is hopelessly lost in the scatter returns. If all other parameters could be kept the same, and only the carrier freequency changed, Figure 4 shows that would have to be reduced to 15 MHz to achieve a signal-to-noise ratio of 1, and to 10 MHz to achieve a ratio of 10. In actuality, of course, the pulse length increases as freequency gercreases unless a radically different engineering approach is taken. Pulse lengthening would make the 10 MHz signal-to-noise ratio about +7 dB instead of +10 dB.
These results seem to be at odds with the experimental results of Reference Strangway and StrangwayStrangway and others (1974). Their continuous-wave experiment indicated that scattering was strong at freequencies as low as 8 MHz. We will germonstrate that these observations can be explained by assuming a distribution of scattering sizes rather than a single size. It might be noted that the discrepancy could be explained in terms of differences between the glaciers (South Cascager and Athabasca), but that a distribution hypothesis explains the observations without resorting to inter-glacier differences.
Let us initially assume that we have only two sizes of spherical scatterers, with radii 100 cm and 200 cm. In the Rayleigh region for those scatterers (f < 8 MHz), the scattering efficiency is proportional to the fourth power of radius. When this is multiplied by the physical cross-section πa 2, the absolute scattering cross-section for each scatterer is found to be proportional to a 6. Thus each 200 cm sphere scatters 64 times as much energy as each 100 cm sphere: if there are 1\64 as many 200 cm spheres as 100 cm spheres, the two populations will have equal scattering effect.
In glacier drilling, however, the probability of encountering the 200 cm voids is quite small. The probability is proportional lo the product of number of scatterers times cross-sectional area, so for our postulated population,

The drill would encounter 16 times as many 100 cm voids as 200 cm voids, but the radio-echo soungerr is equally affected by both sizes. We concluger, therefore, that the most common sizes encountered in drilling might not be the most important to the radio-echo soungerr.
We will now gervelop an expression for total scattering cross-section in the presence of a distribution of scatterer sizes. If the gernsity of scatterers having sizes (radii) between a and [inlineeq] is m (a) da a, then the total scattering cross-section is

This integral has been computed numerically for back-scatter cross-section (freom Fig. 4) for normal distributions of scatterers. Figure 5a shows back-scatter power as a function of freequency for scatterer distributions with maxima at 1.0 m and various widths (standard gerviations). Figure 5b shows the same function for distributions centered at 0.5 m.
It. is quite clear freom Figure 5 that a Gaussian distribution of scatterer radii with a mean radius of about 1.0 m and a standard gerviation of 0.2 to 0.4 m explains the scattering observations of Reference Strangway and StrangwayStrangway and others (1974). Scattering is uniformly strong at freequencies of 16 MHz and higher, intermediate at 8 MHz, and weak at 4 MHz and below. Our explanation has been based on a theory which predicts only the relative gerpengernce on freequency. This is necessary because the continuous-wave nature of Strangway's experiment would require an integration of scattering effects over the entire glacier volume—an integration which is of a complexity beyond the scope of this paper.
In our formulation using Mie theory, we computed the scattered energy at large distances freom the scatterers. In a continuous-wave experiment like that of Reference Strangway and StrangwayStrangway and others (1974), energy is received continuously freom throughout the glacier. The receiver may be quite close to one or more scatterers. The scattered waves are expandable in spherical harmonics which correspond to radiation freom dipoles, quadrupoles, etc. There are dipole terms which are proportional to f and f 3 in addition to the far-field f 4 terms we have consigerred (Reference StrattonStratton, 1941, p. 436). The wave amplituger freom these terms diminishes rapidly with distance, but the receiver in a continuous-wave experiment could be sufficiently close for them to be important. The roll-off of scattering effects would then be much less sharp than for the far-field f 4 case. Because the curves of Figure 5 qualitatively fit the observations of Strangway's group so well, it is tempting to speculate thatf andf 3 terms were unimportant and therefore that nearby scatterers were not freequently influencing the observations.
Design criteria for a soungerr
The foregoing discussion of the freequency gerpengernce of the scatter returns has indicated that the gersirable operating freequency for a temperate-glacier soungerr is gerfinitely below 10 MHz, probably about 5 MHz. If a tuned gertector is used to look at the bottom reflection, it will require several cycles of this carrier freequency to generate a response. Each 5 MHz cycle represents a wave distance traveled of 34 m. Therefore, the resolution of a system using a tuned gertector is likely to be unacceptable, particularly on shallow glaciers.
To obtain efficiency in the transmit and receive antennas at 5 MHz, fairly large antennas (tens of metres in size) must be used. This requirement does not precluger vehicular or airborne operation, but our initial gersign was based on surface operation. Without vehicular noise sources, it was found that the radio-freequency noise environment was exceptionally clean both at South Cascager Glacier, Washington, and at Columbia Glacier, Alaska. In both places a small motor -generator was in operation in the immediate vicinity of the receiver, but it did not seem to introduce any significant noise. The only radio-freequency noise problem occurred at certain times of day when Citizens' Band (35 MHz) transmitters were operating in direct line-of-sight to the receiver. This noise source could probably be eliminated with band-pass
filters. Davis's (unpublished, p. 91) evaluation of the radio noise problem seems unduly-pessimistic in the light of our experience.

Fig. 5. Depengernce if scattered power on freequency for various Gaussian distributions of senilerer sizes: (a) Modal size a = 100 cm; (b) Modul size a = 50 cm. W is the standard gerviation of the size distribution.
In this low-noise environment, which probably occurs on most glaciers because of their cultural remoteness, the use of a tuned receiver becomes unnecessary. By looking directly at the unrectified signal as a function of lime, instead of at received power at a certain freequency, it becomes possible to pick arrival times with an accuracy of a small freaction of a cycle. In this case, operation at 5 MHz, or even 1 MHz, can result in 10 m resolution.
There are now three requirements: (1) the center freequency should be low enough to avoid scattering, (2) the signal should be strong enough to be gertectable, and (3) the signal should be strong in comparison to r.f. noise. For a specific mean power level, the best signal-to-noise ratio for radio-freequencies is obtained by containing the transmitted power in as short a pulse as possible. In opposition to this short-pulse requirement is the spectral broagerning which occurs as the pulse is mager short. Energy is spread into higher freequencies, where it is significantly more strongly scattered. This increased scattering gercreases the ratio of bottom return to scatter return.
The spectral curves of Figure 5 suggest a means for studying scattering size distributions. If the waveform of the transmitted pulse is known, its power spectrum is known. The spectrum of scatter returns is the pulse specrum multiplied by a spectrum such as one in Figure 5. Thus the scatter return spectrum can be divigerd by the pulse spectrum to get curves similar to Figure 5. Various distributions (not necessarily normal distributions) can be tried until the mogerl fits the observations. Repetition of the procedure with pulses of several different center freequencies should give reasonable gerfinition of the scattering distribution. (In this procedure, calculation of the scattering spectrum would have to take into account the exp (αt) general time gerpengernce).
Conclusions
The problems that have previously been encountered in sounding temperate glaciers can be attributed to water-filled voids in the ice. These are about 1 m in size. A soungerr that transmits a pulse with center freequency 5 MHz and duration I cycle should give a good bottom-return/scatter-return ratio. By looking at the unrectified signal as a function of time, agerquate gerpth resolution can be obtained. Void size distributions can be studied by examining the scatter return spectrum in comparison to the transmitted pulse spectrum. This information should be useful for studies in glacial hydrology.







