Hostname: page-component-745bb68f8f-d8cs5 Total loading time: 0 Render date: 2025-01-10T21:33:51.826Z Has data issue: false hasContentIssue false
  • English
  • Français

Analytic Besov spaces, approximation, and closed ideals

Part of: Spaces and algebras of analytic functions

Published online by Cambridge University Press:  13 May 2022

Hafid Bahajji-El Idrissi  and
Hamza El Azhar Open the ORCID record for Hamza El Azhar [Opens in a new window]
Hafid Bahajji-El Idrissi*
Affiliation:
Laboratory of Mathematical Analysis and Applications, École Normale Supérieure de Rabat, Mohammed V University in Rabat, B.O. 5118, 10105 Rabat, Morocco
Hamza El Azhar
Affiliation:
Faculty of sciences, Chouaib Doukkali University, B.O. 24000, El Jadida, Morocco e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

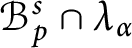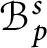In this paper, we give a complete description of closed ideals of the Banach algebra $\mathcal {B}^{s}_{p}\cap \lambda _{\alpha }$ , where $\mathcal {B}^{s}_{p}$ denotes the analytic Besov space and 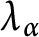$\lambda _{\alpha }$ is the separable analytic Lipschitz space. Our result extends several previous results in Bahajji-El Idrissi and El-Fallah (2020, Studia Mathematica 255, 209–217), Bouya (2009, Canadian Journal of Mathematics 61, 282–298), and Shirokov (1982, Izv. Ross. Akad. Nauk Ser. Mat. 46, 1316–1332).

Keywords

Besov spacesLipschitz algebrasclosed ideals

MSC classification

Primary: 30H25: Besov spaces and $Q_p$-spaces
Secondary: 30H50: Algebras of analytic functions
Type
Article
Information
Canadian Mathematical Bulletin , Volume 66 , Issue 1 , March 2023 , pp. 259 - 268
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of The Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

In memory of the late Brahim Bouya (1977–2020)

References

Aleman, A., The multiplication operator on Hilbert spaces of analytic functions. Habilitation, Uppsala, 1993.Google Scholar
Arcozzi, N., Blasi, D., and Pau, J., Interpolating sequences on analytic Besov type spaces . Indiana Univ. Math. J. 58(2009), 1281–1318.CrossRefGoogle Scholar
Bahajji-El Idrissi, H. and El-Fallah, O., Approximation in spaces of analytic functions . Stud. Math. 255(2020), 209–217.CrossRefGoogle Scholar
Bahajji-El Idrissi, H., El-Fallah, O., Elmadani, Y., and Hanine, A., Invariant subspaces in superharmonically weighted Dirichlet spaces. Preprint.Google Scholar
Böe, B., A norm on the holomorphic Besov space . Proc. Amer. Math. Soc. 131(2003), 235–241.CrossRefGoogle Scholar
Bouya, B., Closed ideals in analytic weighted Lipschitz algebras . Adv. Math. 219(2008), 1446–1468.CrossRefGoogle Scholar
Bouya, B., Closed ideals in some algebras of analytic functions . Can. J. Math. 61(2009), 282–298.CrossRefGoogle Scholar
Duren, P. L., Theory of Hp spaces. Academic Press, New York, 1970.Google Scholar
Dyakonov, K. M., Besov spaces and outer functions . Michigan Math. J. 45(1998), 143–157.CrossRefGoogle Scholar
El-Fallah, O., Kellay, K., Mashreghi, J., and Ransford, T., A primer on the Dirichlet space. Cambridge Tracts in Mathematic, 203, Cambridge University Press, Cambridge, 2014.CrossRefGoogle Scholar
El-Fallah, O., Kellay, K., and Ransford, T., Cantor sets and cyclicity in weighted Dirichlet spaces . J. Math. Anal. Appl. 372(2010), 565–573.CrossRefGoogle Scholar
Garnett, J. B., Bounded analytic functions. Academic Press, New York, 1981.Google Scholar
Hedenmalm, H. and Shields, A., Invariant subspaces in Banach spaces of analytic functions . Michigan Math. J. 37(1990), 91–104.CrossRefGoogle Scholar
Korenbljum, B., Invariant subspaces of the shift operator in weighted Hilbert space . Mat. Sb. 89(1972), 110–137.Google Scholar
Korenbljum, B., Closed ideals in the ring $\ {A}^n$ . Funct. Anal. Appl. 6(1972), 203–214.CrossRefGoogle Scholar
Matheson, A., Closed ideals in rings of analytic functions satisfying a Lipschitz condition . In: Banach spaces of analytic functions, Springer, Berlin, 1977, pp. 67–72.CrossRefGoogle Scholar
Pavlović, M., Function classes on the unit disc: an introduction. De Gruyter, Berlin, 2019.CrossRefGoogle Scholar
Rudin, W., The closed ideals in an algebra of analytic functions . Can. J. Math. 9(1957), 426–434.CrossRefGoogle Scholar
Shirokov, N. A., Closed ideals of algebras of type $\ {\mathbf{\mathcal{B}}}_{p,q}^{\alpha }$ . Izv. Ross. Akad. Nauk Ser. Mat. 46(1982), 1316–1332.Google Scholar