General remarks

The basic physics of remote sensing of snow and ice in the TM bands is well established. The spectral reflectivity of snow depends on grain-size and shape, near-surface liquid-water content, surface roughness, impurities, and the angle of solar incidence. Various models have been developed for calculating the snow albedo (e.g. Reference Smith and ColwellSmith 1983). Reflectivity in snow generally decreases with increasing wavelength in the visual and “near” infra-red, and variations with wavelength in the albedo of snow, firn, and ice are broadly parallel (Reference Qunzhu, Meisheng, Xuezhi, Fengxian, Xianzhang and WenkunQunzhu and others 1984). Snow albedo decreases with increasing grain-size at wavelengths above 0.7 μm, but it varies little in the wavelengths below this value (Reference Choudhury and ChangChoudhury and Chang 1981). Thus grain-size variations do not show in TM bands 1, 2, and 3. By contrast, TM bands 5 and 7 discriminate well between snow, ice clouds, and water clouds; in these bands, albedoes of the three features increase in the order given (Reference DozierDozier 1985).

Surface temperatures

TM band 6 records radiation at about 11 μm. This band lies in an atmospheric window that is transparent to water vapour. Terrestrial black-body radiation at temperatures in the range −25° to 0°C reaches its maximum near this wavelength.

Reference Markham and BarkerMarkham and Barker (1986) provided data for calculating ground temperatures from the digital numbers recorded at the satellite. The spectral radiance, Lλ, is given in mW cm⁻² ster⁻¹ μm⁻¹ from the following equation:

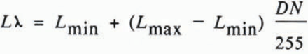
where L _min and L _max are calibration constants given as 0.1238 and 1.5600 mW cm⁻² ster⁻¹ μm⁻¹ respectively, and DN is the observed digital number for each pixel, ranging from 0 to 255. These constants apply to data acquired after 15 January 1984.

The effective brightness temperature T, in K, of the viewed Earth-atmosphere system can then be calculated from Lλ according to the following equation:

Markham and Barker (1986) gave K₁ = 60.776 mW cm ⁻² ster⁻¹ μm^–1, and K₂ = 1260.56 K. They also stated that these numbers are the pre-launch calibration constants.

Figures 2a, 3a, and 4a show the computed brightness temperatures of quads 1, 2, and 4 recorded at the satellite at 07.52 GMT on 8 February 1985. Generally the brightness temperatures decrease with increasing elevation, and the effects of material reflectivity and the incidence angle of the Sun are superimposed on the elevation effects, so that the highest temperatures occur on surfaces of low albedo facing the Sun. Orheim and Lucchitta (1987) discussed some of these effects, which had been observed in the TM6 data. Here we explore whether the brightness temperatures correspond to ground observations.

We were able to reconstruct the ground-temperature distribution and to compare it with the satellite-measured temperatures because the Norwegian Antarctic Research Expedition 1984–85 operated a meteorological station on a snow-field (71°53’S, 5°10’E; 1600 ma.s.l.) and observed temperatures from 17 January to 14 February 1985 (Fig. 5a), both at the station and at many other locations (personal communication from T. Eiken). The station was about 10 km east of the image area of quad 2 (Fig. 3a), close enough to give representative values. Weather observations at 08.00 GMT on 8 February 1985 included the following: (at 2 m above the surface) air temperature: −12°C, and wind: 13 ms⁻¹ from south; clouds: 0; precipitation: 0; and visibility: >50 km.

Mobile field parties measured air temperatures 2 m above the surface of the snow-field, at seven sites within quad 2 (Fig. 3b), from 19 January to 9 February 1985, typically over a 3d period (personal communication from T. Eiken). The sites range from 1380 to 2680 m in elevation. Temperature observations were also made at six sites, ranging in elevation from 50 to 2700 m, outside the area shown in this scene.

Air temperatures were linearly related to elevation. Observed temperature gradients for six sites, differing by >400 m in elevation from that of the meteorological station, ranged from 0.5°C/100m to l.l°C/100m. The mean gradient was 0.7°C/100m, and the gradients of the sites with the largest difference in elevation from that of the meteorological station were closest to this value. Using this gradient, we extrapolated the observations in order to construct maps of the air temperature 2 m above the surface for quads 1, 2, and 4 at 08.00 GMT for 8 February 1985 (Figs 2b, 3b, 4b). Comparison of extrapolated temperatures with measured temperatures indicates that the maps reproduce air temperatures over the large featureless snow areas to ±3°C.

One further set of ground temperatures is available. Water bodies, including 14 lakes as large as 3km² in surface area, were observed in several places at about 100 m elevation in quad 1 (Orheim and Lucchitta 1987). Each lake had an albedo of about 0 in the six reflective bands. Their temperatures must have been 0°C or slightly lower if the water surface was covered by an ice layer (which was probably thin, because it was visually transparent). Pixel DN values for one of these lakes are given in Figure 6.

Observations at an ice-free site (Fig. 5b) demonstrate the more complex temperature variations over rock. The site was near the meteorological station. Its temperatures as recorded at the time of the satellite pass were −2.5°C below the gravel surface (under rock), −5.5°C at the surface (at the base of vegetation), and −6°C in the air 15 cm above the surface. These values clearly show the influence of the positive-radiation balance for low-albedo surfaces facing the Sun. On the other hand, on this clear day, areas in shadow had much lower surface temperatures (Fig. 3a). The present comparison of image and ground temperatures is restricted to areas of low relief where ground temperatures are homogeneous over large extents, making it easy to match pixel and ground data. We do not have sufficient field data to attempt to reproduce the greatly varying ground temperatures within the mountains and over blue-ice fields.

Fig. 3a. Quad 2 (upper right quadrant) of scene 5034407520.

(a) Colour-coded brightness temperatures, determined from TM-band 6 data. The areas in shadow behind the mountains are the coldest (dark blue and purple). The warm areas in the centre of the image are bare rock outcrops of Jutulsessen (largely white), lakes (red), and various blue-ice fields (orange). The latter are formed on the lee (west) side of nunataks and rock ridges and other topographic forms that interrupt drifting snow; the blue-ice fields align with the prevailing wind direction. Note also the cold areas in the snow-fields below the mountains, probably caused by cold air from the inland ice sheet flowing down along the ice streams and glaciers. The picture is 90 km wide, north toward upper left.

Fig. 3b. (b) Contour map, showing air temperatures in °C 2 m above the snow surface (heavy solid lines), and elevation contours (dotted lines). Locations of air-temperature observations are shown by x.

The satellite recorded the exo-atmospheric radiation from the ground. To correlate its measurements with those on the ground, we must consider two principal variables: (1) the relation between the measured air temperature and brightness temperatures, and (2) the amount of atmospheric absorption.

Scene 5034407520 was collected under cloud-free conditions, without detectable haze in the atmosphere, with a solar elevation angle of 22.1°, and during rising temperatures that indicate a slightly positive radiation balance for level snow surfaces. Strong winds at the time of image acquisition (13 ms⁻¹, the strongest recorded by the expedition) imply an efficient heat exchange between the snow surface and the air. Data for similar conditions (e.g.Reference Vinje Vinje 1975) show snow-surface and air temperatures to correspond within 1–2°C. Therefore we assume that our measured snow and air temperatures were equal. Brightness temperatures are the product of the ground temperature and emissivity. At TM-band 6 wavelengths, the surface approximates a black body, and snow emissivity probably deviates little from unity. That the emissivity at the thermal band is constant is supported by observations that show marked patterns on the snow surface in the infra-red reflective bands 5 and 7 (see below). These patterns probably reflect different snow-surface properties, yet the patterns are not visible on thermal band 6 in the same area.

Fig. 5a. Fig. 5a. Mean daily air temperature at snow-field at 1600 m elevation, 17 January —14 February 1985. See text for the location of the measurements.

Fig. 5b. Fig. 5b. Ground and air temperatures recorded above rock near the same snow-field, 8–11 February 1985 (fromReference Somme S⌀mme 1986).

The recorded brightness temperature depends also on atmospheric absorption. The Landsat scene investigated contains a small group of what are probably alto-cumulus clouds (Orheim and Lucchitta 1987); the effect of these clouds can be seen as a brightness-temperature reduction of about 2°C. We assume that few other large atmospheric absorption effects existed, in particular because the low temperatures permit only a low water-vapour content. Atmospheric absorption over Antarctica should be less than that over more temperate latitudes, so that any constant calibration compensating for atmospheric absorption should, at this location, give rise to errors on the warm side.

Bearing in mind the potential errors discussed above, we conclude that the temperature maps (Figs 2b, 3b, and 4b) should reproduce the real near-surface temperatures of level snow to ±4°C. However, the comparisons of satellite brightness temperatures with measured surface temperatures (Figs 2a, 3a, and 4a) show that the brightness temperatures for the coldest snow areas are about 20°C too low, whereas the temperatures for the water bodies appear to be 8°C too low. These deviations are too large to be physically plausible. In general, the changes in brightness reflect those in the near-ground temperatures, but the brightness temperatures are too low and have a gradient that is too high.Table II is a summary of the results for the quads investigated. The DN values in the table exclude those representing less than 0.1% of the total number of pixels (see also Fig. 7), in order to eliminate spurious information. However, this also excludes the values for the small water bodies in quad 1, which have DN values as high as 72 (Fig. 6).

Fig. 6. Fig. 6. Pixel DN values for a lake of ≈2 km diameter at the edge of Jutulstraumen in quad 1, scene 5034407520. DN values of 67–72 in the larger circled area represent water which is possibly covered by a very thin layer of ice. Lower DN values in the central part of the lake (the smaller circled area) result from a cover of snow and ice. Such partial cover was commonly observed on the lakes.

These results expand the temperature range of the Landsat-5 TM-band 6 radiometric calibration undertaken in June 1984 by Reference Schott and VolchokSchott and Volchok (1985); their range comprised surface temperatures between approximately 12° and 35°C. They found that satellite-predicted and ground temperatures agreed at about 22°C, but that the sensor had a systematic gain error of 1.43, causing the higher temperatures to be given as increasingly too warm. They suggested that this error was produced by moisture accumulation in the instrument. Markham and Barker (1986) also noted that the post-launch calibration constants may differ from the pre-launch constants used in (equ.2). Our results indicate that the instrument has a gain of 1.63, i.e. about 20% higher than that given by Schott and Volchok (1985).

Fig. 4a. Quad 4 (lower right quadrant) of scene 5034407520 (a) Colour-coded brightness temperatures, determined from TM-band 6 data. The coldest areas (dark blue and purple) show BTs of −40°C. Note that the surface-snow patterns seen in TM bands 5 and 7 (fig. 8 in Orheim and Lucchitta 1987) and reflected in PC2 (Fig. 10) do not show in the BTs. Note also the small-scale variations in brightness temperatures of the snow surface above 2300 m elevation (Fig. 4b). The picture is 90 km wide, north toward upper left.

Fig. 4b. (b) Contour map, showing air temperatures in °C 2 m above the snow surface (heavy lines, dashed where located approximately), and elevation contours (dotted lines).

Fig. 7. Fig. 7. TM-band 6 DN frequencies for quads 1, 2, and 4 of scene 5034407520. Note different vertical scales. The differences in DN frequency distribution demonstrate the differences in elevation and radiance between the quads. Quad 1 contains the lowest and warmest areas of the scene, including water bodies and blue-ice fields at low elevations. Quad 2 covers the largest elevation intervals (Table II), and also includes most of the nunataks within the scene. These show a large range of £Ws, depending upon inclination to the Sun. Quad 4 is the most homogeneous and covers mostly high-elevation snow areas with low DN values.

A new temperature-contour map is shown in Figure 8. For its preparation we used our ground-temperature end members for the imaged area (0°C for lakes and −20°C for the coldest areas) and apportioned the radiance measured by TM band 6 to this temperature range. This map portrays the relative temperature variations as sensed by the satellite instrument, but it has temperature values that more realistically reflect the actual temperatures on the ground.

Table III gives pixel DN values and the corresponding revised brightness temperatures shown in Figure 8. We recommend additional ground-truth testing to clarify whether this Table can generally be used for estimating <0°C surface temperatures from DNs measured by Landsat-5.

Fig. 8. Fig. 8. Entire scene of 5034407520, showing temperature contours at 2°C intervals. The temperatures are calculated from TM-band 6 radiance values so as to reflect known temperature end members on the ground. Temperatures range from 0°C (white) to −23°C (dark blue), as shown by the temperature scale. The picture is 180 km wide, north toward upper left.

We conclude that the TM-band 6 data faithfully reproduced the relative temperature variations within a scene but had large errors in absolute temperatures. The brightness-temperature maps also show variations in surface temperature which we cannot test by ground truth, but Which we believe are real. The most important of these are probably related to variations in inclination and reflectivity, and were described previously. We also note the effects of cold air flowing down the ice streams (e.g. Figs 3a and 8) and detailed variations in snow temperatures over the inland plateau (Figs 4a and 8), which must have other causes.

Principal component technique

PCs are generally superior to individual bands or combinations of bands for displaying certain characteristics of a scene. Table IV gives the eigenvalue matrix and per cent variance of each PC of quad 4. The table shows that most of the information from the scene is contained in PCs 1–3. PCs 4 and 5 contain mostly noise and systematic image defects such as striping.

Short-term changes in surface signatures

In Figure 12, a multispectral composite of TM bands 4, 5, and 7 of scene 5034407520 (collected on 8 February 1985) can be compared with a multispectral composite of the same bands of scene 5036207394 (collected on 26 February 1985) in their area of overlap (Fig. 1). The strong patterns on the surface, shown in PC2 and reflecting largely data from TM bands 5 and 7 (Fig. 10) of the earlier image, have undergone large changes, and they have faded almost completely in the image taken 18 d later. The edges of the former patterns are still faintly visible, but the differences in reflectivity have disappeared, primarily because the low-albedo areas have lightened. Some of these areas have become even lighter than the surrounding areas, so that the signatures are reversed in the later image.

The increased albedo could be caused by a decrease in surface grain-size, most probably caused by deposition of new snow on only the low-albedo areas. (Without deposition of new snow, the grain-size of the surface will normally increase during the summer.) However, the patterns do not appear to be related to the topography, so that topographic trapping of drifting snow seems unlikely. Such differential deposition could instead be caused by variations in surface roughness, i.e. deposition on non-glazed surfaces only.

Fig. 11. Fig. 11. Multispectral colour composite of TM-band ratios 4/5, 2/5, and 2/4 of quad 1 of scene 5034407520. Note the dendritic pattern running down-slope to Jutulstraumen, in the left centre of the image (arrow), and the lakes (yellow) in the same area. High-albedo snow shows as blue; different types of ice range from pale yellow to orange. The picture area is 90 km wide, north toward upper left.

An alternative explanation is that the features in the first image were ephemeral effects caused by the high winds on 8 February, and perhaps were related to various physical properties associated with newly drifted snow. We do not believe that the phenomena were caused by low-level clouds or thin fog. Comparison with the blue-ice areas shows that there is no difference in the images, which would be expected if such weather conditions existed across the scene. Clearly, more ground information is needed to resolve these problems.