Hostname: page-component-669899f699-ggqkh Total loading time: 0 Render date: 2025-04-29T06:15:50.244Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of horizontal magnetic fields on flow morphologies and global transports in liquid metal thermal convection

Published online by Cambridge University Press:  27 November 2024

Xin-Yuan Chen Open the ORCID record for Xin-Yuan Chen [Opens in a new window] ,
Juan-Cheng Yang Open the ORCID record for Juan-Cheng Yang [Opens in a new window]  and
Ming-Jiu Ni Open the ORCID record for Ming-Jiu Ni [Opens in a new window]
Xin-Yuan Chen
Affiliation:
State Key Laboratory for Strength and Vibration of Mechanical Structures, School of Aerospace, Xi'an Jiaotong University, Xi'an 710049, PR China
Juan-Cheng Yang*
Affiliation:
State Key Laboratory for Strength and Vibration of Mechanical Structures, School of Aerospace, Xi'an Jiaotong University, Xi'an 710049, PR China
Ming-Jiu Ni*
Affiliation:
State Key Laboratory for Strength and Vibration of Mechanical Structures, School of Aerospace, Xi'an Jiaotong University, Xi'an 710049, PR China School of Engineering Science, University of Chinese Academy of Sciences, Beijing 100049, PR China
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

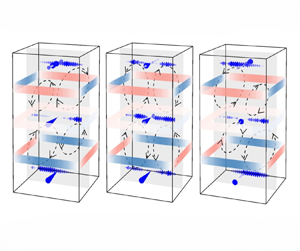
We report an experimental study of Rayleigh–Bénard convection of liquid metal GaInSn in a cuboid cell with an aspect ratio of 0.5 under the effect of a horizontal magnetic field. The Rayleigh number spans a range of $3.8\times 10^5 \leqslant Ra \leqslant 1.1\times 10^7$, while the magnetic field strength reaches up to 0.5 T, corresponding to a maximum Hartmann number to 2041. By combining temperature and velocity measurements, we identify several flow morphologies, including a novel cellular pattern characterized by four stacked vortices that periodically squeeze and induce velocity reversals. Based on the identified flow morphologies, we partition the entire ($Ra, Ha$) parameter space into five distinct flow regimes and systematically investigate the flow characteristics within each regime. The temperature gradient and oscillation frequency exhibit scaling relationships with the combined parameters $Ra$ and $Ha$. Notably, we observe a coupling between flow regime and global transport efficiencies, particularly in a regime dominated by the double-roll structure, which experiences a maximum 36 % decrease in heat transfer efficiency compared with the single-roll structure. The dependencies of heat and momentum transport on $Ra$ and $Ha$ follow scaling laws as $Nu \sim (Ha^{-2/3}RaPr^{-1})^{3/5}$ and $Re \sim (Ha^{-1}RaPr^{-1})^{4/3}$, respectively.

JFM classification

MHD and Electrohydrodynamics: Magneto convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 1000 , 10 December 2024 , A59
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Ahlers, G., et al. 2022 Aspect ratio dependence of heat transfer in a cylindrical Rayleigh–Bénard cell. Phys. Rev. Lett. 128 (8), 084501.CrossRefGoogle Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.CrossRefGoogle Scholar
Akhmedagaev, R., Zikanov, O., Krasnov, D. & Schumacher, J. 2020 Turbulent Rayleigh–Bénard convection in a strong vertical magnetic field. J. Fluid Mech. 895, R4.CrossRefGoogle Scholar
Aref, H. & Zawadzki, I. 1991 Linking of vortex rings. Nature 354 (6348), 50–53.CrossRefGoogle Scholar
Aurnou, J.M. & King, E.M. 2017 The cross-over to magnetostrophic convection in planetary dynamo systems. Proc. R. Soc. Lond. A 473 (2199), 20160731.Google ScholarPubMed
Bader, S.H. & Zhu, X. 2023 Scaling relations in quasi-static magnetoconvection with a strong vertical magnetic field. J. Fluid Mech. 976, A4.CrossRefGoogle Scholar
Barleon, L., Casal, V. & Lenhart, L. 1991 MHD flow in liquid-metal-cooled blankets. Fusion Engng Des. 14 (3–4), 401–412.CrossRefGoogle Scholar
Brown, E. & Ahlers, G. 2007 Temperature gradients, and search for non-Boussinesq effects, in the interior of turbulent Rayleigh–Bénard convection. Europhys. Lett. 80 (1), 14001.CrossRefGoogle Scholar
Brown, E. & Ahlers, G. 2009 The origin of oscillations of the large-scale circulation of turbulent Rayleigh–Bénard convection. J. Fluid Mech. 638, 383–400.CrossRefGoogle Scholar
Burr, U. & Müller, U. 2001 Rayleigh–Bénard convection in liquid metal layers under the influence of a vertical magnetic field. Phys. Fluids 13 (11), 3247–3257.CrossRefGoogle Scholar
Burr, U. & Müller, U. 2002 Rayleigh–Bénard convection in liquid metal layers under the influence of a horizontal magnetic field. J. Fluid Mech. 453, 345–369.CrossRefGoogle Scholar
Busse, F.H. 2008 Asymptotic theory of wall-attached convection in a horizontal fluid layer with a vertical magnetic field. Phys. Fluids 20 (2), 024102.CrossRefGoogle Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Courier Corporation.Google Scholar
Chen, L., Wang, Z.-B. & Ni, M.-J. 2024 a Effects of horizontal magnetic fields on turbulent Rayleigh–Bénard convection in a cuboid vessel with aspect ratio $\varGamma = 5$. J. Fluid Mech. 980, A24.CrossRefGoogle Scholar
Chen, X., Huang, S.-D., Xia, K.-Q. & Xi, H.-D. 2019 Emergence of substructures inside the large-scale circulation induces transition in flow reversals in turbulent thermal convection. J. Fluid Mech. 877, R1.CrossRefGoogle Scholar
Chen, X., Wang, D.-P. & Xi, H.-D. 2020 Reduced flow reversals in turbulent convection in the absence of corner vortices. J. Fluid Mech. 891, R5.CrossRefGoogle Scholar
Chen, X.-Y., Xie, Y.-C., Yang, J.-C. & Ni, M.-J. 2023 Strong coupling of flow structure and heat transport in liquid metal thermal convection. J. Fluid Mech. 975, A21.CrossRefGoogle Scholar
Chen, X.-Y., Yang, J.-C. & Ni, M.-J. 2024 b Flow mode and global transport of liquid metal thermal convection in a cavity with $\varGamma = 1/3$. Phys. Rev. Fluids 9 (2), 023503.CrossRefGoogle Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh-Bénard convection. Eur. Phys. J. E 35, 1–25.CrossRefGoogle ScholarPubMed
Cioni, S., Chaumat, S. & Sommeria, J. 2000 Effect of a vertical magnetic field on turbulent Rayleigh–Bénard convection. Phys. Rev. E 62 (4), R4520.CrossRefGoogle ScholarPubMed
Cioni, S., Ciliberto, S. & Sommeria, J. 1997 Strongly turbulent Rayleigh–Bénard convection in mercury: comparison with results at moderate Prandtl number. J. Fluid Mech. 335, 111–140.CrossRefGoogle Scholar
Davidson, P.A. 1999 Magnetohydrodynamics in materials processing. Annu. Rev. Fluid Mech. 31 (1), 273–300.CrossRefGoogle Scholar
Davidson, P.A. 2017 Introduction to Magnetohydrodynamics. Cambridge University Press.Google Scholar
Ecke, R.E. & Shishkina, O. 2023 Turbulent rotating Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 55, 603–638.CrossRefGoogle Scholar
Fauve, S., Laroche, C. & Libchaber, A. 1981 Effect of a horizontal magnetic field on convective instabilities in mercury. J. Phys. Lett. 42 (21), 455–457.CrossRefGoogle Scholar
Grannan, A.M., Cheng, J.S., Aggarwal, A., Hawkins, E.K., Xu, Y., Horn, S., Sánchez-Álvarez, J. & Aurnou, J.M. 2022 Experimental pub crawl from Rayleigh–Bénard to magnetostrophic convection. J. Fluid Mech. 939, R1.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.CrossRefGoogle Scholar
Houchens, B.C., Witkowski, L.M. & Walker, J.S. 2002 Rayleigh–Bénard instability in a vertical cylinder with a vertical magnetic field. J. Fluid Mech. 469, 189–207.CrossRefGoogle Scholar
Ihli, T., Basu, T.K., Giancarli, L.M., Konishi, S., Malang, S., Najmabadi, F., Nishio, S., Raffray, A.R., Rao, C.V.S., Sagara, A., et al. 2008 Review of blanket designs for advanced fusion reactors. Fusion Engng Des. 83 (7–9), 912–919.CrossRefGoogle Scholar
Ji, D. & Brown, E. 2020 Low-dimensional model of the large-scale circulation of turbulent Rayleigh–Bénard convection in a cubic container. Phys. Rev. Fluids 5 (6), 064606.CrossRefGoogle Scholar
Jones, C.A. 2011 Planetary magnetic fields and fluid dynamos. Annu. Rev. Fluid Mech. 43, 583–614.CrossRefGoogle Scholar
King, E.M. & Aurnou, J.M. 2013 Turbulent convection in liquid metal with and without rotation. Proc. Natl Acad. Sci. USA 110 (17), 6688–6693.CrossRefGoogle ScholarPubMed
Lim, Z.L., Chong, K.L., Ding, G.-Y. & Xia, K.-Q. 2019 Quasistatic magnetoconvection: heat transport enhancement and boundary layer crossing. J. Fluid Mech. 870, 519–542.CrossRefGoogle Scholar
Liu, W., Krasnov, D. & Schumacher, J. 2018 Wall modes in magnetoconvection at high Hartmann numbers. J. Fluid Mech. 849, R2.CrossRefGoogle Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.CrossRefGoogle Scholar
Moffatt, K. & Dormy, E. 2019 Self-Exciting Fluid Dynamos. Cambridge University Press.CrossRefGoogle Scholar
Moreau, R. 1999 The fundamentals of MHD related to crystal growth. Prog. Cryst. Growth Charact. Mater. 38 (1–4), 161–194.CrossRefGoogle Scholar
Pan, D.-Y., Huang, Y.-F., Lyu, Z., Yang, J.-C. & Ni, M.-J. 2024 Development of the multichannel pulsed ultrasonic doppler velocimeter for the measurement of liquid metal flow. Exp. Fluids (submitted). arXiv:2409.02815.Google Scholar
Plevachuk, Y., Sklyarchuk, V., Eckert, S., Gerbeth, G. & Novakovic, R. 2014 Thermophysical properties of the liquid Ga–In–Sn eutectic alloy. J. Chem. Engng Data 59 (3), 757–763.CrossRefGoogle Scholar
Plevachuk, Y., Sklyarchuk, V., Eckert, S., Gerbeth, G. & Novakovic, R. 2015 Correction to “thermophysical properties of the liquid Ga–In–Sn eutectic alloy”. J. Chem. Engng Data 60 (9), 2756–2756.CrossRefGoogle Scholar
Ren, L., Tao, X., Zhang, L., Ni, M.-J., Xia, K.-Q. & Xie, Y.-C. 2022 Flow states and heat transport in liquid metal convection. J. Fluid Mech. 951, R1.CrossRefGoogle Scholar
Scheel, J.D. & Schumacher, J. 2016 Global and local statistics in turbulent convection at low Prandtl numbers. J. Fluid Mech. 802, 147–173.CrossRefGoogle Scholar
Schindler, F., Eckert, S., Zürner, T., Schumacher, J. & Vogt, T. 2022 Collapse of coherent large scale flow in strongly turbulent liquid metal convection. Phys. Rev. Lett. 128 (16), 164501.CrossRefGoogle ScholarPubMed
Sommeria, J. & Moreau, R. 1982 Why, how, and when, MHD turbulence becomes two-dimensional. J. Fluid Mech. 118, 507–518.CrossRefGoogle Scholar
Stevens, R.J.A.M., van der Poel, E.P., Grossmann, S. & Lohse, D. 2013 The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. 730, 295–308.CrossRefGoogle Scholar
Sun, C., Xi, H.-D. & Xia, K.-Q. 2005 Azimuthal symmetry, flow dynamics, and heat transport in turbulent thermal convection in a cylinder with an aspect ratio of 0.5. Phys. Rev. Lett. 95 (7), 074502.CrossRefGoogle Scholar
Takeshita, T., Segawa, T., Glazier, J.A. & Sano, M. 1996 Thermal turbulence in mercury. Phys. Rev. Lett. 76 (9), 1465.CrossRefGoogle ScholarPubMed
Tasaka, Y., Igaki, K., Yanagisawa, T., Vogt, T., Zuerner, T. & Eckert, S. 2016 Regular flow reversals in Rayleigh–Bénard convection in a horizontal magnetic field. Phys. Rev. E 93 (4), 043109.CrossRefGoogle Scholar
Vogt, T., Ishimi, W., Yanagisawa, T., Tasaka, Y., Sakuraba, A. & Eckert, S. 2018 Transition between quasi-two-dimensional and three-dimensional Rayleigh–Bénard convection in a horizontal magnetic field. Phys. Rev. Fluids 3 (1), 013503.CrossRefGoogle Scholar
Vogt, T., Yang, J.-C., Schindler, F. & Eckert, S. 2021 Free-fall velocities and heat transport enhancement in liquid metal magneto-convection. J. Fluid Mech. 915, A68.CrossRefGoogle Scholar
Wang, Y., Lai, P.-Y., Song, H. & Tong, P. 2018 Mechanism of large-scale flow reversals in turbulent thermal convection. Sci. Adv. 4 (11), eaat7480.CrossRefGoogle ScholarPubMed
Wei, P. 2021 The persistence of large-scale circulation in Rayleigh–Bénard convection. J. Fluid Mech. 924, A28.CrossRefGoogle Scholar
Weiss, N.O. & Proctor, M.R.E. 2014 Magnetoconvection. Cambridge University Press.CrossRefGoogle Scholar
Weiss, S. & Ahlers, G. 2011 Turbulent Rayleigh–Bénard convection in a cylindrical container with aspect ratio $\varGamma = 0.50$ and Prandtl number $Pr= 4.38$. J. Fluid Mech. 676, 5–40.CrossRefGoogle Scholar
Xi, H.-D. & Xia, K.-Q. 2008 Flow mode transitions in turbulent thermal convection. Phys. Fluids 20 (5), 055104.CrossRefGoogle Scholar
Xie, Y.-C., Ding, G.-Y. & Xia, K.-Q. 2018 Flow topology transition via global bifurcation in thermally driven turbulence. Phys. Rev. Lett. 120 (21), 214501.CrossRefGoogle ScholarPubMed
Xu, A., Chen, X. & Xi, H.-D. 2021 Tristable flow states and reversal of the large-scale circulation in two-dimensional circular convection cells. J. Fluid Mech. 910, A33.CrossRefGoogle Scholar
Xu, A., Xu, B.-R. & Xi, H.-D. 2023 Wall-sheared thermal convection: heat transfer enhancement and turbulence relaminarization. J. Fluid Mech. 960, A2.CrossRefGoogle Scholar
Yan, M., Calkins, M.A., Maffei, S., Julien, K., Tobias, S.M. & Marti, P. 2019 Heat transfer and flow regimes in quasi-static magnetoconvection with a vertical magnetic field. J. Fluid Mech. 877, 1186–1206.CrossRefGoogle Scholar
Yanagisawa, T., Hamano, Y., Miyagoshi, T., Yamagishi, Y., Tasaka, Y. & Takeda, Y. 2013 Convection patterns in a liquid metal under an imposed horizontal magnetic field. Phys. Rev. E 88 (6), 063020.CrossRefGoogle Scholar
Yanagisawa, T., Yamagishi, Y., Hamano, Y., Tasaka, Y. & Takeda, Y. 2011 Spontaneous flow reversals in Rayleigh–Bénard convection of a liquid metal. Phys. Rev. E 83 (3), 036307.CrossRefGoogle ScholarPubMed
Yang, J.C., Vogt, T. & Eckert, S. 2021 Transition from steady to oscillating convection rolls in Rayleigh–Bénard convection under the influence of a horizontal magnetic field. Phys. Rev. Fluids 6 (2), 023502.CrossRefGoogle Scholar
Zürner, T., Liu, W., Krasnov, D. & Schumacher, J. 2016 Heat and momentum transfer for magnetoconvection in a vertical external magnetic field. Phys. Rev. E 94 (4), 043108.CrossRefGoogle Scholar
Zürner, T., Schindler, F., Vogt, T., Eckert, S. & Schumacher, J. 2020 Flow regimes of Rayleigh–Bénard convection in a vertical magnetic field. J. Fluid Mech. 894, A21.CrossRefGoogle Scholar
Zwirner, L., Tilgner, A. & Shishkina, O. 2020 Elliptical instability and multiple-roll flow modes of the large-scale circulation in confined turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 125 (5), 054502.CrossRefGoogle ScholarPubMed