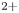1. Introduction
The current development of laser-plasma particle acceleration technology is exhibiting its importance in experiments involving proton and ion particles and the wide applications that they can provide (Daido, Nishiuchi & Pirozhkov Reference Daido, Nishiuchi and Pirozhkov2012; Pommarel et al. Reference Pommarel, Vauzour, Mégnin-Chanet, Bayart, Delmas, Goudjil, Nauraye, Letellier, Pouzoulet, Schillaci, Romano, Scuderi, Cirrone, Deutsch, Flacco and Malka2017). Traditional laser-plasma acceleration of protons and ions is performed when a high-power laser (terawatt (TW) and petawatt (PW) power) irradiates a solid target in a well-known acceleration process called target normal sheath acceleration (TNSA) (Borghesi Reference Borghesi2014). This acceleration mechanism is capable of generating high-energy particle bunches with energy distributions of several tens of MeV (Macchi, Borghesi & Passoni Reference Macchi, Borghesi and Passoni2013). Unfortunately, however, solid targets produce a lot of debris and are generally slow to replace, limiting their usefulness in the new generation of femtosecond laser facilities operating at high repetition rates (HRRs). Laser facilities like APOLLON (France) (Burdonov et al. Reference Burdonov2021), CoReLS (Korea) (Yoon et al. Reference Yoon, Kim, Choi, Sung, Lee, Lee and Nam2021), ELI-NP (Romania) (Radier et al. Reference Radier, Chalus, Charbonneau, Thambirajah, Deschamps, David, Barbe, Etter, Matras and Ricaud2022), ELI-Beamlines L3-HAPLS (Czech Republic) (Borneis et al. Reference Borneis, Laštovička, Sokol, Jeong, Condamine, Renner, Tikhonchuk, Bohlin, Fajstavr and Hernandez2021), BELLA (USA) (Hakimi et al. Reference Hakimi, Obst-Huebl, Huebl, Nakamura, Bulanov, Steinke, Leemans, Kober, Ostermayr, Schenkel, Gonsalves, Vay, van Tilborg, Toth, Schroeder, Esarey and Geddes2022) or CLPU-VEGA3 (Spain) (Roso Reference Roso2018) will support shot rates from 1 to 10 Hz. The interest in the possibility of new physics and direct applications due to the increase in the number of generated particles and the capability to deliver high-energy particles in short periods of time triggered the development of alternative targets. Several alternatives to provide HRR targets are currently being developed worldwide to overcome the above-commented limitations: multistage solid targets (Dover et al. Reference Dover2020; Loughran et al. Reference Loughran, Streeter, Ahmed, Astbury, Balcazar, Borghesi, Bourgeois, Curry, Dann and DiIorio2023), cryogenic ribbons (Margarone et al. Reference Margarone2016; Rehwald et al. Reference Rehwald2023), liquid targets (Hilz et al. Reference Hilz, Ostermayr, Huebl, Bagnoud, Borm, Bussmann, Gallei, Gebhard, Haffa, Hartmann, Kluge, Lindner, Neumayr, Schaefer, Schramm, Thirolf, Rösch, Wagner, Zielbauer and Schreiber2018; Puyuelo-Valdes et al. Reference Puyuelo-Valdes, de Luis, Hernandez, Apiñaniz, Curcio, Henares, Huault, Pérez-Hernández, Roso, Gatti and Volpe2022) or gas-jet targets (Sylla et al. Reference Sylla, Veltcheva, Kahaly, Flacco and Malka2012).
Here we will focus on the development of near-critical density gas-jet targets for laser-plasma ion acceleration. High-density gases can reach near-critical electron densities once fully ionized ($n_c \approx 10^{21}\ {\rm cm}^{-3}$![]() depending on the laser wavelength). The critical density is a region of interest for laser-plasma acceleration for the different acceleration regimes that can be triggered and the enhancement of the coupling efficiency of the laser to the plasma compared to underdense or overdense plasmas. In addition, gas targets have several advantages. The main one is the implicit self-refreshment of the target after the laser interaction which makes them a perfect candidate for high repetition rate operation. They also produce relatively pure ion beams since the gas mix purity can be controlled. Finally, gas targets can be optically probed that makes them interesting to study alternative acceleration schemes and to understand underlying physics.
depending on the laser wavelength). The critical density is a region of interest for laser-plasma acceleration for the different acceleration regimes that can be triggered and the enhancement of the coupling efficiency of the laser to the plasma compared to underdense or overdense plasmas. In addition, gas targets have several advantages. The main one is the implicit self-refreshment of the target after the laser interaction which makes them a perfect candidate for high repetition rate operation. They also produce relatively pure ion beams since the gas mix purity can be controlled. Finally, gas targets can be optically probed that makes them interesting to study alternative acceleration schemes and to understand underlying physics.
High-density gas targets have been used for electron acceleration (Faure et al. Reference Faure, Glinec, Pukhov, Kiselev, Gordienko, Lefebvre, Rousseau, Burgy and Malka2004; Geddes et al. Reference Geddes, Toth, van Tilborg, Esarey, Schroeder, Bruhwiler, Nieter, Cary and Leemans2004; Mangles et al. Reference Mangles, Murphy, Najmudin, Thomas, Collier, Dangor, Divall, Foster, Gallacher, Hooker, Jaroszynski, Langley, Mori, Norreys, Tsung, Viskup, Walton and Krushelnick2004) and high repetition rate operation is now available (Guénot et al. Reference Guénot, Gustas, Vernier, Beaurepaire, Böhle, Bocoum, Lozano, Jullien, Lopez-Martens, Lifschitz and Faure2017; Faure et al. Reference Faure, Gustas, Guénot, Vernier, Böhle, Ouillé, Haessler, Lopez-Martens and Lifschitz2018). Laser-plasma ion acceleration has been moderately studied with CO$_2$![]() lasers ($\lambda =10\ \mathrm {\mu }$
lasers ($\lambda =10\ \mathrm {\mu }$![]() m, $n_c=1.12 \times 10^{19}\ {\rm cm}^{-3}$
m, $n_c=1.12 \times 10^{19}\ {\rm cm}^{-3}$![]() ) (Palmer et al. Reference Palmer, Dover, Pogorelsky, Babzien, Dudnikova, Ispiriyan, Polyanskiy, Schreiber, Shkolnikov, Yakimenko and Najmudin2011; Haberberger et al. Reference Haberberger, Tochitsky, Fiuza, Gong, Fonseca, Silva, Mori and Joshi2012; Tresca et al. Reference Tresca, Dover, Cook, Maharjan, Polyanskiy, Najmudin, Shkolnikov and Pogorelsky2015). Haberberger et al. (Reference Haberberger, Tochitsky, Fiuza, Gong, Fonseca, Silva, Mori and Joshi2012) have demonstrated the production of proton beams up to 20 MeV with an extremely narrow energy spread. Collisionless shockwave acceleration (CSA) was identified as the main acceleration mechanism in that experiment (Fiuza et al. Reference Fiuza, Stockem, Boella, Fonseca, Silva, Haberberger, Tochitsky, Gong, Mori and Joshi2012). Then, the interaction with Nd:YAG lasers ($\lambda =1\ \mathrm {\mu }$
) (Palmer et al. Reference Palmer, Dover, Pogorelsky, Babzien, Dudnikova, Ispiriyan, Polyanskiy, Schreiber, Shkolnikov, Yakimenko and Najmudin2011; Haberberger et al. Reference Haberberger, Tochitsky, Fiuza, Gong, Fonseca, Silva, Mori and Joshi2012; Tresca et al. Reference Tresca, Dover, Cook, Maharjan, Polyanskiy, Najmudin, Shkolnikov and Pogorelsky2015). Haberberger et al. (Reference Haberberger, Tochitsky, Fiuza, Gong, Fonseca, Silva, Mori and Joshi2012) have demonstrated the production of proton beams up to 20 MeV with an extremely narrow energy spread. Collisionless shockwave acceleration (CSA) was identified as the main acceleration mechanism in that experiment (Fiuza et al. Reference Fiuza, Stockem, Boella, Fonseca, Silva, Haberberger, Tochitsky, Gong, Mori and Joshi2012). Then, the interaction with Nd:YAG lasers ($\lambda =1\ \mathrm {\mu }$![]() m, $n_c=1 \times 10^{21}\ {\rm cm}^{-3}$
m, $n_c=1 \times 10^{21}\ {\rm cm}^{-3}$![]() ) was studied by several groups leading to acceleration of protons up to 600 keV (Chen et al. Reference Chen, Vranic, Gangolf, Boella, Antici, Bailly-Grandvaux, Loiseau, Pépin, Revet, Santos, Schroer, Starodubtsev, Willi, Silva, d'Humières and Fuchs2017), and Puyuelo-Valdes et al. (Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Ribeyre, Santos, Tikhonchuk and Tarisien2019a) measured protons up to 6 MeV with a slightly peaked spectra and He$^{2+}$
) was studied by several groups leading to acceleration of protons up to 600 keV (Chen et al. Reference Chen, Vranic, Gangolf, Boella, Antici, Bailly-Grandvaux, Loiseau, Pépin, Revet, Santos, Schroer, Starodubtsev, Willi, Silva, d'Humières and Fuchs2017), and Puyuelo-Valdes et al. (Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Ribeyre, Santos, Tikhonchuk and Tarisien2019a) measured protons up to 6 MeV with a slightly peaked spectra and He$^{2+}$![]() ions up to 15 MeV in the transverse direction (Puyuelo-Valdes et al. Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Santos and Tarisien2019b). Hole boring (HB) (Wilks et al. Reference Wilks, Kruer, Tabak and Langdon1992) was identified as the main acceleration mechanism. Despite excellent results, experiments carried out with CO$_2$
ions up to 15 MeV in the transverse direction (Puyuelo-Valdes et al. Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Santos and Tarisien2019b). Hole boring (HB) (Wilks et al. Reference Wilks, Kruer, Tabak and Langdon1992) was identified as the main acceleration mechanism. Despite excellent results, experiments carried out with CO$_2$![]() and Nd:YAG are limited to low repetition rates (less than a few shots per hour). With the recent development of HRR Ti:Sa laser systems ($\lambda =800$
and Nd:YAG are limited to low repetition rates (less than a few shots per hour). With the recent development of HRR Ti:Sa laser systems ($\lambda =800$![]() nm, $n_c=1.75 \times 10^{21}\ {\rm cm}^{-3}$
nm, $n_c=1.75 \times 10^{21}\ {\rm cm}^{-3}$![]() ), a significant research effort is now devoted to producing ion beams with high energy and high average flux, such as might be useful for medical isotope production (Spencer et al. Reference Spencer, Ledingham, Singhal, McCanny, McKenna, Clark, Krushelnick, Zepf, Beg, Tatarakis, Dangor, Norreys, Clarke, Allott and Ross2001) or cancer therapy (Ledingham et al. Reference Ledingham, Bolton, Shikazono and Ma2014). Sylla et al. (Reference Sylla, Flacco, Kahaly, Veltcheva, Lifschitz, Malka, d'Humières, Andriyash and Tikhonchuk2013) reported transverse acceleration of He$^+$
), a significant research effort is now devoted to producing ion beams with high energy and high average flux, such as might be useful for medical isotope production (Spencer et al. Reference Spencer, Ledingham, Singhal, McCanny, McKenna, Clark, Krushelnick, Zepf, Beg, Tatarakis, Dangor, Norreys, Clarke, Allott and Ross2001) or cancer therapy (Ledingham et al. Reference Ledingham, Bolton, Shikazono and Ma2014). Sylla et al. (Reference Sylla, Flacco, Kahaly, Veltcheva, Lifschitz, Malka, d'Humières, Andriyash and Tikhonchuk2013) reported transverse acceleration of He$^+$![]() ions up to 250 keV with a Ti:Sa laser. They optically probed the laser plasma interaction zone, which revealed channelling of the laser, self-focusing and an efficient energy deposition at the point of laser beam collapse. Singh et al. (Reference Singh, Pathak, Shin, Choi, Nakajima, Lee, Sung, Lee, Rhee, Aniculaesei, Kim, Pae, Cho, Hojbota, Lee, Mollica, Malka, Ryu, Kim and Nam2020) measured protons and He$^{2+}$
ions up to 250 keV with a Ti:Sa laser. They optically probed the laser plasma interaction zone, which revealed channelling of the laser, self-focusing and an efficient energy deposition at the point of laser beam collapse. Singh et al. (Reference Singh, Pathak, Shin, Choi, Nakajima, Lee, Sung, Lee, Rhee, Aniculaesei, Kim, Pae, Cho, Hojbota, Lee, Mollica, Malka, Ryu, Kim and Nam2020) measured protons and He$^{2+}$![]() ions up to 750 keV in the transverse direction, characterized by an exponentially decaying spectra and accelerated through mass-dependent radial CSA. Recently, an experimental campaign was carried out at CLPU-VEGA3 by Ospina-Bohórquez et al. (Reference Ospina-Bohórquez2023) where alpha particles were detected with ${\approx }1$
ions up to 750 keV in the transverse direction, characterized by an exponentially decaying spectra and accelerated through mass-dependent radial CSA. Recently, an experimental campaign was carried out at CLPU-VEGA3 by Ospina-Bohórquez et al. (Reference Ospina-Bohórquez2023) where alpha particles were detected with ${\approx }1$![]() MeV amu$^{-1}$
MeV amu$^{-1}$![]() at 17$^\circ$
at 17$^\circ$![]() . The experiment was supported by simulations that show acceleration from a CSA-type mechanism at the rapidly expanding walls of the laser-induced plasma channel. Some interesting ideas are being developed such as tailoring the gas density profile to optimize the acceleration process (Marquès et al. Reference Marquès2021) or asymmetric nozzles (Rovige et al. Reference Rovige, Huijts, Vernier, Andriyash, Sylla, Tomkus, Girdauskas, Raciukaitis, Dudutis, Stankevic, Gecys and Faure2021). Here we present the successful acceleration of multi-MeV protons and He$^{2+}$
. The experiment was supported by simulations that show acceleration from a CSA-type mechanism at the rapidly expanding walls of the laser-induced plasma channel. Some interesting ideas are being developed such as tailoring the gas density profile to optimize the acceleration process (Marquès et al. Reference Marquès2021) or asymmetric nozzles (Rovige et al. Reference Rovige, Huijts, Vernier, Andriyash, Sylla, Tomkus, Girdauskas, Raciukaitis, Dudutis, Stankevic, Gecys and Faure2021). Here we present the successful acceleration of multi-MeV protons and He$^{2+}$![]() ions at a high repetition rate Ti:Sa PW-class laser facility. This was achieved via optimization of the laser interaction through interferometry and transverse 2w-emission imaging. A study of nozzle damage is also presented, which is the main limitation for producing high repetition rate laser-driven ion beams for various applications.
ions at a high repetition rate Ti:Sa PW-class laser facility. This was achieved via optimization of the laser interaction through interferometry and transverse 2w-emission imaging. A study of nozzle damage is also presented, which is the main limitation for producing high repetition rate laser-driven ion beams for various applications.
2. Experimental set-up
The experiment was carried out in the VEGA3 laser facility at CLPU (Spain). The laser system consists of a Ti:Sa laser ($\lambda =800$![]() nm) that can deliver energies up to 30 J, temporal duration up to 30 fs and up to 1 Hz repetition rate. The laser beam is focused by an $f$
nm) that can deliver energies up to 30 J, temporal duration up to 30 fs and up to 1 Hz repetition rate. The laser beam is focused by an $f$![]() /11 off-axis parabolic mirror to a spot of $12\ \mathrm {\mu }$
/11 off-axis parabolic mirror to a spot of $12\ \mathrm {\mu }$![]() m full-width at half-maximum (FWHM). The energy on target is extrapolated from calibrations recorded at low energy, and the focal spot at high energy is estimated to remain the same. We estimate that 21 % of the energy on target is within the first Airy disk at FWHM, based on images of the focal spot taken at low energy and taking into account compressor and transport loses. This gives an effective energy of approximately 6.3 J on target. The laser energy and pulse duration are measured on-shot (the latter using a second harmonic autocorrelator system). The laser energy remained constant throughout this study but the temporal pulse duration was fixed up to a maximum of 300 fs for the whole experiment (negative chirp). The reason for using 300 fs was to compare with the results obtained in picosecond laser pulse facilities (such as the experiment by Puyuelo-Valdes et al. (Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Ribeyre, Santos, Tikhonchuk and Tarisien2019a)) and then to move to shorter pulse durations. However, the laser beam time duration for this experimental campaign was not enough to perform the full study. The intensity used in this study was $1.85 \times 10^{19}\ {\rm W}\ {\rm cm}^{-2}$
m full-width at half-maximum (FWHM). The energy on target is extrapolated from calibrations recorded at low energy, and the focal spot at high energy is estimated to remain the same. We estimate that 21 % of the energy on target is within the first Airy disk at FWHM, based on images of the focal spot taken at low energy and taking into account compressor and transport loses. This gives an effective energy of approximately 6.3 J on target. The laser energy and pulse duration are measured on-shot (the latter using a second harmonic autocorrelator system). The laser energy remained constant throughout this study but the temporal pulse duration was fixed up to a maximum of 300 fs for the whole experiment (negative chirp). The reason for using 300 fs was to compare with the results obtained in picosecond laser pulse facilities (such as the experiment by Puyuelo-Valdes et al. (Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Ribeyre, Santos, Tikhonchuk and Tarisien2019a)) and then to move to shorter pulse durations. However, the laser beam time duration for this experimental campaign was not enough to perform the full study. The intensity used in this study was $1.85 \times 10^{19}\ {\rm W}\ {\rm cm}^{-2}$![]() . The laser contrast of VEGA3 is up to $10^{-12}$
. The laser contrast of VEGA3 is up to $10^{-12}$![]() at 0.1 ns with no significant pre-pulses that could ionize and thus modify the accelerating properties of the gas target.
at 0.1 ns with no significant pre-pulses that could ionize and thus modify the accelerating properties of the gas target.
The set-up of the experiment is shown in figure 1. The gas used is a mixture of He (97 %) and H$_2$![]() (3 %). The gas target consist of a supersonic gas-jet nozzle that produces a high-density region away from the nozzle exit. The gas travels vertically out of the nozzle along the $z$
(3 %). The gas target consist of a supersonic gas-jet nozzle that produces a high-density region away from the nozzle exit. The gas travels vertically out of the nozzle along the $z$![]() -axis, where zero distance is defined at the nozzle surface (Henares et al. Reference Henares, Tarisien, Puyuelo, Marquès, Nguyen-Bui, Gobet, Raymond, Versteegen and Hannachi2018). This target can produce near-critical electron densities when fully ionized. For reference, the critical density is $n_c=1.74 \times 10^{21}\ {\rm cm}^{-3}$
-axis, where zero distance is defined at the nozzle surface (Henares et al. Reference Henares, Tarisien, Puyuelo, Marquès, Nguyen-Bui, Gobet, Raymond, Versteegen and Hannachi2018). This target can produce near-critical electron densities when fully ionized. For reference, the critical density is $n_c=1.74 \times 10^{21}\ {\rm cm}^{-3}$![]() for our Ti:Sa laser. The nozzle design consists of a convergent-divergent geometry with a straight duct at the exit (Rovige et al. Reference Rovige, Huijts, Vernier, Andriyash, Sylla, Tomkus, Girdauskas, Raciukaitis, Dudutis, Stankevic, Gecys and Faure2021). The hydrodynamic flow follows a supersonic expansion due to the convergent-divergent region whereas the straight part makes the gas converge, producing a sharp maximum transverse density profile from the accumulation of oblique shocks. The gas-jet nozzle is mounted on a Clark Cooper solenoid valve EX30 that can provide continuous or pulsed gas flux. The high density is reached by using 1000 bars inlet pressure delivered by a Haskel gas booster model AGT-62/152. The nozzle is designed to produce a high-density shock at distances ranging in $Z$
for our Ti:Sa laser. The nozzle design consists of a convergent-divergent geometry with a straight duct at the exit (Rovige et al. Reference Rovige, Huijts, Vernier, Andriyash, Sylla, Tomkus, Girdauskas, Raciukaitis, Dudutis, Stankevic, Gecys and Faure2021). The hydrodynamic flow follows a supersonic expansion due to the convergent-divergent region whereas the straight part makes the gas converge, producing a sharp maximum transverse density profile from the accumulation of oblique shocks. The gas-jet nozzle is mounted on a Clark Cooper solenoid valve EX30 that can provide continuous or pulsed gas flux. The high density is reached by using 1000 bars inlet pressure delivered by a Haskel gas booster model AGT-62/152. The nozzle is designed to produce a high-density shock at distances ranging in $Z$![]() values from 900 to 1500 mm depending on the construction parameters. The generated density profile was calculated using computational fluid dynamics (CFD) simulations by the FLUENT software (ANSYS FLUENT Academic Research Mechanical 2022), which numerically solves the Navier–Stokes equations on a discrete grid (additional information can be found in Henares et al. Reference Henares, Puyuelo-Valdes, Hannachi, Ceccotti, Ehret, Gobet, Lancia, Marquès, Santos, Versteegen and Tarisien2019). Peak atomic densities in the interval between $1 \times 10^{21}\ {\rm cm}^{-3}$
values from 900 to 1500 mm depending on the construction parameters. The generated density profile was calculated using computational fluid dynamics (CFD) simulations by the FLUENT software (ANSYS FLUENT Academic Research Mechanical 2022), which numerically solves the Navier–Stokes equations on a discrete grid (additional information can be found in Henares et al. Reference Henares, Puyuelo-Valdes, Hannachi, Ceccotti, Ehret, Gobet, Lancia, Marquès, Santos, Versteegen and Tarisien2019). Peak atomic densities in the interval between $1 \times 10^{21}\ {\rm cm}^{-3}$![]() and $0.5 \times 10^{21}\ {\rm cm}^{-3}$
and $0.5 \times 10^{21}\ {\rm cm}^{-3}$![]() were obtained for lower $Z$
were obtained for lower $Z$![]() distances and higher $Z$
distances and higher $Z$![]() distances of the shock, respectively. Tungsten and UVFS fused silica materials were studied for the nozzle construction. The gas target is mounted in a $XYZ$
distances of the shock, respectively. Tungsten and UVFS fused silica materials were studied for the nozzle construction. The gas target is mounted in a $XYZ$![]() axis motor platform to finely position the shock at the interaction point with respect to the calculated one.
axis motor platform to finely position the shock at the interaction point with respect to the calculated one.

Figure 1. Set-up of the experiment and diagnostics in the VEGA3 experimental chamber (see text for details). The graphics on the right show (top) a phase map image of the shock obtained with PHASICS and (bottom) the density profiles obtained by computational fluid dynamics simulations of a shock nozzle at $Z = 900\ \mathrm {\mu }$![]() m (blue) and interferometric density measurement (green).
m (blue) and interferometric density measurement (green).
Three Thomson parabola (TP) spectrometers, placed at 0$^\circ$![]() , 60$^\circ$
, 60$^\circ$![]() and 90$^\circ$
and 90$^\circ$![]() with respect to the laser forward axis, were used to characterize ion emission from the target. A TP consists of a magnetic dipole followed by a pair of electric plates which allow to measure the energy distributions of different ion species. BAS-MS and BAS-SR imaging plates (IPs) were used to record the particles and they were analysed using a FUJIFILM FLA-7000 reader. The IPs were protected by aluminized Mylar foils of $2\ \mathrm {\mu }$
with respect to the laser forward axis, were used to characterize ion emission from the target. A TP consists of a magnetic dipole followed by a pair of electric plates which allow to measure the energy distributions of different ion species. BAS-MS and BAS-SR imaging plates (IPs) were used to record the particles and they were analysed using a FUJIFILM FLA-7000 reader. The IPs were protected by aluminized Mylar foils of $2\ \mathrm {\mu }$![]() m thickness. The IP response functions to ions are taken from Bonnet et al. (Reference Bonnet, Comet, Denis-Petit, Gobet, Hannachi, Tarisien, Versteegen and Aleonard2013). The sensitivity limitations (including Mylar protective foils) for IP-MS are 0.78 MeV for protons and 2.7 MeV for He ions, and for IP-SR are 0.62 MeV for protons and 2.5 MeV for He ions. TPs were calibrated by measuring the magnetic field between the plates and then comparing the expected particle trajectories with results from particle accelerators. TPs were positioned at 35 cm from the gas target with $500\ \mathrm {\mu }$
m thickness. The IP response functions to ions are taken from Bonnet et al. (Reference Bonnet, Comet, Denis-Petit, Gobet, Hannachi, Tarisien, Versteegen and Aleonard2013). The sensitivity limitations (including Mylar protective foils) for IP-MS are 0.78 MeV for protons and 2.7 MeV for He ions, and for IP-SR are 0.62 MeV for protons and 2.5 MeV for He ions. TPs were calibrated by measuring the magnetic field between the plates and then comparing the expected particle trajectories with results from particle accelerators. TPs were positioned at 35 cm from the gas target with $500\ \mathrm {\mu }$![]() m-diameter entrance pinholes. A high voltage was used to deflect the particles. They will be referenced as TP0 (0$^\circ$
m-diameter entrance pinholes. A high voltage was used to deflect the particles. They will be referenced as TP0 (0$^\circ$![]() ), TP60 (60$^\circ$
), TP60 (60$^\circ$![]() ) and TP90 (90$^\circ$
) and TP90 (90$^\circ$![]() ). More specific information of TP0 can be found from Salgado-López et al. (Reference Salgado-López, Apiñaniz, Henares, Pérez-Hernández, de Luis, Volpe and Gatti2022), and for TP60 and TP90 from Baccou (Reference Baccou2016).
). More specific information of TP0 can be found from Salgado-López et al. (Reference Salgado-López, Apiñaniz, Henares, Pérez-Hernández, de Luis, Volpe and Gatti2022), and for TP60 and TP90 from Baccou (Reference Baccou2016).
The imaging system consists of a microscope to calculate the focal spot and to determine the pointing. In the same path, a wavefront sensor PHASICS SID4 interferometer (Plateau et al. Reference Plateau, Matlis, Geddes, Gonsalves, Shiraishi, Lin, van Mourik and Leemans2010) was installed to estimate the rate of damage of the nozzle after the shot, to correlate the reference of the laser interaction point with the position of the shock nozzle and, in some cases, to measure the real density before each shot. Using the latter allowed to finely position the shock in transverse and vertical planes with respect to the laser axis within an error of $3\ \mathrm {\mu }$![]() m. The longitudinal position of the shock nozzle was arranged by a camera at 90$^\circ$
m. The longitudinal position of the shock nozzle was arranged by a camera at 90$^\circ$![]() with respect to the laser axis. This camera was also used to set a diagnostic to measure the 2$\omega$
with respect to the laser axis. This camera was also used to set a diagnostic to measure the 2$\omega$![]() emission of the plasma generated by the laser interaction.
emission of the plasma generated by the laser interaction.
3. Results
Optimization of the laser interaction was carried out in two stages. Before the laser shot, the wavefront sensor PHASICS SID4 measured the density profile and the position of the shock was determined. After the laser shot, the $2 \omega$![]() diagnostic was used to fine optimize the intensity of the emission of the plasma generated by the interaction. Figure 2 shows an example of the optimization in $XYZ$
diagnostic was used to fine optimize the intensity of the emission of the plasma generated by the interaction. Figure 2 shows an example of the optimization in $XYZ$![]() directions (longitudinal $L$
directions (longitudinal $L$![]() , transversal $T$
, transversal $T$![]() and vertical $V$
and vertical $V$![]() , respectively). One should note that vertical movements $V$
, respectively). One should note that vertical movements $V$![]() are relative movements with respect to the reference and they are different to the absolute distance of the shock from the nozzle exit ($Z$
are relative movements with respect to the reference and they are different to the absolute distance of the shock from the nozzle exit ($Z$![]() ). The nozzle assembly was moved in all directions and major changes in the emission were observed when exiting the high-density region. The transverse sensitivity of the interaction using shock nozzles was observed to be very high: no signal was observed outside of a $100\ \mathrm {\mu }$
). The nozzle assembly was moved in all directions and major changes in the emission were observed when exiting the high-density region. The transverse sensitivity of the interaction using shock nozzles was observed to be very high: no signal was observed outside of a $100\ \mathrm {\mu }$![]() m range. The range in longitudinal position to obtain a clear interaction was larger (estimated to be approximately $200\ \mathrm {\mu }$
m range. The range in longitudinal position to obtain a clear interaction was larger (estimated to be approximately $200\ \mathrm {\mu }$![]() m) probably due to the laser interaction in the density profile wings and the laser Rayleigh length (${>}200\ \mathrm {\mu }$
m) probably due to the laser interaction in the density profile wings and the laser Rayleigh length (${>}200\ \mathrm {\mu }$![]() m). It was not possible to determine if the highest efficiency was found when the laser was focused at the centre of the density profile of the gas jet or in the rising slope (the latter effect was observed using De Laval nozzles in previous experiments). The vertical optimization range was estimated to be larger, but no reliable measurement could be performed and nozzles rapidly degraded when approaching the interaction point to the nozzle exit.
m). It was not possible to determine if the highest efficiency was found when the laser was focused at the centre of the density profile of the gas jet or in the rising slope (the latter effect was observed using De Laval nozzles in previous experiments). The vertical optimization range was estimated to be larger, but no reliable measurement could be performed and nozzles rapidly degraded when approaching the interaction point to the nozzle exit.

Figure 2. Camera recording the 2$\omega$![]() plasma interaction region at 90$^\circ$
plasma interaction region at 90$^\circ$![]() with respect to the laser axis (the red arrow indicates the laser direction). The pseudo-colour images are only processed assigning a lookup table. The first position in panel (a) is where the maximum density is expected by interferometry ($Z = 1350\ \mathrm {\mu }$
with respect to the laser axis (the red arrow indicates the laser direction). The pseudo-colour images are only processed assigning a lookup table. The first position in panel (a) is where the maximum density is expected by interferometry ($Z = 1350\ \mathrm {\mu }$![]() m). The laser is fixed at 0 position and the nozzle has been moved to different positions to optimize the signal (relative movements with respect to the reference): (a) starting point; (b) nozzle moved longitudinal (laser axis) $-$
m). The laser is fixed at 0 position and the nozzle has been moved to different positions to optimize the signal (relative movements with respect to the reference): (a) starting point; (b) nozzle moved longitudinal (laser axis) $-$![]() 0.2 mm; (c–f) nozzle moved transversely $-$
0.2 mm; (c–f) nozzle moved transversely $-$![]() 0.05 mm, centred and $+$
0.05 mm, centred and $+$![]() 0.05 mm, and back again to the centred position, respectively; (g) nozzle moved up to 1400 mm ($+$
0.05 mm, and back again to the centred position, respectively; (g) nozzle moved up to 1400 mm ($+$![]() 0.05 mm); (h) centred again in vertical, some damage starts to be seen; (i,j) nozzle moved longitudinal (laser axis) $-$
0.05 mm); (h) centred again in vertical, some damage starts to be seen; (i,j) nozzle moved longitudinal (laser axis) $-$![]() 0.3 mm and then $+$
0.3 mm and then $+$![]() 0.2 mm; (k) nozzle centred again in the maximum signal position. The nozzle now shows evidence of damage.
0.2 mm; (k) nozzle centred again in the maximum signal position. The nozzle now shows evidence of damage.
For every shot and at each angle, the traces of protons, He$^{1+}$![]() and He$^{2+}$
and He$^{2+}$![]() , were analysed. For each ion species, a parabolic trace and a background zone close to it were isolated on the IP. The background was carefully subtracted for each parabola. He$^{1+}$
, were analysed. For each ion species, a parabolic trace and a background zone close to it were isolated on the IP. The background was carefully subtracted for each parabola. He$^{1+}$![]() traces were not observed, so it is possible to consider that the high-intensity laser fully ionized both gases during the interaction. Since the IPs are passive detectors, the accumulation of several laser interactions was performed. To avoid fading effects, the IPs were scanned within 20 minutes after the laser interaction.
traces were not observed, so it is possible to consider that the high-intensity laser fully ionized both gases during the interaction. Since the IPs are passive detectors, the accumulation of several laser interactions was performed. To avoid fading effects, the IPs were scanned within 20 minutes after the laser interaction.
3.1. $H^{+}$ acceleration
acceleration
Figures 3(a)–3(c) show representative proton energy spectra for angles 0$^\circ$![]() , 60$^\circ$
, 60$^\circ$![]() and 90$^\circ$
and 90$^\circ$![]() in an accumulation of five shots using IP-MS. All shots performed in the campaign showed proton signal in the 90$^\circ$
in an accumulation of five shots using IP-MS. All shots performed in the campaign showed proton signal in the 90$^\circ$![]() and 60$^\circ$
and 60$^\circ$![]() detectors, but only some shots could accelerate protons in the forward direction. Figure 3(a) presents the energy spectra detected at 0$^\circ$
detectors, but only some shots could accelerate protons in the forward direction. Figure 3(a) presents the energy spectra detected at 0$^\circ$![]() . It is characterized by a decreasing exponential up to 2 MeV and up to 10$^{10}$
. It is characterized by a decreasing exponential up to 2 MeV and up to 10$^{10}$![]() protons/MeV/sr directed along the laser propagation axis. The energy spectra at 60$^\circ$
protons/MeV/sr directed along the laser propagation axis. The energy spectra at 60$^\circ$![]() and 90$^\circ$
and 90$^\circ$![]() show a sharp cut-off at 1.75 and 2.2 MeV, respectively (figures 3b and 3c). It is important to note the two exponential distributions in the energy spectrum at 90$^\circ$
show a sharp cut-off at 1.75 and 2.2 MeV, respectively (figures 3b and 3c). It is important to note the two exponential distributions in the energy spectrum at 90$^\circ$![]() (discussed later), the first one decreasing (if extrapolated) at 1.75 MeV and the second at 2.2 MeV, and the increase of the number of particles up to 10$^{12}$
(discussed later), the first one decreasing (if extrapolated) at 1.75 MeV and the second at 2.2 MeV, and the increase of the number of particles up to 10$^{12}$![]() protons/MeV/sr for the lower energies, compared to the value of 10$^{10}$
protons/MeV/sr for the lower energies, compared to the value of 10$^{10}$![]() protons/MeV/sr detected at 60$^\circ$
protons/MeV/sr detected at 60$^\circ$![]() at the same energies. Figure 3(d) is a special case where an isolated bunch was observed at 60$^\circ$
at the same energies. Figure 3(d) is a special case where an isolated bunch was observed at 60$^\circ$![]() angle showing energies up to 2.3 MeV, with a maximum number of particles of 10$^{10}$
angle showing energies up to 2.3 MeV, with a maximum number of particles of 10$^{10}$![]() protons/MeV/sr at approximately 1.75 MeV. The energy spectra at 90$^\circ$
protons/MeV/sr at approximately 1.75 MeV. The energy spectra at 90$^\circ$![]() of this case showed a decreasing exponential up to 1.5 MeV (not shown).
of this case showed a decreasing exponential up to 1.5 MeV (not shown).

Figure 3. Proton energy spectra accumulated over five shots and measured at different detection angles with IP-MS: (a) 0$^\circ$![]() with maximum energies of 2 MeV; (b) 60$^\circ$
with maximum energies of 2 MeV; (b) 60$^\circ$![]() with maximum energies of 1.75 MeV and (c) 90$^\circ$
with maximum energies of 1.75 MeV and (c) 90$^\circ$![]() with maximum energies of 2.2 MeV. (d) Spectrum accumulated over 10 shots measured at 60$^\circ$
with maximum energies of 2.2 MeV. (d) Spectrum accumulated over 10 shots measured at 60$^\circ$![]() showing an energy bunch up to 2.3 MeV. The blue-dashed line marks the background noise spectrum.
showing an energy bunch up to 2.3 MeV. The blue-dashed line marks the background noise spectrum.
3.2. He$^{2+}$ acceleration
acceleration
Only perfectly 2$\omega$![]() -optimized laser-target interactions showed He signal in the 90$^\circ$
-optimized laser-target interactions showed He signal in the 90$^\circ$![]() detector, while no He signal was observed at 60$^\circ$
detector, while no He signal was observed at 60$^\circ$![]() and 0$^\circ$
and 0$^\circ$![]() . Figure 4(a) shows the results of the energy spectrum of He ions at 90$^\circ$
. Figure 4(a) shows the results of the energy spectrum of He ions at 90$^\circ$![]() . Figure 4(b) shows the scanned IP-SR with the expected parabolas path to distinguish species. The He$^{2+}$
. Figure 4(b) shows the scanned IP-SR with the expected parabolas path to distinguish species. The He$^{2+}$![]() and H$^+$
and H$^+$![]() traces do not overlap. He$^{1+}$
traces do not overlap. He$^{1+}$![]() was not observed and full ionization was considered without recombinations. The maximum He$^{2+}$
was not observed and full ionization was considered without recombinations. The maximum He$^{2+}$![]() energies were 5.8 MeV with a number of particles up to $10^9$
energies were 5.8 MeV with a number of particles up to $10^9$![]() particle/MeV/sr.
particle/MeV/sr.

Figure 4. (a) He$^{2+}$![]() energy spectrum measured at 90$^\circ$
energy spectrum measured at 90$^\circ$![]() with IP-SR showing a maximum energy up to 5.8 MeV (accumulation of three shots). The blue-dashed line indicates the background noise spectrum. (b) Scanned IP-SR that shows the different parabolic traces of proton and He$^{2+}$
with IP-SR showing a maximum energy up to 5.8 MeV (accumulation of three shots). The blue-dashed line indicates the background noise spectrum. (b) Scanned IP-SR that shows the different parabolic traces of proton and He$^{2+}$![]() . Expected deflection is indicated by a red dashed line (He$^{1+}$
. Expected deflection is indicated by a red dashed line (He$^{1+}$![]() is also indicated to distinguish species). The He right part of the trace stops in the cut-off for the sensitivity of IP-SR (indicated by a black line). The zero deflection is shown in the circle coming from direct X-rays. The exit of the nozzle is also visible due to X-ray imaging by the pinhole.
is also indicated to distinguish species). The He right part of the trace stops in the cut-off for the sensitivity of IP-SR (indicated by a black line). The zero deflection is shown in the circle coming from direct X-rays. The exit of the nozzle is also visible due to X-ray imaging by the pinhole.
3.3. Electron acceleration
In addition, the electron signature was observed along the forward direction using a larger IP-MS in the TP spectrometer (see figure 5). Since the TP is not built to specifically measure electrons, the background noise was too high for spectrum reconstruction. The maximum electron energies were calculated according to the minimum visible signal over the background. This estimation gave maximum energies of approximately 70 MeV and the minimum energies observed were 32 MeV, where the trace exits the IP. This maximum energy value is well above the expected ponderomotive scaling defined by Wilks et al. (Reference Wilks, Langdon, Cowan, Roth, Singh, Hatchett, Key, Pennington, MacKinnon and Snavely2001), but this enhancement is the clear imprint of a near-critical interaction seen by Debayle et al. (Reference Debayle, Mollica, Vauzour, Wan, Flacco, Malka, Davoine and Gremillet2017). Specific set-up of the TP will be needed to measure the electron spectrum in the propagation axis.

Figure 5. Electron trace in a scanned IP-MS at 0$^\circ$![]() . The voltage of the TP was set to 0. The pseudo-colour image is processed assigning a lookup table and selecting the appropriate range of brightness/contrast for clarification.
. The voltage of the TP was set to 0. The pseudo-colour image is processed assigning a lookup table and selecting the appropriate range of brightness/contrast for clarification.
4. Discussion
Transverse acceleration of protons and ions can be explained by laser self-focusing, collapse and electron expulsion in radial directions (Krushelnick et al. Reference Krushelnick, Clark, Najmudin, Salvati, Santala, Tatarakis, Dangor, Malka, Neely, Allott and Danson1999). Then the ponderomotive force distributes the electron perpendicular to the laser beam direction, and the ions are pulled and subsequently accelerated. This behaviour has been seen in several references (Sarkisov et al. Reference Sarkisov, Bychenkov, Novikov, Tikhonchuk, Maksimchuk, Chen, Wagner, Mourou and Umstadter1999; Sylla et al. Reference Sylla, Flacco, Kahaly, Veltcheva, Lifschitz, Malka, d'Humières, Andriyash and Tikhonchuk2013; Puyuelo-Valdes et al. Reference Puyuelo-Valdes, Henares, Hannachi, Ceccotti, Domange, Ehret, d'Humieres, Lancia, Marquès, Santos and Tarisien2019b; Ospina-Bohórquez Reference Ospina-Bohórquez2022) in He gas-jet targets and gives a decreasing exponential energy spectrum as we have measured. At 0$^\circ$![]() , this signal is superimposed by some peaks (indicated by arrows in figure 3a) that could be the mark of an alternative acceleration process such as CSA or HB. Peaked structures have also been observed by Wei et al. (Reference Wei, Mangles, Najmudin, Walton, Gopal, Tatarakis, Dangor, Clark, Evans, Fritzler, Clarke, Hernandez-Gomez, Neely, Mori, Tzoufras and Krushelnick2004) where the detector spectrometer was placed at 100$^\circ$
, this signal is superimposed by some peaks (indicated by arrows in figure 3a) that could be the mark of an alternative acceleration process such as CSA or HB. Peaked structures have also been observed by Wei et al. (Reference Wei, Mangles, Najmudin, Walton, Gopal, Tatarakis, Dangor, Clark, Evans, Fritzler, Clarke, Hernandez-Gomez, Neely, Mori, Tzoufras and Krushelnick2004) where the detector spectrometer was placed at 100$^\circ$![]() . They considered this signal characteristic of laser-driven CSA. The two-component spectrum at 90$^\circ$
. They considered this signal characteristic of laser-driven CSA. The two-component spectrum at 90$^\circ$![]() in figure 3(c) could also be the mark of alternative acceleration schemes that compete with each other. This energy spectrum could be related to the bunched spectra measured at 60$^\circ$
in figure 3(c) could also be the mark of alternative acceleration schemes that compete with each other. This energy spectrum could be related to the bunched spectra measured at 60$^\circ$![]() in figure 3(d) if the low-energy part is removed. If this is the case, the formation of a collisionless shockwave could accelerate the protons to twice the shock velocity (Silva et al. Reference Silva, Marti, Davies, Fonseca, Ren, Tsung and Mori2004).
in figure 3(d) if the low-energy part is removed. If this is the case, the formation of a collisionless shockwave could accelerate the protons to twice the shock velocity (Silva et al. Reference Silva, Marti, Davies, Fonseca, Ren, Tsung and Mori2004).
Note that background noise in the 0$^\circ$![]() detector was caused by electric arcs between the high-voltage plates of the TP. These discharges come from electric breakdown due to the increase of pressure in the experimental chamber during the interaction. Other angles showed less background noise due to a better isolation of the TPs. The choice of BAS-MS and BAS-SR IPs was found to be a problem for measuring He ions since their sensitivity for low energies is approximately 3 MeV (due to their protection layers). For future experiments, BAS-TR IP will be used as they have a minimum sensitivity of approximately 0.8 MeV for He ions (since the protection layer is not present). A second option will be to isolate the TP equipped by a micro channel plate (MCP) detector in an independent chamber with differential vacuum. The MCP detector has the advantage of increased sensitivity and possibility of HRR operation.
detector was caused by electric arcs between the high-voltage plates of the TP. These discharges come from electric breakdown due to the increase of pressure in the experimental chamber during the interaction. Other angles showed less background noise due to a better isolation of the TPs. The choice of BAS-MS and BAS-SR IPs was found to be a problem for measuring He ions since their sensitivity for low energies is approximately 3 MeV (due to their protection layers). For future experiments, BAS-TR IP will be used as they have a minimum sensitivity of approximately 0.8 MeV for He ions (since the protection layer is not present). A second option will be to isolate the TP equipped by a micro channel plate (MCP) detector in an independent chamber with differential vacuum. The MCP detector has the advantage of increased sensitivity and possibility of HRR operation.
5. High repetition rate operation
Nozzle survival is a vitally important issue for HRR experiments. The strong interaction of the high-power laser with matter provokes a plasma that expands and interacts with the nozzle duct. This irreversibly damages the nozzle by the modification of its internal geometry. It is important to note that stainless steel, copper or polymers only stand one shot under these extreme conditions. Tungsten and UV fused silica nozzles were explored as nozzle materials to resist the plasma expansion damage. Figure 6 shows the density profiles of two tungsten nozzles after several laser interactions compared with the theoretical density profile given by the simulations in undamaged conditions. It is possible to see that after 25 shots, the density profile at the interaction distance is still reasonable and close to the predicted one, so tungsten is a suitable material to use in these types of experiment. Figure 7 shows scanning electron microscopy (SEM) pictures of the external exit diameter of the nozzles comparing an undamaged nozzle (figure 7a) with nozzles used during this campaign. The nozzle shown in figure 7(b) has been used for 21 laser shots and still could be used even if the density profile is slightly deteriorated. The nozzle shown in figure 7(c) has been used in 41 laser shots and was clearly damaged. In the latter, the straight duct is completely deformed, and the gas shock is faint and its position ambiguous.

Figure 6. Density profile comparison between measurements performed by interferometry and simulations at two distances from the nozzle exit using two shock nozzles: (a) $Z = 1510\ \mathrm {\mu }$![]() m, after 19 shots and (b) $Z = 1230\ \mathrm {\mu }$
m, after 19 shots and (b) $Z = 1230\ \mathrm {\mu }$![]() m, after 25 shots. The central high-density region is complicated to reconstruct due to the error using the Abel inversion at the symmetry axis. Anyway, we can clearly see that the high-density shock still resists in spite of the damage and the agreement with a theoretical undamaged nozzle is good.
m, after 25 shots. The central high-density region is complicated to reconstruct due to the error using the Abel inversion at the symmetry axis. Anyway, we can clearly see that the high-density shock still resists in spite of the damage and the agreement with a theoretical undamaged nozzle is good.

Figure 7. Scanning electron microscopy (SEM) pictures of the exit of the nozzles: (a) tungsten nozzle not used; (b) tungsten nozzle used in 21 laser shots; (c) tungsten nozzle used in 41 laser shots. The straight duct is clearly damaged. (d) Side picture of a UVFS fused silica nozzle after one shot at $Z = 400\ \mathrm {\mu }$![]() m. The original profile is marked by a red dashed line.
m. The original profile is marked by a red dashed line.
However, UVFS fused silica has been also tested as nozzle material. The fused silica nozzle resisted the 1000 bar backing pressure, but unfortunately the nozzle was shot at $400\ \mathrm {\mu }$![]() m due to an error and the nozzle was destroyed (figure 7d shows a slice of material removed after the laser shot). It was concluded that the nozzle could not resist the plasma shockwave and subsequent heating. Anyway, this material is expected to resist if used at higher Z distances and it will be tested in future campaigns. We consider that the extrapolation is not possible comparing the resistance of the fused silica nozzle with the tungsten nozzle at 1 mm above the outlet, where tungsten nozzles have been operated.
m due to an error and the nozzle was destroyed (figure 7d shows a slice of material removed after the laser shot). It was concluded that the nozzle could not resist the plasma shockwave and subsequent heating. Anyway, this material is expected to resist if used at higher Z distances and it will be tested in future campaigns. We consider that the extrapolation is not possible comparing the resistance of the fused silica nozzle with the tungsten nozzle at 1 mm above the outlet, where tungsten nozzles have been operated.
A second problem found for high repetition rate operation is the vacuum degradation of the experimental chamber. This can cause problems in the diagnostics using high voltages (sparks) and the absorption of particles if running continuously. This caused problems in our main detectors (IPs) since it blurred the main signal increasing the background noise as previously mentioned. Mylar protection was not enough to stop the light from the sparks due to its transparent nature. In addition, the extraction of the residual gas limited the maximum repetition rate of the experiment to have a proper vacuum environment (below $10^{-5}$![]() mbar) using a 1000 bar gas target. In these conditions, it was possible to perform consecutive laser shots with a repetition rate of 2 min$^{-1}$
mbar) using a 1000 bar gas target. In these conditions, it was possible to perform consecutive laser shots with a repetition rate of 2 min$^{-1}$![]() , which represents a significant advance compared with present state-of-art of laser-plasma acceleration of protons in gases. There is room for improvement if the residual gas is quickly extracted (via a gas catcher or isolation of the interaction region). This result opens the possibility of automatization and control of the particle source at HRR (Feister et al. Reference Feister, Cassou, Dann, Döpp, Gauron, Gonsalves, Joglekar, Marshall, Neveu and Schlenvoigt2023).
, which represents a significant advance compared with present state-of-art of laser-plasma acceleration of protons in gases. There is room for improvement if the residual gas is quickly extracted (via a gas catcher or isolation of the interaction region). This result opens the possibility of automatization and control of the particle source at HRR (Feister et al. Reference Feister, Cassou, Dann, Döpp, Gauron, Gonsalves, Joglekar, Marshall, Neveu and Schlenvoigt2023).
6. Conclusion
In conclusion, maximum proton energies of 2.2 MeV and 1.75 MeV were obtained at 90$^\circ$![]() and 60$^\circ$
and 60$^\circ$![]() , respectively, and optimization has been successfully reached to obtain forward proton acceleration with maximum energies of 2 MeV. In addition, bunched proton distributions at 60$^\circ$
, respectively, and optimization has been successfully reached to obtain forward proton acceleration with maximum energies of 2 MeV. In addition, bunched proton distributions at 60$^\circ$![]() were also observed. In the case of He$^{2+}$
were also observed. In the case of He$^{2+}$![]() , maximum energies were obtained of approximately 5.8 MeV only in the transverse direction. Finally, electrons were measured up to 70 MeV in the forward direction. It was proven that the optimization of the interaction position (via both interferometry and 2$\omega$
, maximum energies were obtained of approximately 5.8 MeV only in the transverse direction. Finally, electrons were measured up to 70 MeV in the forward direction. It was proven that the optimization of the interaction position (via both interferometry and 2$\omega$![]() emission) was fundamental to enhance particle acceleration at HRR. In addition, it a technical milestone was established with 41 shots performed with one single nozzle until fully damaged with a maximum repetition rate of 2 min$^{-1}$
emission) was fundamental to enhance particle acceleration at HRR. In addition, it a technical milestone was established with 41 shots performed with one single nozzle until fully damaged with a maximum repetition rate of 2 min$^{-1}$![]() . It was shown that high-resistance materials and higher distances from the outlet could enhance the nozzle survival. A study involving several 100s of shots in optimized conditions should be needed to definitely demonstrate the HRR potentials of the system. In future experiments, we plan to decrease the laser pulse duration down to 30 fs, and to scan densities slightly above and below the critical density to identify the best parameters for optimization of the ion spectral profile. Particle-in-cell (PIC) simulations will be essential to validate and help to understand these results, though these simulations have a high computational cost due to the large interaction regions that are needed to completely include the gas expansion. We have identified a need of diagnostic development and improvement of the gas extraction method to increase the repetition rate of the source, but the potential usefulness of gas-jet targets for an HRR particle source have been demonstrated.
. It was shown that high-resistance materials and higher distances from the outlet could enhance the nozzle survival. A study involving several 100s of shots in optimized conditions should be needed to definitely demonstrate the HRR potentials of the system. In future experiments, we plan to decrease the laser pulse duration down to 30 fs, and to scan densities slightly above and below the critical density to identify the best parameters for optimization of the ion spectral profile. Particle-in-cell (PIC) simulations will be essential to validate and help to understand these results, though these simulations have a high computational cost due to the large interaction regions that are needed to completely include the gas expansion. We have identified a need of diagnostic development and improvement of the gas extraction method to increase the repetition rate of the source, but the potential usefulness of gas-jet targets for an HRR particle source have been demonstrated.
Acknowledgements
We would like to express our gratitude to the laser and engineering teams at CLPU without whose support this work would not have been possible.
Editor Victor Malka thanks the referees for their advice in evaluating this article.
Declaration of interest
The authors report no conflict of interest.
Funding
This work received funding from Grant PID2021-125389OA-I00 funded by MCIN/AEI/10.13039/501100011033/FEDER, UE and by “ERDF A way of making Europe”, by the “European Union”.