1.1. Mapping

Remapping of EGT from 1947 to 1978 was carried out using a variety of methods of differing reliability: 1947, 1956, 1959, 1960, 1962, and 1965 US Navy aerial oblique photographs were used to plot maps using a Wilson photoalidade.

In 1966 and 1967, US Navy vertical photographs cover only parts of EGT, whereas in 1970 and 1978 complete coverage by vertical photography was obtained on request. The January 1970 map was plotted stereophoto grammetrically, without ground control, and the original map was ˜9% too large, since the uncorrected aircraft altimeter reading was used. Controlled (January 1978) photos were not acceptable for stereo-plotting so the map was constructed by the radial line method. The assumption was made that between 1970 and 1978, approximate steady-state thickness conditions prevailed (section 1.6). A reduction transform was then applied to the surface elevations on the 1970 map; the same transform was applied in the horizontal plane. Less reliable maps were produced for 1947, 1956, 1959, 1960, 1965, and 1967. Outlines for 1972, 1974, and 1976 were obtained from LANDSAT imagery.

1.2. Ice-flow rates from marginal tooth displacements

Individual teeth were numbered (14 on each edge). Each tooth was identified on preceding maps, where possible, and its distance from the hinge-line origin measured. Despite massive melt-back and calving, it was found possible to place some reliability on the time-distance plots (Fig.2).

1.3. Direct measurement of ice displacement

From 26 December 1977 to 25 January 1978, the positions of 12 reference poles were determined by resection to seven control points (Fig.l[c]).Elevation differences were corrected for curvature and refraction as well as for tidal variation. Most poles were surveyed three times. Due to scatter in the data, it is not possible to resolve the transverse velocity gradient. The data (Fig.3) are therefore taken to apply along the centre line, and the smooth curve put through the points is used to derive the longitudinal strain-rate. Velocities derived from tooth displacements are plotted in Figure 3; a possible higher average velocity seems to occur on the southern margin, particularly towards the hinge. The reliability of the derived strain-rates is shown by plotting a measurement made using a wire strainmeter (Reference Goodman and HoldsworthGoodman and Holdsworth 1978).

1.4. Ice thickness and water depths

Airborne radar has been flown along the centre line of EGT in 1967, 1978, and 1979 by the combined NSF/SPRI/TUD operation. For ice thinner than about 140 m, interpretation of ice bottom returns is complicated by possible returns from the marginal indentations and surface crevasses. Figure 4 shows the adopted geometric centre-line ice thickness. Absolute and relative errors in thickness are √15 m and ±10 m, respectively. Thickness at the margins is roughly 0.75H, where H is the centre thickness. Water depths were measured at 15 edge sites by calibrated tag-line. About 250 to 500 m of the northern edge seems to be resting on the ocean bottom, but there is no evidence of further grounding.

Fig.4. Data for EGT: ice thickness along the centre line with computed temperatures, sea-water temperatures, surface and basal ice balances

1.5. Surface net mass balance, density, and temperature

The surface net balance was estimated from measurements made at an array of wooden posts installed in 1973 by New Zealand Ministry of Works personnel. From measurements made up to 1977-78 an approximate balance curve has been derived (Fig.4). Firn densities were measured on core samples taken to 10.5 m depth, and these varied from 0.45 to 0.65 Mg m⁻³. The 10 m temperature was -19.8±0.1^°C.

Basal ice temperatures derived from equivalent water-depth temperatures (Reference Jacobs, Huppert , Holdsworth and DrewryJacobs and others 1981) are given in Figure 4. From these data, a steady-state finite element simulation of EGT temperature has been performed, in order to determine the mean column temperature along the centre line of EGT. Mean temperatures range from -14 to -12^°C (see section 2).

1.6. Mass balance of EGT

The mass-balance equation (Reference BuddBudd 1966) is:

(1)

where is the surface net balance is the basal balance is the average glacier column density,ρ_i is the density of ice, is the vertical strain-rate, V_x is the horizontal velocity component, and H is the total ice thickness at distance x from the hinge line. For steady state ∂H/∂t = 0 and (1) may be solved for .

Values of thus derived are shown in Figure 4. Despite large errors in H and ∂H/∂t, there appears to be a trend of increasing melt rate toward the free end except at x=10 to 11 km where some freezing may occur. That melting is taking place is positively indicated by the results of Reference Jacobs, Huppert , Holdsworth and Drewry Jacobs and others (1981), which show steps in conductivity-temperature-depth (CTD) profiles taken to depths of 250 m close to EGT.

2.1. Derivation of flow law “viscosity” parameter B

The flow law is assumed to be of the form:

(2)

where

(2a)

is the effective strain-rate, and

(2b)

is the effective stress, is the component of strain-rate and is the component of the deviator stress in which (i,j)=(x,y,z) (x-longitudinal, y-transverse and z-vertical). B is dependent on ice temperature, structure, and impurity; n is usually, and will herein be taken as, 3.

It may be shown that vertical plane shear stresses are small compared to normal stresses. The maximum value of σ_zx is (Reference Sanderson and DoakeSanderson and Doake 1979):

where h = (l–ρ/ρ_w)H in which ρ_w is the density of seawater. Substituting h=32 m, H=208 m, dh/dx=0.0025, (x=4000 m),

Other stresses of the type σ_ij(i≠j) are assumed to be small, except possibly at the hinge line or in the region of re-entrants

Values of and τ were computed using Equations (2a) and (2b) (Reference ThomasThomas 1973). In (2a) the vertical strain-rate was determined from the (approximate) incompressibility relationship to be

where is taken as ˜0.9 rather than 1.0 (section 3).

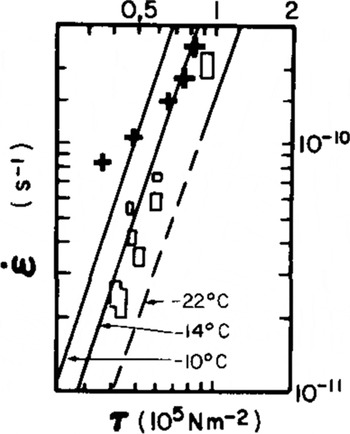
The plot of points is shown in Figure 5. Individual values of B may be computed from these data using Equation (2) and assuming n=3. The results are shown in the following tabulation:

Fig.5 Plot of effective strain-rate vs effective stress (τ) for points on centre line of EGT. Line slopes are for n = 3 for temperatures given inReference Thomas Thomas (1973[b]). (Open rectangles are data from other ice shelves.)

These data may be interpreted as follows: as the ice thins and the mean ice temperature increases, and as crevasse depths are a significant fraction of the total ice thickness, the ice tends to become "softer" This would evidently explain the apparent drift of points away from a straight line in Figure 5. Thus the strain-rates are higher than expected for a given effective stress.

There is now better agreement with the data of Reference ThomasThomas (1973). The “equilibrium” profile study of EGT (Reference SandersonSanderson 1979) should now be revised with B= B(x). The now greater effective viscosity of the ice near the hinge line would result in slower thinning rates there, and render, as required, a better model fit to the data in the hinge zone (Reference SandersonSanderson 1979: 441).

3.1. Geometry

From Figure 1 it may be seen that a transverse asymmetry develops with time; the thickest ice becomes closer to the south edge, as a result of progressive melting along the southern edge, which greatly exceeds melting along the northern edge. A dynamic centre line is shown on the 1970 map. Figure 6 shows a plot of the radius of curvature of this line. Despite the large scatter in data points, an increase in curvature with distance (x) is quite apparent. A possible initial curvature may be due to differential exit speeds on either side of the hinge line.

Fig.6 Curvature of dynamic centre line of EGT, measured from Figure 1. Points obtained by two operators from 1970 and 1978 maps.

3.2. Lateral half-widths of EGT and determination of melt rates

Figure 7 shows the half-widths for the northern and southern sides of the dynamic centre line : melting is clearly most severe on the southern side to the extent that lateral creep spreading is completely obliterated. On the northern side, lateral spreading is seen as far as x≈5 km, before melting-back dominates. In order to compute melt rates, it is necessary to compute the lateral spreading due to Weertman creep (Reference WeertmanWeertman 1957). Plots Of half-widths for different are shown in Figure 7, and a value of α= 0.9 is considered likely. A time scale (Fig.3) was established using

(4)

From this, the incremental spreading ∆y is computed for distance steps ∆x of 500 m (∆t ˜ 4a): The resulting theoretical half-wraths minus the measured half-widths give the meltback (Fig.7). These melt rates probably correspond with the average surface water temperature of -1.3^°C (Reference Jacobs, Huppert , Holdsworth and DrewryJacobs and others 1981) and are shown in Figure 8 together with basal melt rates which fall below the line of Reference Morgan, Budd and HusseinyMorgan and Budd (1978), possibly because of lower water speeds on the bottom surface. Differential melt rates on either side of EGT thus increase its apparent curvature over the curvature due to creep bending.

Fig.7 Plot of half-widths of EGT measured from the dynamic centreline. Spreading rates for the transverse strain factor α = 0.5, 0.9, and 1.0 are shown. Derived melt rates for the north and south edges are given

Fig.8 Ice melt rates versus water temperature

3.3. Curvature induced by dynamic processes

The curvature of the “dynamic” centre line may be caused by differential exit speeds on either side of the hinge line or by ocean current pressure exerted from the south

3.3.1. Differential exit speeds

Figure 3 shows that if there is a difference in flow rates on either side of the hinge line, it is not greater than about the error of the measurements (±5 m a⁻¹). If V_n and V_s are the exit speeds on the north and south edges, separated by a full glacier width of W₀, then the curvature generated at the hinge line would be

and for V_s -V_n = 1 m a⁻¹, (V_n +V_s)/2 = 85 m a⁻¹, and W₀ ˜ 2 000 m,

An initial curvature of this magnitude may be possible (see Fig.6).

3.3.2. Current pressure acting on the south edge of EGT

Because melt rates on the southern edge have been shown to be about three times those on the northern edge (Fig.7) and because curvature increases with distance from the hinge line (Fig.6), the effects of ocean current pressure on EGT must be examined. As no direct current measurements are available in the vicinity of EGT, we use the following information: (i) visual evidence for a northward current sweeping around EGT can be seen in figure 1 of Reference HoldsworthHoldsworth (1974) (see also Reference Holdsworth and GlynnHoldsworth and Glynn 1981), (ii) holes drilled through the sea ice on the south side produced turbulent up wellings (holes on the north side apparently did not), and (iii) hydrographic and sailing manuals for Antarctica indicate the existence of a strong (3 knots (1.5 m s⁻¹)) current setting north around the coast in the vicinity of EGT

A water current of uniform speed is assumed to flow into EGT from the south and to lose momentum to the glacier. A mean current flow constant with depth and distance from the coast is taken as 1 knot (0.5 m s⁻¹). We wish to determine the bending moment and, subsequently, the bending stresses induced in EGT. For these purposes, the form of EGT is somewhat idealized to facilitate computation. If the force acting on an elemental area of EGT of length dx and height is 0.5 C_D ρ H V_c ² dx, where C_D is the form drag coefficient (˜1.95) and V_c is the current speed (H is taken as in ((1)),then the moment M_x ^'of this force about a vertical axis at x^' from the origin is

(5)

(see Reference Holdsworth and GlynnHoldsworth and Glynn (1981)). The resulting moment distribution is shown in Figure 9. The maximum (edge) bending stress at x^' is

(6)

where W is the actual width as measured from Figure 1, and n = 3 (section 2). H _e is the “effective thickness” of EGT (0.75H< H_e < H). (The effective thickness is H minus crevasse depth, ~30 m.) Figure 9 shows values of (max). The highest stresses can occur beyond the hinge line, depending on the variation of W(x) and, to a lesser extent, on the local variations of H_e. Thus, some significant stress maxima may occur far from the hinge line. The bending stress and creep strain history of selected points along the edge of EGT will now be examined to see if curvatures comparable to the observed curvatures (Fig.6) may be generated. It is necessary, first, to present the history of the length variations of EGT (Fig.10). Figure 11 presents the time variation of bending stress for three selected points on the edge of EGT using the information in Figure 10, Equations (5) and (6) and a deduced value of dW/dt.

Fig.9 Plot of bending moment and bending stress EGT (1978

Fig.10 Length-time record for EGT (1902 to present)

Next, the strain-rates corresponding to these stresses will be computed. The assumption is made that bending and linear creep stresses may be directly superimposed. At a particular point on the edge of a smooth margined EGT model, the stress (deviator) components become

where is the bending stress in the edge parellel to the x-axis and is the Weertman creep stress in the same direction. The transverse component of the creep stress is assumed to be 0.9 A flow law of the same form as Equation (2) is assumed, so that in component form

(7)

where B and n are the values obtained previously

The creep rates are computed separately using Equation (7) to obtain

The stresses shown in Figure 11 were used to compute, in stages, the value of for each stress level, and the total creep bending increment ∆lover length l was then computed from where ∆t and ∆x,are the time and distance increments, respectively

Fig.11 Bending stress history for selected points (Fig.1(b)) on north and south edges of EGT, with and without Weertman creep

The mean curvature R ^–1 over l is obtained from the expressions

(8)

For point A (Fig1(b)) travelling a total distance l˜9 500 m, the mean curvatur , which is greater than the mean observed curvature (Fig.6) by a factor of 6. This discrepancy may be explained by any or all of the following: (i) the value of B used in Equation 7 is too low, (ii) the water current speed (and hence pressure) assumed to be incident on EGT is too high, and (iii) the presence of fast ice around EGT retards lateral deformation.

Possible errors in B and V_c are unlikely to account for the above discrepancy, except possibly if cases (i) and (ii) occur together. More likely, however, is case (iii): consider the compressive stress induced in the sea ice as a result of the pressure exerted on EGT. A normal stress of magnitude

would be induced in the sea ice of thickness h_j. For typical values of H = 200 m, and H_j = 3.5 m, this stress is ~0.013 MN m⁻² which is probably sufficient to cause only very slow creep in the sea ice. If we next assume that lateral deformation is essentially only allowed over 1/6 of the total time (or an average of two months per year), then the curvature, recomputed through Equation 8, becomes about 40 × 10⁻⁶ m⁻¹. This is now approximately equal to the observed curvature. The complete history of breakout of fast ice around EGT is not known. It is, however, known that breakout does not occur every year, although the ice probably becomes loose and weak around the glacier. Such a condition might allow significant deformation of EGT during the summer

Another possibility, not considered above because of lack of information, is that a northward current does not prevail throughout the entire year.

5.1. Vibration calving mechanism

Calving of EGT was dfscussed in Reference Holdsworth and GlynnHoldsworth and Glynn (1981) with particular emphasis on the vibration mechanism acting as a trigger. Evidently, open water conditions are necessary for this mechanism to be sufficiently activated. Local bending stress maxima may occur far from the hinge line (Reference Holdsworth and GlynnHoldsworth and Glynn 1981 and Fig.9). A particularly vulnerable section is indicated by B in Figure 1(b). This is approximately the line of the 1911 calving (and presumably, also, the 1942 calving). The localized vertical thinning between 8 and 9 km (Fig.4) is associated with a pronounced surface valley and is a potential location for calving in the future.

5.2. Calving at the hinge line by horizontal bending

It is of interest to examine the history of the bending stress at the hinge line, particularly since the three most easterly teeth on the south edge have recently disintegrated (Fig.1(b)). The 1978 width of EGT at the section G-H was 860 m, whereas in 1970 the narrowest section was 1300 m; and all previous maps show, in general, at least a 1000 m width, given the uncertainties in mapping accuracy.

The south edge hinge-line tensile stress has been computed using Equations (5) and (6) and results are shown in Figure 12, where the time scale is keyed to that in Figure 10. It may be seen that the rate of increase of tensile stress due to bending is decreasing with time because the thinning, advancing ice exerts a progressively smaller bending moment at the hinge. If basal melting predominates, the hinge-line width remains constant at 1200 m and no calving occurs (an unlikely situation); then the maximum length of EGT, of about 16 km, would be reached shortly after 2000 AD.

From recent field observations (R Holdsworth private communication), it appears that the re-entrant rift at the hinge line is becoming deeper, hence by Equation (6), since W is decreasing, must be increasing (beyond the values given in Figure 12 which has been computed using a typical average glacier width W = 1200 m). A potential feedback stress-width regime now exists, since and the stress (or the accompanying strain-rate) will tend to drive a re-entrant or crack deeper into the glacier, reducing W.

Fig.12 Idealized south edge hinge tensile stress vs time for constant width w = 1200 m) and thickness H. Maximum and minimum stresses derived using an effective thickness of 0.75H and H (H = 340m). Steep lines beginning 1976-78 indicate actual recent stress development.